7 Essential Properties of Parallelograms to Master
Unlocking the Secrets of Parallelograms
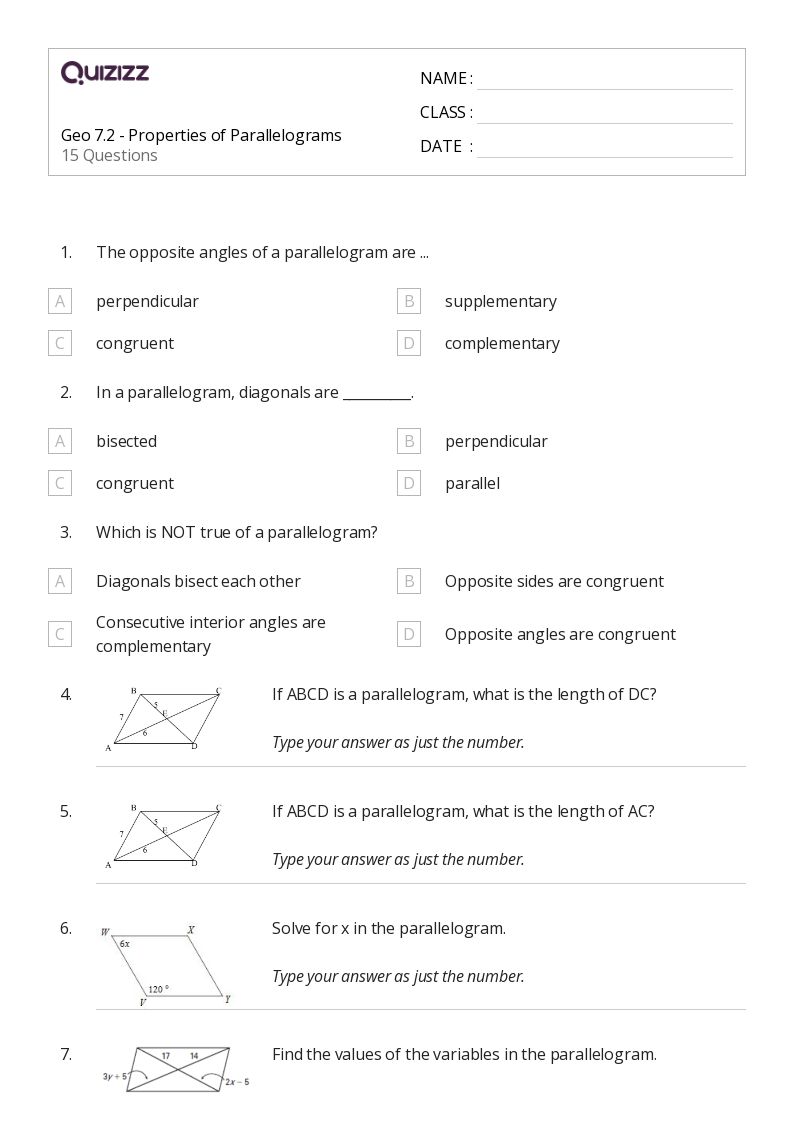
Parallelograms are a fundamental concept in geometry, and understanding their properties is crucial for solving problems and mastering more advanced mathematical concepts. In this article, we will delve into the 7 essential properties of parallelograms that you need to know to become a geometry master.
Property 1: Definition and Classification
A parallelogram is a quadrilateral with opposite sides that are parallel to each other. It can be classified into different types, including:
- Rectangle: A parallelogram with right angles (90 degrees)
- Rhombus: A parallelogram with all sides of equal length
- Square: A parallelogram with all sides of equal length and right angles
- Trapezoid: A parallelogram with only one pair of parallel sides
📝 Note: A parallelogram can be a rectangle, but a rectangle is not always a parallelogram.
Property 2: Opposite Sides are Equal
One of the most important properties of parallelograms is that opposite sides are equal in length. This means that if you have a parallelogram with sides of length a, b, c, and d, then a = c and b = d.
| Side | Length | |
|---|---|---|
| a | || | c |
| b | || | d |
Property 3: Opposite Angles are Equal
Another essential property of parallelograms is that opposite angles are equal. This means that if you have a parallelogram with angles A, B, C, and D, then A = C and B = D.
📝 Note: This property can be used to find missing angles in a parallelogram.
Property 4: Consecutive Angles are Supplementary
Consecutive angles in a parallelogram are supplementary, meaning they add up to 180 degrees. This property can be useful in solving problems involving angles.
- A + B = 180 degrees
- C + D = 180 degrees
Property 5: Diagonals Bisect Each Other
The diagonals of a parallelogram bisect each other, meaning they intersect at their midpoints. This property can be used to find the midpoint of a diagonal.
📝 Note: The diagonals of a parallelogram do not necessarily intersect at right angles.
Property 6: Area and Perimeter
The area of a parallelogram can be found using the formula:
Area = base × height
The perimeter of a parallelogram can be found by adding the lengths of all its sides.
- Perimeter = a + b + c + d
Property 7: Symmetry
Parallelograms have line symmetry, meaning they can be divided into two identical halves by a line of symmetry. This property can be useful in solving problems involving symmetry.
As we can see, mastering the properties of parallelograms is essential for becoming proficient in geometry. By understanding these 7 essential properties, you will be able to solve problems and unlock the secrets of more advanced mathematical concepts.
And that’s the key to becoming a geometry master!
What is the definition of a parallelogram?
+A parallelogram is a quadrilateral with opposite sides that are parallel to each other.
What are the different types of parallelograms?
+There are four main types of parallelograms: rectangle, rhombus, square, and trapezoid.
What is the formula for the area of a parallelogram?
+The formula for the area of a parallelogram is Area = base × height.