Master Product of Powers with 5 Essential Rules
Mastering the Product of Powers with 5 Essential Rules
The product of powers is a fundamental concept in algebra, and mastering it is crucial for solving complex mathematical problems. The product of powers refers to the multiplication of two or more exponential expressions with the same base. In this article, we will explore the five essential rules for working with the product of powers, along with examples and illustrations to help you understand the concepts better.
Rule 1: Multiplying Exponents with the Same Base
When multiplying two exponential expressions with the same base, you can simply add the exponents. This rule can be expressed mathematically as:
am × an = am+n
For example:
23 × 24 = 23+4 = 27
📝 Note: When adding exponents, make sure the bases are the same. If the bases are different, you cannot add the exponents.
Rule 2: Multiplying Exponents with Different Bases
When multiplying two exponential expressions with different bases, you cannot simply add the exponents. Instead, you need to multiply the bases and keep the exponents separate.
am × bn = am × bn
For example:
23 × 34 = 23 × 34
📝 Note: When multiplying exponents with different bases, make sure to keep the exponents separate and do not attempt to add them.
Rule 3: Raising a Power to a Power
When raising a power to a power, you need to multiply the exponents. This rule can be expressed mathematically as:
(am)n = am×n
For example:
(23)4 = 23×4 = 212
📝 Note: When raising a power to a power, make sure to multiply the exponents correctly.
Rule 4: Negative Exponents
When working with negative exponents, you need to follow the rule:
a-m = 1/am
For example:
2-3 = 1⁄23
📝 Note: When working with negative exponents, make sure to flip the fraction and change the sign of the exponent.
Rule 5: Zero Exponents
When working with zero exponents, you need to follow the rule:
a0 = 1
For example:
20 = 1
📝 Note: When working with zero exponents, the result is always 1, regardless of the base.
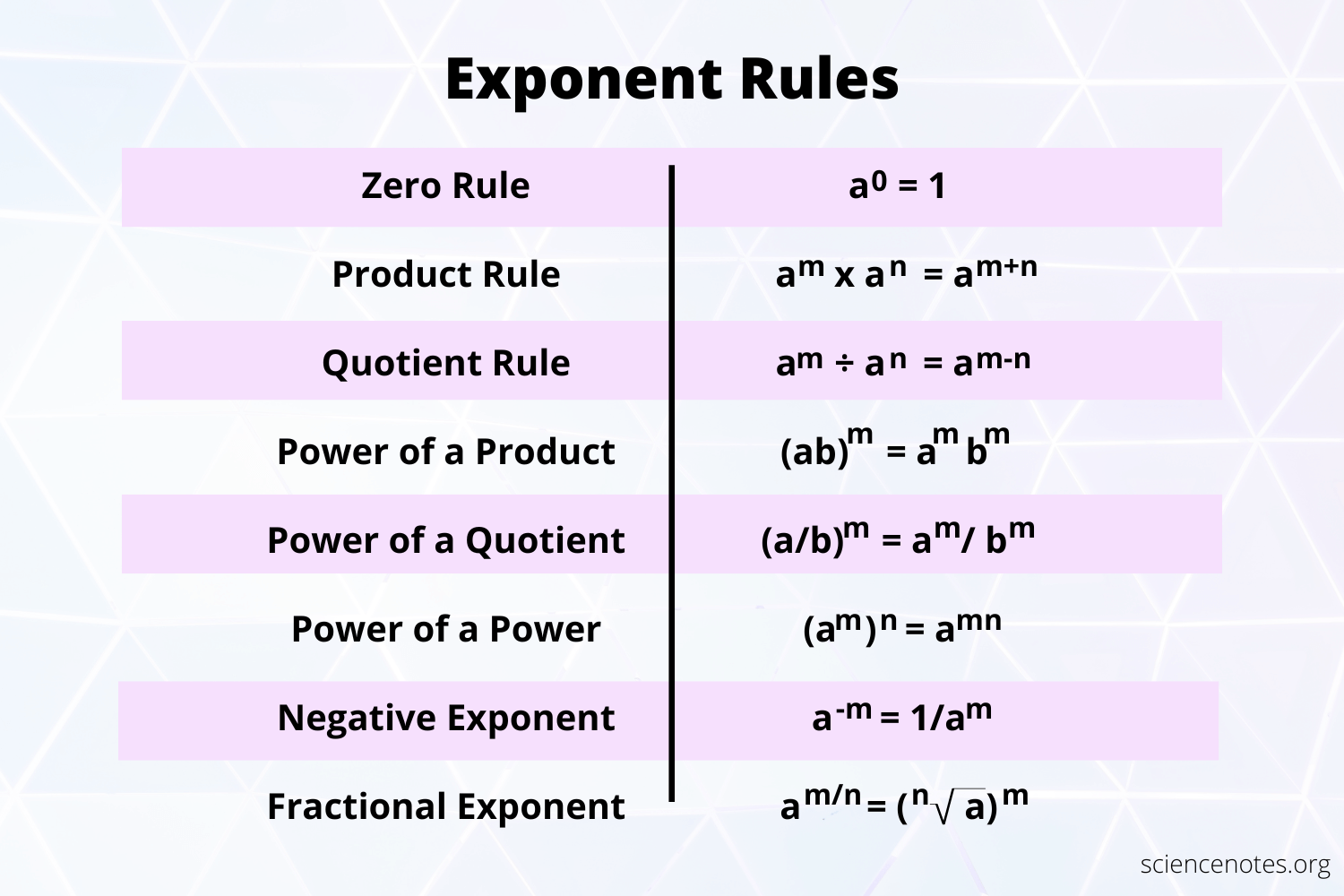
| Rule | Mathematical Expression | Example |
|---|---|---|
| Rule 1: Multiplying Exponents with the Same Base | am × an = am+n | 23 × 24 = 23+4 = 27 |
| Rule 2: Multiplying Exponents with Different Bases | am × bn = am × bn | 23 × 34 = 23 × 34 |
| Rule 3: Raising a Power to a Power | (am)n = am×n | (23)4 = 23×4 = 212 |
| Rule 4: Negative Exponents | a-m = 1/am | 2-3 = 1/23 |
| Rule 5: Zero Exponents | a0 = 1 | 20 = 1 |
In conclusion, mastering the product of powers requires understanding and applying the five essential rules outlined above. By following these rules, you can simplify complex exponential expressions and solve mathematical problems with ease.
What is the product of powers in algebra?
+The product of powers refers to the multiplication of two or more exponential expressions with the same base.
How do I multiply exponents with the same base?
+When multiplying exponents with the same base, you can simply add the exponents.
What is the rule for raising a power to a power?
+When raising a power to a power, you need to multiply the exponents.
How do I handle negative exponents?
+When working with negative exponents, you need to flip the fraction and change the sign of the exponent.
What is the result of zero exponents?
+When working with zero exponents, the result is always 1, regardless of the base.