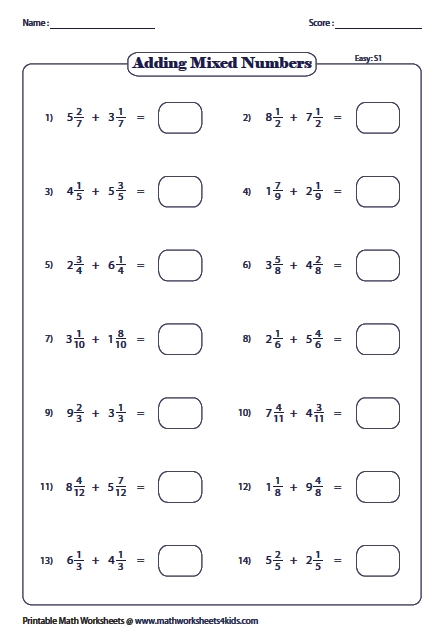
Add Mixed Fractions with Ease Worksheets
Mixed Fractions with Ease: A Comprehensive Guide
Understanding mixed fractions can be a daunting task for many students. However, with the right approach and practice, it can become second nature. In this article, we will explore the concept of mixed fractions, their importance, and provide a range of worksheets to help you master them.
What are Mixed Fractions?
A mixed fraction is a combination of a whole number and a proper fraction. It represents a quantity that is greater than one whole unit. For example, 2 3⁄4 is a mixed fraction, where 2 is the whole number and 3⁄4 is the proper fraction.
Why are Mixed Fractions Important?
Mixed fractions are used extensively in real-life applications, such as cooking, measurement, and finance. They help us to express quantities that are greater than one whole unit in a more concise and meaningful way.
How to Add Mixed Fractions
Adding mixed fractions can be a bit tricky, but with the right steps, it can be done easily. Here are the steps to follow:
- Step 1: Convert the mixed fractions to improper fractions.
- Step 2: Find the least common denominator (LCD) of the two fractions.
- Step 3: Add the fractions using the LCD.
- Step 4: Convert the result back to a mixed fraction.
For example, let’s add 2 3⁄4 and 1 1⁄2:
- Step 1: Convert the mixed fractions to improper fractions: 11⁄4 and 3⁄2.
- Step 2: Find the LCD: 4.
- Step 3: Add the fractions: 11⁄4 + 6⁄4 = 17⁄4.
- Step 4: Convert the result back to a mixed fraction: 4 1⁄4.
Worksheets for Adding Mixed Fractions
Here are some worksheets to help you practice adding mixed fractions:
Worksheet 1:
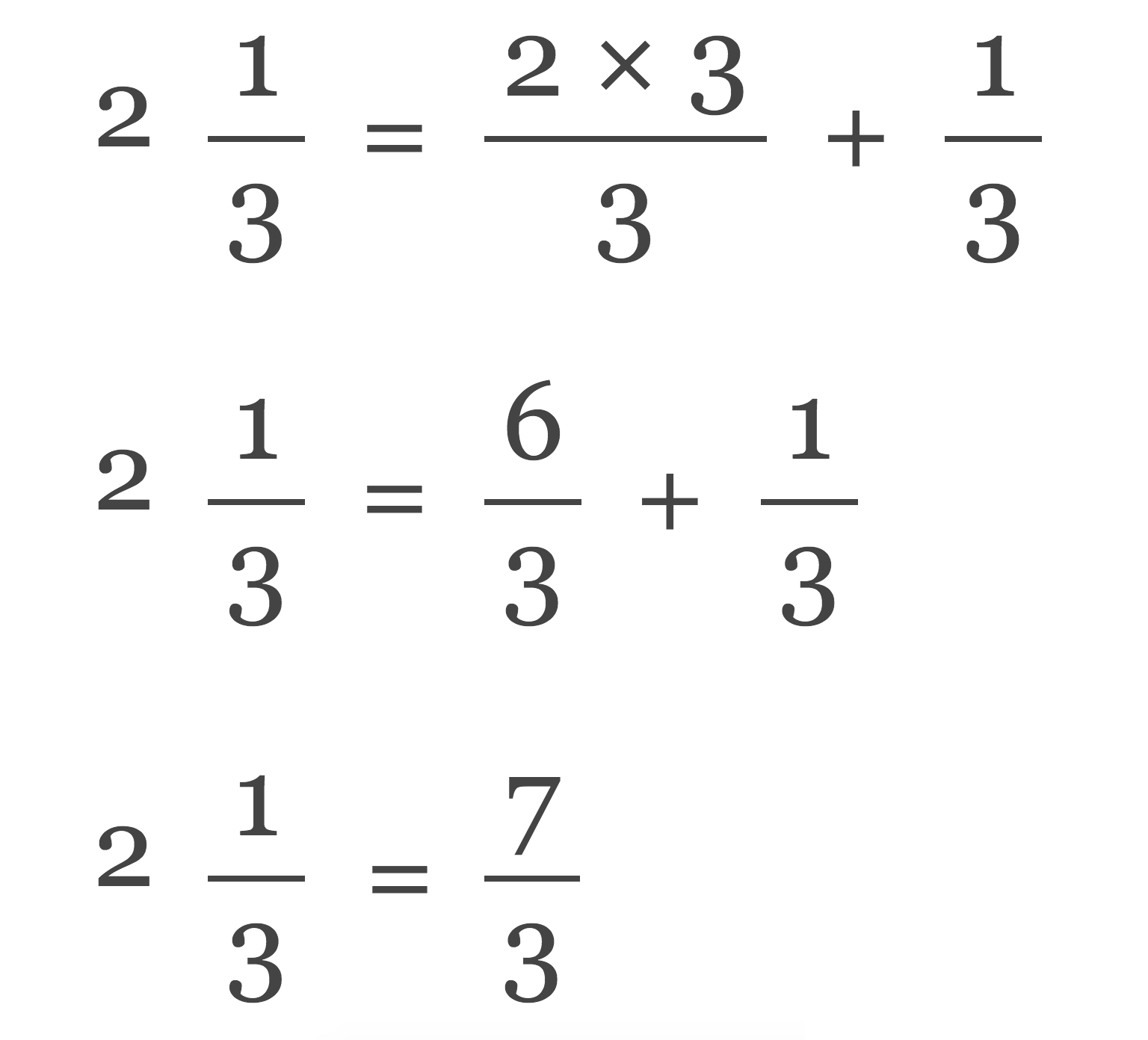
| Mixed Fractions | Result |
|---|---|
| 1 1⁄2 + 2 3⁄4 | |
| 2 3⁄4 + 1 1⁄2 | |
| 3 1⁄2 + 2 1⁄4 |
Worksheet 2:
| Mixed Fractions | Result |
|---|---|
| 2 1⁄4 + 1 3⁄4 | |
| 3 3⁄4 + 2 1⁄2 | |
| 1 1⁄2 + 3 3⁄4 |
Worksheet 3:
| Mixed Fractions | Result |
|---|---|
| 2 2⁄3 + 1 1⁄3 | |
| 3 1⁄2 + 2 2⁄3 | |
| 1 3⁄4 + 3 1⁄2 |
How to Subtract Mixed Fractions
Subtracting mixed fractions is similar to adding them. Here are the steps to follow:
- Step 1: Convert the mixed fractions to improper fractions.
- Step 2: Find the least common denominator (LCD) of the two fractions.
- Step 3: Subtract the fractions using the LCD.
- Step 4: Convert the result back to a mixed fraction.
For example, let’s subtract 2 3⁄4 from 4 1⁄2:
- Step 1: Convert the mixed fractions to improper fractions: 11⁄4 and 9⁄2.
- Step 2: Find the LCD: 4.
- Step 3: Subtract the fractions: 18⁄4 - 11⁄4 = 7⁄4.
- Step 4: Convert the result back to a mixed fraction: 1 3⁄4.
Worksheets for Subtracting Mixed Fractions
Here are some worksheets to help you practice subtracting mixed fractions:
Worksheet 1:
| Mixed Fractions | Result |
|---|---|
| 4 1⁄2 - 2 3⁄4 | |
| 3 1⁄2 - 1 1⁄4 | |
| 2 3⁄4 - 1 1⁄2 |
Worksheet 2:
| Mixed Fractions | Result |
|---|---|
| 2 1⁄4 - 1 3⁄4 | |
| 3 3⁄4 - 2 1⁄2 | |
| 1 1⁄2 - 3 3⁄4 |
Worksheet 3:
| Mixed Fractions | Result |
|---|---|
| 2 2⁄3 - 1 1⁄3 | |
| 3 1⁄2 - 2 2⁄3 | |
| 1 3⁄4 - 3 1⁄2 |
Mixed Fractions Word Problems
Here are some word problems to help you practice applying mixed fractions in real-life situations:
- Problem 1: Tom has 2 3⁄4 cups of flour in a bowl. He adds 1 1⁄2 cups of flour to it. How many cups of flour does Tom have now?
- Problem 2: A bookshelf has 3 1⁄2 shelves. If 2 1⁄4 shelves are removed, how many shelves are left?
- Problem 3: A recipe requires 2 3⁄4 cups of sugar. If you have 1 1⁄2 cups of sugar, how much more sugar do you need?
Conclusion
Mixed fractions are an essential concept in mathematics, and mastering them can help you in a wide range of real-life applications. By practicing the worksheets and word problems provided in this article, you can become proficient in adding and subtracting mixed fractions with ease.
What is a mixed fraction?
+A mixed fraction is a combination of a whole number and a proper fraction.
How do I add mixed fractions?
+To add mixed fractions, convert them to improper fractions, find the least common denominator, add the fractions, and convert the result back to a mixed fraction.
What are some real-life applications of mixed fractions?
+Mixed fractions are used in cooking, measurement, and finance, among other areas.