5 Ways to Master Parallel Perpendicular or Neither
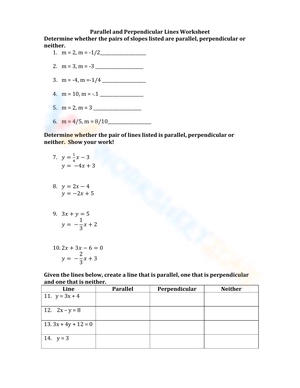
Mastering Parallel, Perpendicular, or Neither: A Comprehensive Guide
Geometry is a fascinating subject that deals with the study of shapes, sizes, and positions of objects. One of the fundamental concepts in geometry is understanding the relationships between lines, specifically whether they are parallel, perpendicular, or neither. In this article, we will explore five ways to master parallel, perpendicular, or neither, making it easier for you to tackle geometry problems with confidence.
Understanding the Basics
Before we dive into the five ways to master parallel, perpendicular, or neither, let’s quickly review the definitions:
- Parallel lines: Lines that lie in the same plane and never intersect, no matter how far they are extended. Symbol: ||
- Perpendicular lines: Lines that intersect at a 90-degree angle (right angle). Symbol: ⊥
- Neither: Lines that are not parallel or perpendicular, meaning they intersect at an angle other than 90 degrees.
1. Visualizing with Diagrams
One of the most effective ways to understand parallel, perpendicular, or neither is by visualizing diagrams. Draw lines on a piece of paper and label them as parallel, perpendicular, or neither. This hands-on approach helps you internalize the concepts and recognize the differences between the three.
Example:
Draw two lines, AB and CD, on a piece of paper. Label them as parallel (||). Now, draw a third line, EF, that intersects AB at a 90-degree angle. Label EF as perpendicular to AB (⊥). Finally, draw a fourth line, GH, that intersects AB at an angle other than 90 degrees. Label GH as neither parallel nor perpendicular to AB.
2. Using Slope-Intercept Form
Another way to determine whether lines are parallel, perpendicular, or neither is by using the slope-intercept form of a line (y = mx + b). If two lines have the same slope (m), they are parallel. If the slopes are negative reciprocals of each other, the lines are perpendicular.
Example:
Find the slope of the lines 2x + 3y = 5 and x - 2y = -3.
- Line 1: 2x + 3y = 5 → y = (-2⁄3)x + 5⁄3 (slope = -2⁄3)
- Line 2: x - 2y = -3 → y = (1⁄2)x + 3⁄2 (slope = 1⁄2)
Since the slopes are not the same, the lines are not parallel. However, the slopes are not negative reciprocals of each other either (-2⁄3 ≠ -1/(1⁄2)), so the lines are neither parallel nor perpendicular.
3. Applying the Parallel and Perpendicular Postulates
The parallel and perpendicular postulates are useful theorems that can help you determine the relationships between lines.
- Parallel Postulate: If two lines are cut by a transversal and the interior angles on the same side of the transversal are supplementary (add up to 180 degrees), then the lines are parallel.
- Perpendicular Postulate: If two lines are cut by a transversal and the interior angles on the same side of the transversal are right angles (90 degrees), then the lines are perpendicular.
Example:
Given lines AB and CD, with transversal EF intersecting them, find the relationship between AB and CD.
- ∠AEF + ∠CEF = 180° (supplementary angles)
- ∠AEF = 90° and ∠CEF = 90° (right angles)
Since the angles are supplementary, AB || CD. However, if the angles were right angles, AB ⊥ CD.
4. Utilizing Coordinate Geometry
Coordinate geometry provides a powerful tool for determining the relationships between lines. By finding the coordinates of the points where two lines intersect, you can determine whether the lines are parallel, perpendicular, or neither.
Example:
Find the relationship between the lines passing through points (2,3) and (4,5), and points (1,2) and (3,4).
- Line 1: (2,3) and (4,5)
- Line 2: (1,2) and (3,4)
Calculate the slopes of the lines:
- Line 1: slope = (5-3)/(4-2) = 2⁄2 = 1
- Line 2: slope = (4-2)/(3-1) = 2⁄2 = 1
Since the slopes are the same, the lines are parallel.
5. Practicing with Real-World Applications
Finally, practicing with real-world applications helps reinforce your understanding of parallel, perpendicular, or neither. Look for examples in architecture, engineering, or design, where these concepts are crucial.
Example:
Design a rectangular garden with a length of 10 meters and a width of 5 meters. The garden has a path running diagonally from one corner to the other. What is the relationship between the path and the sides of the garden?
- Draw a diagram of the garden and label the sides and the path.
- Use the slope-intercept form to find the slope of the path and the sides.
- Determine the relationship between the path and the sides using the slope-intercept form.
Since the path intersects the sides at 90-degree angles, the path is perpendicular to the sides.
📝 Note: Practice is key to mastering parallel, perpendicular, or neither. Try to solve as many problems as you can to reinforce your understanding of these concepts.
In conclusion, mastering parallel, perpendicular, or neither requires a combination of visualizing diagrams, using slope-intercept form, applying postulates, utilizing coordinate geometry, and practicing with real-world applications. By following these five ways, you’ll become proficient in identifying the relationships between lines and be well-prepared to tackle more complex geometry problems.
What is the difference between parallel and perpendicular lines?
+Parallel lines are lines that lie in the same plane and never intersect, no matter how far they are extended. Perpendicular lines are lines that intersect at a 90-degree angle (right angle).
How can I determine whether two lines are parallel, perpendicular, or neither?
+You can use the slope-intercept form, apply the parallel and perpendicular postulates, utilize coordinate geometry, or practice with real-world applications to determine the relationship between two lines.
What is the importance of understanding parallel, perpendicular, or neither in real-life applications?
+Understanding parallel, perpendicular, or neither is crucial in various fields such as architecture, engineering, design, and more, where accurate calculations and spatial awareness are essential.



