5 Ways to Prove Parallel Lines
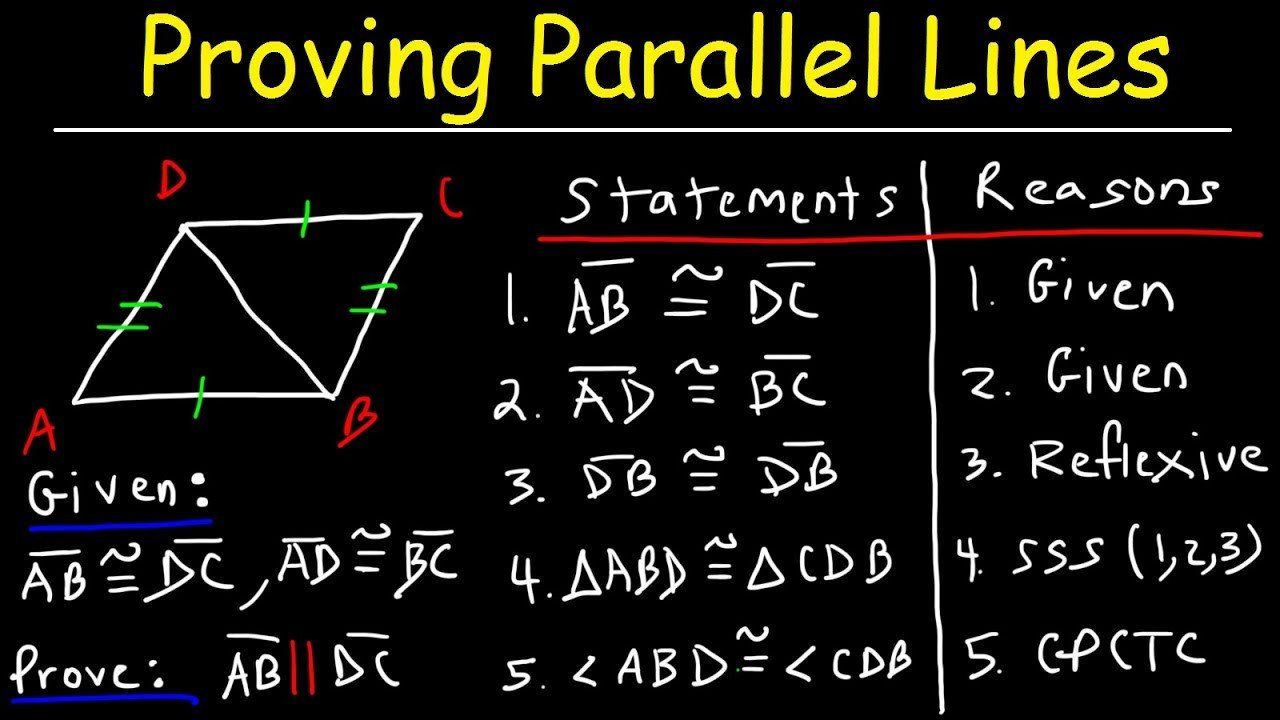
Understanding Parallel Lines
In geometry, parallel lines are lines that lie in the same plane and never intersect, no matter how far they are extended. These lines have the same slope and are always at the same distance apart. To prove that two lines are parallel, we need to establish their relationship using various methods. In this article, we will explore five ways to prove parallel lines, including using corresponding angles, alternate interior angles, alternate exterior angles, consecutive interior angles, and transversal lines.
Method 1: Corresponding Angles
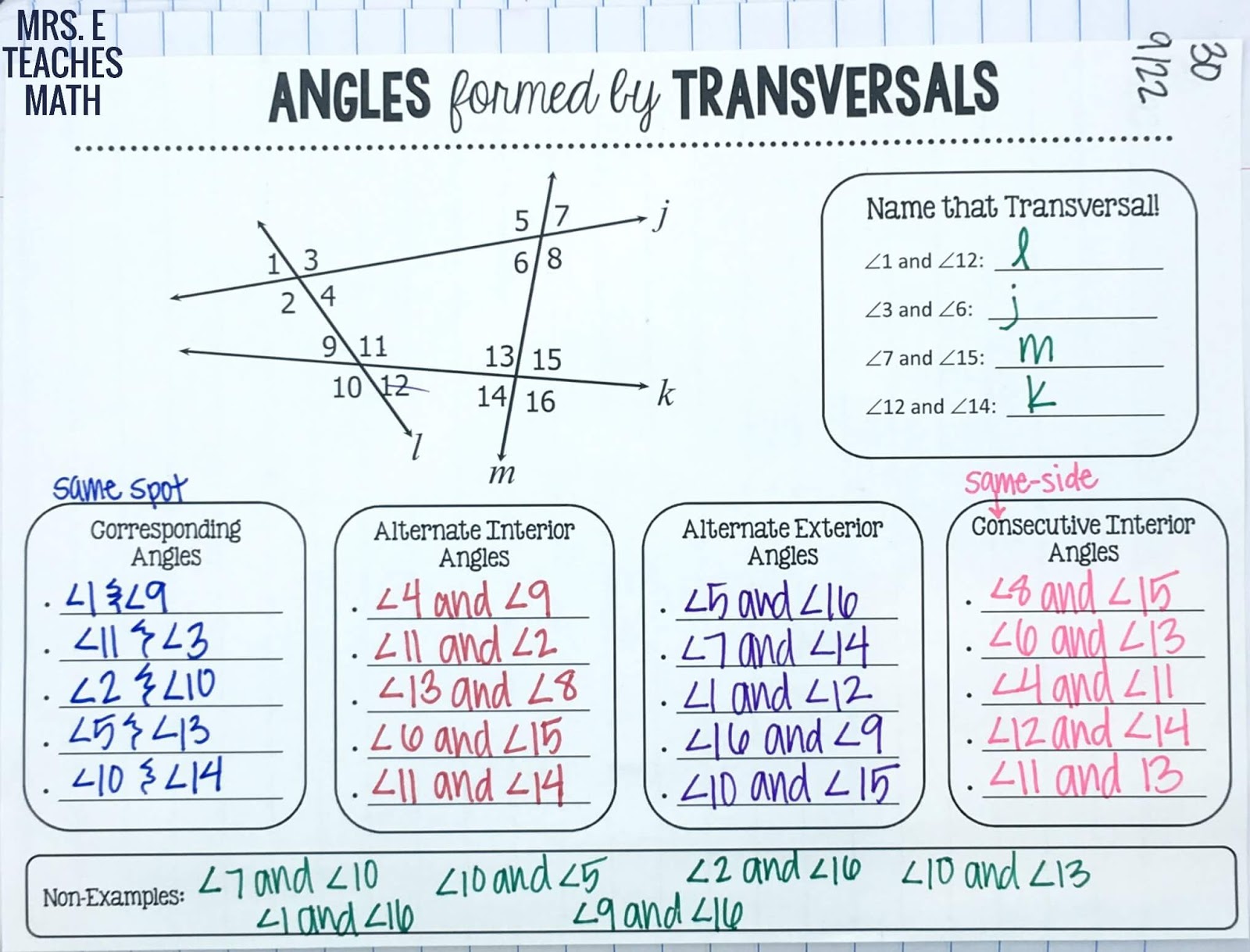
One way to prove parallel lines is by using corresponding angles. Corresponding angles are angles that have the same relative position in two different lines. If two lines intersect a third line (called the transversal), and the corresponding angles are congruent, then the two lines are parallel.
📝 Note: Congruent angles have the same measure.
For example, if we have two lines, AB and CD, and a transversal line EF intersects both lines, and ∠AEB = ∠CEF, then we can conclude that AB || CD.
Method 2: Alternate Interior Angles
Another way to prove parallel lines is by using alternate interior angles. Alternate interior angles are angles that are on opposite sides of the transversal and inside the two lines.
If two lines intersect a third line (the transversal), and the alternate interior angles are congruent, then the two lines are parallel.
For instance, if we have two lines, AB and CD, and a transversal line EF intersects both lines, and ∠AEB = ∠CEB, then we can conclude that AB || CD.
Method 3: Alternate Exterior Angles
Alternate exterior angles are angles that are on opposite sides of the transversal and outside the two lines. If two lines intersect a third line (the transversal), and the alternate exterior angles are congruent, then the two lines are parallel.
For example, if we have two lines, AB and CD, and a transversal line EF intersects both lines, and ∠AED = ∠CEA, then we can conclude that AB || CD.
Method 4: Consecutive Interior Angles
Consecutive interior angles are angles that are on the same side of the transversal and inside the two lines. If two lines intersect a third line (the transversal), and the consecutive interior angles are supplementary (add up to 180°), then the two lines are parallel.
For instance, if we have two lines, AB and CD, and a transversal line EF intersects both lines, and ∠AEB + ∠CEB = 180°, then we can conclude that AB || CD.
Method 5: Transversal Lines
A transversal line is a line that intersects two or more lines. If a transversal line intersects two lines, and the transversal line is perpendicular to both lines, then the two lines are parallel.
For example, if we have two lines, AB and CD, and a transversal line EF intersects both lines, and EF ⊥ AB and EF ⊥ CD, then we can conclude that AB || CD.
| Method | Description |
|---|---|
| Corresponding Angles | If corresponding angles are congruent, then the lines are parallel. |
| Alternate Interior Angles | If alternate interior angles are congruent, then the lines are parallel. |
| Alternate Exterior Angles | If alternate exterior angles are congruent, then the lines are parallel. |
| Consecutive Interior Angles | If consecutive interior angles are supplementary, then the lines are parallel. |
| Transversal Lines | If a transversal line is perpendicular to both lines, then the lines are parallel. |
To summarize, there are five ways to prove parallel lines, including using corresponding angles, alternate interior angles, alternate exterior angles, consecutive interior angles, and transversal lines. By applying these methods, we can establish the relationship between two lines and determine if they are parallel.
What is the definition of parallel lines?
+Parallel lines are lines that lie in the same plane and never intersect, no matter how far they are extended.
How many methods are there to prove parallel lines?
+There are five methods to prove parallel lines: corresponding angles, alternate interior angles, alternate exterior angles, consecutive interior angles, and transversal lines.
What is a transversal line?
+A transversal line is a line that intersects two or more lines.
Related Terms:
- Parallel lines Proofs Worksheet PDF
- Proofs with parallel lines
- Geometry Proofs Worksheet