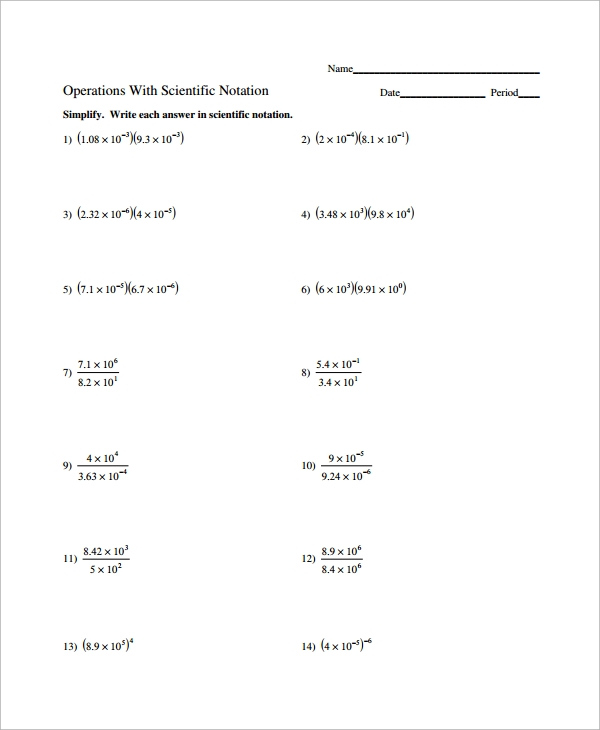
5 Ways to Master Linear Equations and Inequalities
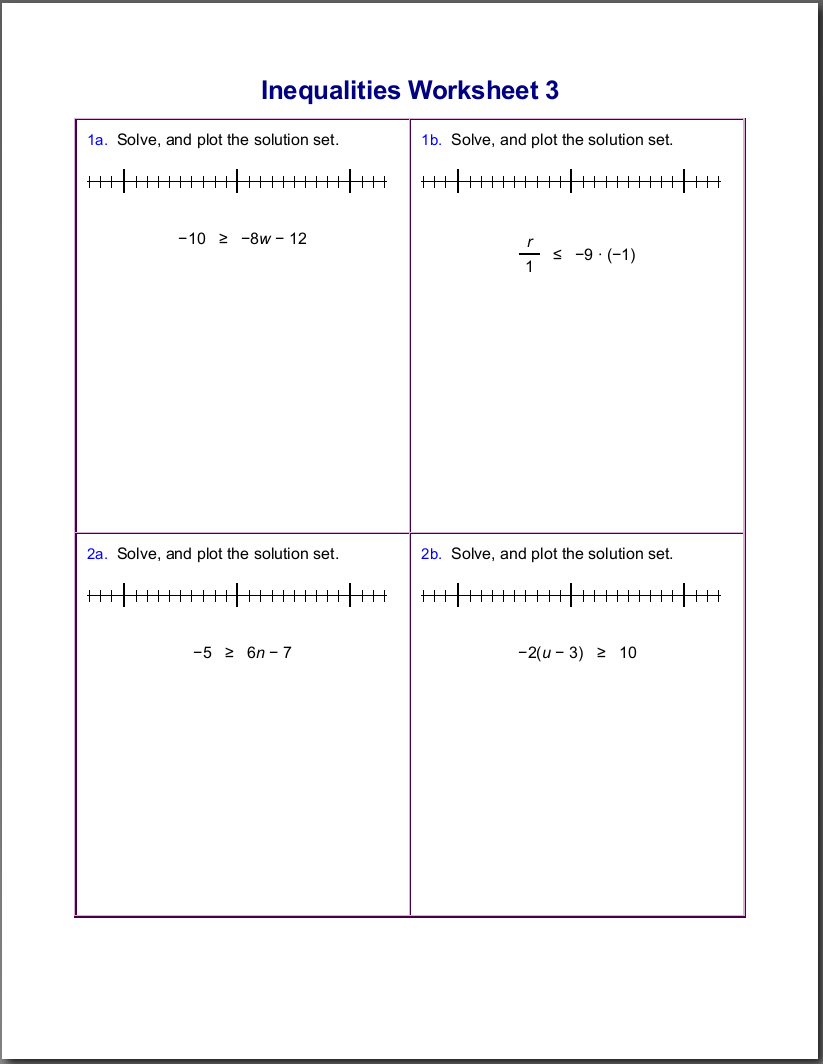
Understanding the Basics of Linear Equations and Inequalities
Linear equations and inequalities are fundamental concepts in mathematics, and mastering them is essential for solving various problems in algebra, geometry, and other branches of mathematics. A linear equation is an equation in which the highest power of the variable is 1, and it can be written in the form of ax + b = c, where a, b, and c are constants. On the other hand, a linear inequality is a statement that two expressions are not equal, and it can be written in the form of ax + b < c, ax + b > c, ax + b ≤ c, or ax + b ≥ c.
1. Learn the Different Types of Linear Equations and Inequalities
There are several types of linear equations and inequalities, and understanding each type is crucial for mastering them. Here are some of the most common types:
- Simple Linear Equations: These are linear equations that have only one variable. For example, 2x + 3 = 5.
- Linear Equations with Two Variables: These are linear equations that have two variables. For example, x + 2y = 4.
- Linear Inequalities: These are statements that two expressions are not equal. For example, 2x + 3 > 5.
- Compound Linear Inequalities: These are linear inequalities that have two or more inequality signs. For example, 2x + 3 > 5 and x - 2 < 3.
2. Understand the Properties of Linear Equations and Inequalities
Linear equations and inequalities have several properties that make them useful for solving problems. Here are some of the most important properties:
- Addition Property: The addition property states that if a = b, then a + c = b + c.
- Subtraction Property: The subtraction property states that if a = b, then a - c = b - c.
- Multiplication Property: The multiplication property states that if a = b, then ac = bc.
- Division Property: The division property states that if a = b, then a/c = b/c.
3. Learn How to Solve Linear Equations and Inequalities
Solving linear equations and inequalities involves isolating the variable and finding its value. Here are some steps to follow:
- Add or subtract the same value to both sides: This is used to isolate the variable.
- Multiply or divide both sides by the same value: This is used to get rid of fractions or decimals.
- Use inverse operations: This is used to isolate the variable.
📝 Note: When solving linear inequalities, it's essential to flip the inequality sign when dividing or multiplying both sides by a negative number.
4. Practice, Practice, Practice!
Practice is key to mastering linear equations and inequalities. Here are some tips to help you practice:
- Start with simple problems: Begin with simple linear equations and inequalities and gradually move on to more complex ones.
- Use online resources: There are many online resources available that provide practice problems and solutions.
- Take your time: Don’t rush through problems. Take your time to think through each step.
5. Apply Linear Equations and Inequalities to Real-Life Situations
Linear equations and inequalities are used to model real-life situations, such as finance, physics, and engineering. Here are some examples:
- Finance: Linear equations and inequalities can be used to calculate interest rates, investment returns, and loan payments.
- Physics: Linear equations and inequalities can be used to model the motion of objects, including distance, speed, and acceleration.
- Engineering: Linear equations and inequalities can be used to design systems, including electronic circuits and mechanical systems.
By following these five steps, you can master linear equations and inequalities and improve your problem-solving skills.
To summarize, mastering linear equations and inequalities requires a deep understanding of the basics, practice, and application to real-life situations. By following these steps, you can become proficient in solving linear equations and inequalities and improve your problem-solving skills.
What is the difference between a linear equation and a linear inequality?
+A linear equation is an equation in which the highest power of the variable is 1, and it can be written in the form of ax + b = c. A linear inequality is a statement that two expressions are not equal, and it can be written in the form of ax + b < c, ax + b > c, ax + b ≤ c, or ax + b ≥ c.
How do I solve a linear equation?
+To solve a linear equation, you need to isolate the variable and find its value. You can do this by adding or subtracting the same value to both sides, multiplying or dividing both sides by the same value, and using inverse operations.
What are some real-life applications of linear equations and inequalities?
+Linear equations and inequalities are used to model real-life situations, such as finance, physics, and engineering. They can be used to calculate interest rates, investment returns, and loan payments, as well as to model the motion of objects and design systems.
Related Terms:
- Linear Equations Worksheet PDF
- Linear equations and Inequalities PDF
- Graphing Linear Inequalities Worksheet PDF