5 Tips to Master Logarithms Worksheet Answer Key
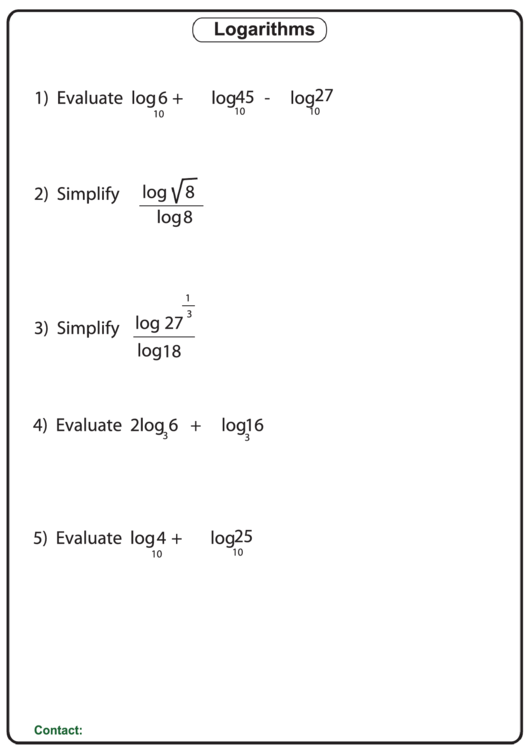
Mastering Logarithms: A Comprehensive Guide
Logarithms are a fundamental concept in mathematics, and mastering them is crucial for success in various fields, including mathematics, physics, engineering, and computer science. In this article, we will provide you with 5 tips to help you master logarithms and solve logarithmic problems with ease.
Tip 1: Understand the Basics of Logarithms
Before diving into complex logarithmic problems, it’s essential to understand the basics of logarithms. A logarithm is the inverse operation of exponentiation. In other words, logarithms answer the question, “To what power must a base number be raised to obtain a given value?” For example, the logarithm of 100 with a base of 10 is 2, because 10^2 = 100.
Key Terms:
- Base: The number that is raised to a power to produce a given value.
- Argument: The value for which the logarithm is calculated.
- Result: The power to which the base must be raised to produce the argument.
Tip 2: Learn the Logarithmic Identities
Logarithmic identities are essential for solving logarithmic problems. Here are some of the most common logarithmic identities:
- Product Rule: log(a × b) = log(a) + log(b)
- Quotient Rule: log(a ÷ b) = log(a) - log(b)
- Power Rule: log(a^n) = n × log(a)
Example:
Using the product rule, we can simplify the expression log(2 × 3) as follows:
log(2 × 3) = log(2) + log(3)
Tip 3: Practice Solving Logarithmic Equations
To become proficient in solving logarithmic equations, practice is key. Here are some examples of logarithmic equations:
- Simple Equations: log(2x) = 3
- Compound Equations: log(2x + 1) = 2
- Equations with Multiple Logarithms: log(x) + log(2x) = 5
Example:
Solve the equation log(2x) = 3.
Using the definition of a logarithm, we can rewrite the equation as:
2x = 10^3
x = 1000⁄2
x = 500
Tip 4: Learn to Graph Logarithmic Functions
Graphing logarithmic functions can help you visualize the relationship between the base, argument, and result. Here are some key features of logarithmic graphs:
- Domain: The set of all possible arguments.
- Range: The set of all possible results.
- x-intercept: The point at which the graph crosses the x-axis.
- y-intercept: The point at which the graph crosses the y-axis.
Example:
Graph the function f(x) = log(x).
The graph of the function f(x) = log(x) has a domain of all positive real numbers and a range of all real numbers. The x-intercept is at (1, 0), and the y-intercept is at (0, undefined).
Tip 5: Use Logarithmic Properties to Simplify Complex Expressions
Logarithmic properties can be used to simplify complex expressions. Here are some examples:
- Simplifying a Product: log(2^3 × 3^2) = log(2^3) + log(3^2) = 3 × log(2) + 2 × log(3)
- Simplifying a Quotient: log(2^3 ÷ 3^2) = log(2^3) - log(3^2) = 3 × log(2) - 2 × log(3)
Example:
Simplify the expression log(2^3 × 3^2).
Using the product rule, we can simplify the expression as follows:
log(2^3 × 3^2) = log(2^3) + log(3^2) = 3 × log(2) + 2 × log(3)
💡 Note: These 5 tips are just the beginning. Practice is key to mastering logarithms. Be sure to work through plenty of examples and exercises to become proficient in solving logarithmic problems.
What is the definition of a logarithm?
+A logarithm is the inverse operation of exponentiation. It answers the question, "To what power must a base number be raised to obtain a given value?"
What are the three main logarithmic identities?
+The three main logarithmic identities are the product rule, quotient rule, and power rule.
How do I graph a logarithmic function?
+To graph a logarithmic function, identify the domain, range, x-intercept, and y-intercept. Use this information to sketch the graph.
By following these 5 tips and practicing regularly, you’ll become proficient in solving logarithmic problems and mastering the concept of logarithms.