Interior Angles Worksheet Answers

Understanding Interior Angles: A Comprehensive Guide
When it comes to geometry, understanding interior angles is crucial for solving problems related to triangles, quadrilaterals, and other polygons. In this guide, we will delve into the world of interior angles, exploring what they are, how to calculate them, and providing answers to common worksheet questions.
What are Interior Angles?
An interior angle is an angle inside a polygon, formed by two adjacent sides. In the case of a triangle, the interior angles are the angles inside the triangle, formed by the three sides. For a quadrilateral, the interior angles are the angles inside the shape, formed by the four sides.
How to Calculate Interior Angles
Calculating interior angles is relatively straightforward. The sum of the interior angles of a triangle is always 180 degrees. For a quadrilateral, the sum of the interior angles is 360 degrees. To calculate the interior angle of a specific polygon, you can use the following formula:
Sum of Interior Angles = (n - 2) x 180
where n is the number of sides of the polygon.
For example, if we want to calculate the sum of the interior angles of a hexagon (a polygon with 6 sides), we can plug in the value of n:
Sum of Interior Angles = (6 - 2) x 180 Sum of Interior Angles = 4 x 180 Sum of Interior Angles = 720
Interior Angles Worksheet Answers
Here are the answers to some common interior angles worksheet questions:
1. What is the sum of the interior angles of a triangle? Answer: 180 degrees
2. What is the sum of the interior angles of a quadrilateral? Answer: 360 degrees
3. What is the sum of the interior angles of a hexagon? Answer: 720 degrees
4. If one angle of a triangle is 60 degrees, and another angle is 80 degrees, what is the third angle? Answer: 40 degrees (since the sum of the interior angles of a triangle is 180 degrees)
5. If a quadrilateral has two right angles (90 degrees each), what is the sum of the other two angles? Answer: 180 degrees (since the sum of the interior angles of a quadrilateral is 360 degrees)
🤔 Note: When solving interior angle problems, always make sure to check your units and ensure that you are working with degrees.
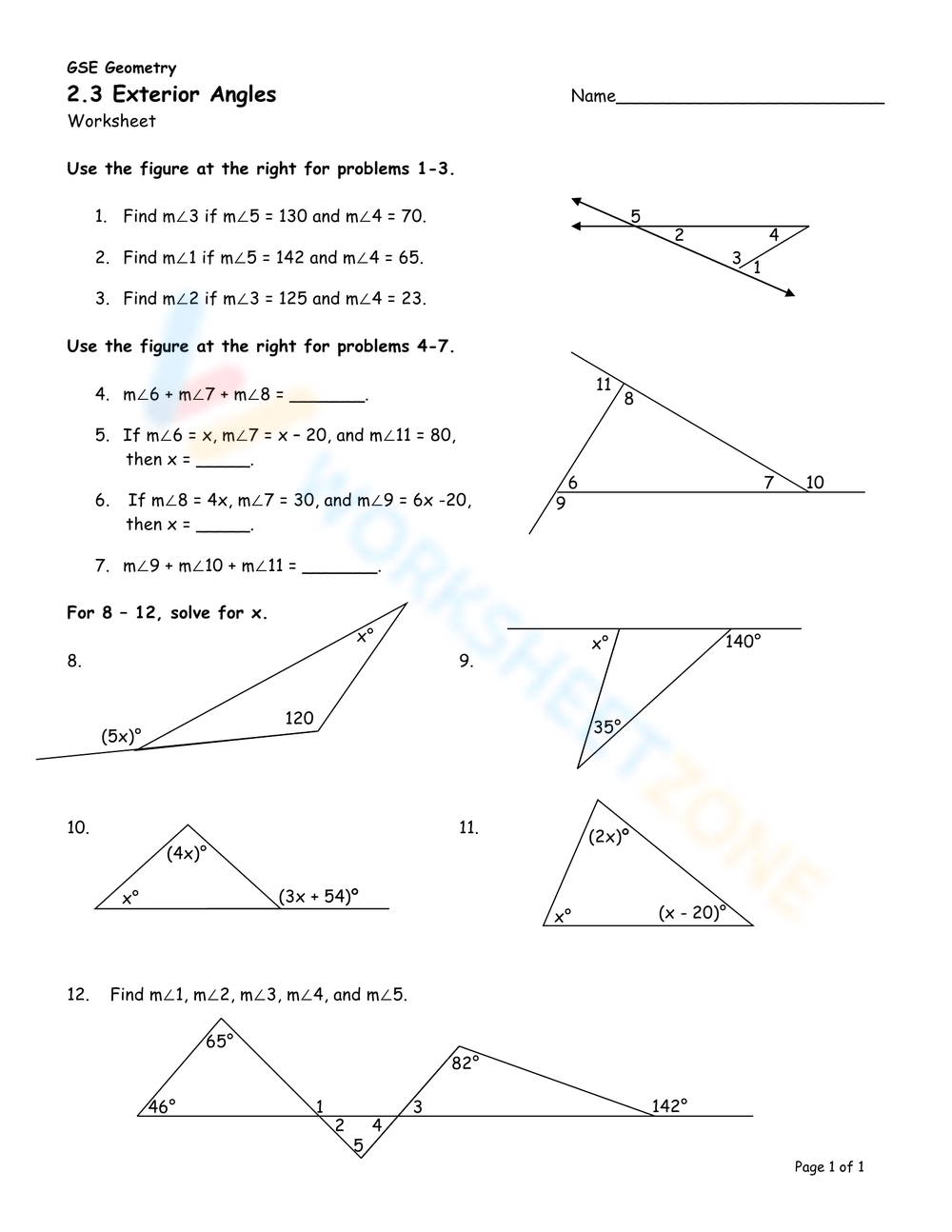
Interior Angles Table
Here is a table summarizing the sum of interior angles for different polygons:
| Polygon | Number of Sides | Sum of Interior Angles |
|---|---|---|
| Triangle | 3 | 180 degrees |
| Quadrilateral | 4 | 360 degrees |
| Pentagon | 5 | 540 degrees |
| Hexagon | 6 | 720 degrees |
In conclusion, understanding interior angles is essential for solving geometry problems. By using the formula for calculating the sum of interior angles and practicing with worksheet questions, you can become more confident in your ability to solve interior angle problems.
What is the difference between an interior angle and an exterior angle?
+An interior angle is an angle inside a polygon, while an exterior angle is an angle outside a polygon.
Can the sum of interior angles of a polygon be negative?
+No, the sum of interior angles of a polygon is always positive.
How do I calculate the interior angle of a regular polygon?
+To calculate the interior angle of a regular polygon, divide the sum of the interior angles by the number of sides.
Related Terms:
- Exterior angles Worksheet Answers
- Geometry interior angles worksheet answers
- Interior angles questions and answers
- Exterior angles of polygons worksheet