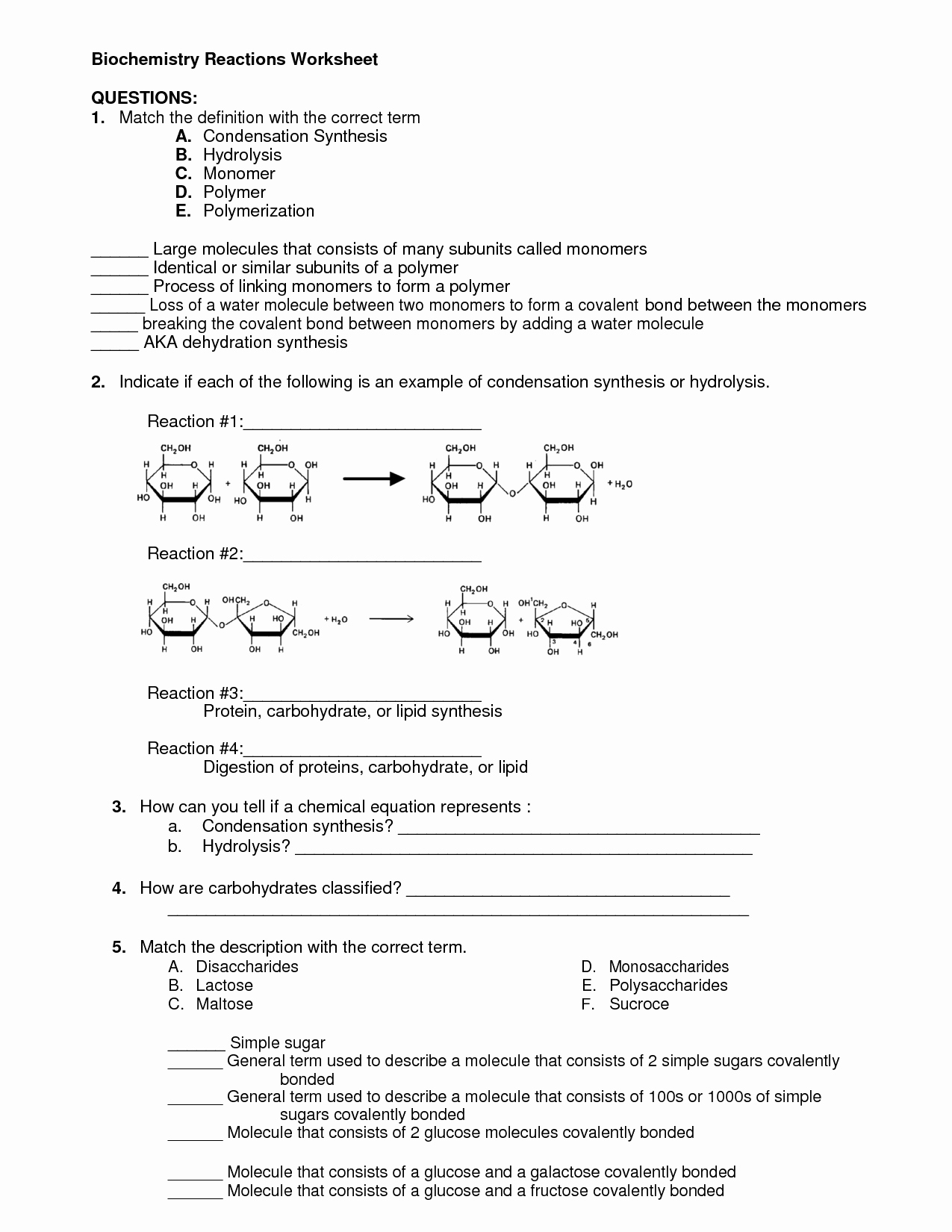
Prove Lines Parallel Worksheet Help and Answers

Determining whether two lines are parallel can be a straightforward task in geometry, especially when you have the right tools and strategies. Here’s a comprehensive guide to help you tackle proving lines parallel worksheet problems with ease.

Understanding Parallel Lines

Before diving into the worksheet, let’s quickly review what parallel lines are. In geometry, two lines are considered parallel if they lie in the same plane and never intersect, no matter how far they are extended. In other words, parallel lines have the same slope but different y-intercepts.

Methods for Proving Lines Parallel

There are several methods to prove that two lines are parallel, including:

• Corresponding Angles Theorem: If two lines are cut by a transversal and the corresponding angles are congruent, then the lines are parallel.
• Alternate Interior Angles Theorem: If two lines are cut by a transversal and the alternate interior angles are congruent, then the lines are parallel.
• Consecutive Interior Angles Theorem: If two lines are cut by a transversal and the consecutive interior angles are supplementary (add up to 180°), then the lines are parallel.
• Slope-Intercept Form: If two lines have the same slope but different y-intercepts, then they are parallel.

Worksheet Help and Answers

Here’s a sample worksheet with solutions to help you better understand how to prove lines parallel:

Problem 1

In the diagram below, prove that lines AB and CD are parallel.

[Image: Diagram showing lines AB and CD cut by a transversal]

Solution

Using the Corresponding Angles Theorem, we can see that ∠1 ≅ ∠5 and ∠2 ≅ ∠6. Since the corresponding angles are congruent, lines AB and CD are parallel.

Problem 2

In the diagram below, prove that lines EF and GH are parallel.

[Image: Diagram showing lines EF and GH cut by a transversal]

Solution

Using the Alternate Interior Angles Theorem, we can see that ∠3 ≅ ∠7 and ∠4 ≅ ∠8. Since the alternate interior angles are congruent, lines EF and GH are parallel.

Problem 3

In the diagram below, prove that lines JK and LM are parallel.

[Image: Diagram showing lines JK and LM cut by a transversal]

Solution

Using the Consecutive Interior Angles Theorem, we can see that ∠9 + ∠10 = 180°. Since the consecutive interior angles are supplementary, lines JK and LM are parallel.

Problem 4

Prove that the lines represented by the equations y = 2x + 1 and y = 2x + 3 are parallel.

Solution

Since both lines have the same slope (m = 2) but different y-intercepts (b = 1 and b = 3), they are parallel.

Notes

📝 Note: When using the slope-intercept form to prove lines parallel, make sure to check that the slopes are equal and the y-intercepts are different.

📝 Note: When using the theorems to prove lines parallel, make sure to identify the corresponding angles, alternate interior angles, or consecutive interior angles correctly.

Conclusion

Proving lines parallel is a fundamental concept in geometry, and with practice, you’ll become more confident in your ability to tackle these types of problems. Remember to use the theorems and formulas provided to help you solve the worksheet problems, and don’t hesitate to ask for help if you need it. Happy problem-solving!