Master the Distributive Property with Practice Worksheets
Understanding the Distributive Property
The distributive property is a fundamental concept in mathematics that helps simplify expressions and equations. It states that a single term can be distributed to multiple terms inside parentheses, which allows us to expand and simplify expressions more easily.
What is the Distributive Property?
The distributive property is a rule that states that for any numbers a, b, and c:
a(b + c) = ab + ac
This means that when a single term is multiplied by a group of terms inside parentheses, we can distribute the multiplication to each term inside the parentheses.
Examples of the Distributive Property
Let’s take a look at some examples to illustrate how the distributive property works:
- 2(x + 3) = 2x + 6
- 3(2x - 4) = 6x - 12
- x(2 + 5) = 2x + 5x
In each of these examples, we can see how the distributive property allows us to expand and simplify the expression.
Why is the Distributive Property Important?
The distributive property is essential in mathematics because it helps us:
- Simplify complex expressions and equations
- Expand and factor expressions
- Solve linear equations and inequalities
- Work with algebraic expressions and equations
Without the distributive property, many mathematical operations would be much more difficult to perform.
How to Use the Distributive Property
Using the distributive property is relatively straightforward. Here are the steps to follow:
- Identify the expression or equation that you want to simplify.
- Look for any parentheses or groups of terms that can be distributed.
- Apply the distributive property by multiplying the term outside the parentheses to each term inside the parentheses.
- Simplify the resulting expression.
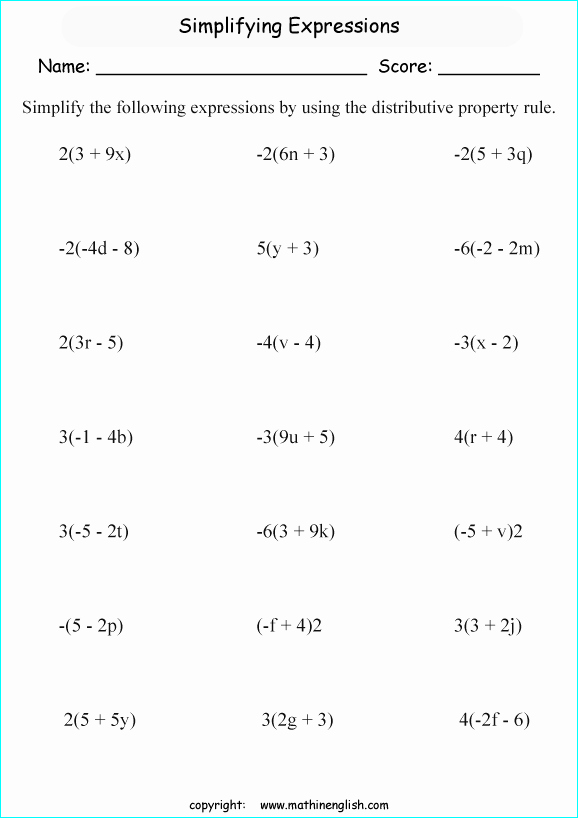
Practice Worksheets
To master the distributive property, practice is key. Here are some practice worksheets to help you get started:
Worksheet 1: Simple Distributive Property

| Expression | Expanded Form |
|---|---|
| 2(x + 3) | |
| 3(2x - 4) | |
| x(2 + 5) |
Worksheet 2: Distributive Property with Variables
| Expression | Expanded Form |
|---|---|
| x(2y + 3z) | |
| 2x(3y - 2z) | |
| 3x(2y + 4z) |
Worksheet 3: Distributive Property with Fractions
| Expression | Expanded Form |
|---|---|
| (1⁄2)(x + 3) | |
| (3⁄4)(2x - 4) | |
| (2⁄3)(x + 5) |
Answers to Practice Worksheets
Worksheet 1: Simple Distributive Property
| Expression | Expanded Form |
|---|---|
| 2(x + 3) | 2x + 6 |
| 3(2x - 4) | 6x - 12 |
| x(2 + 5) | 2x + 5x |
Worksheet 2: Distributive Property with Variables
| Expression | Expanded Form |
|---|---|
| x(2y + 3z) | 2xy + 3xz |
| 2x(3y - 2z) | 6xy - 4xz |
| 3x(2y + 4z) | 6xy + 12xz |
Worksheet 3: Distributive Property with Fractions
| Expression | Expanded Form |
|---|---|
| (1⁄2)(x + 3) | (1⁄2)x + 3⁄2 |
| (3⁄4)(2x - 4) | 3/2x - 3 |
| (2⁄3)(x + 5) | (2⁄3)x + 10⁄3 |
Notes
- Make sure to apply the distributive property to each term inside the parentheses.
- Simplify the resulting expression by combining like terms.
- Practice, practice, practice! The more you practice, the more comfortable you’ll become with using the distributive property.
Conclusion
The distributive property is a powerful tool in mathematics that helps us simplify expressions and equations. With practice and patience, you can master the distributive property and become more confident in your math skills. Remember to apply the distributive property to each term inside the parentheses and simplify the resulting expression.
What is the distributive property?
+The distributive property is a rule that states that for any numbers a, b, and c: a(b + c) = ab + ac.
Why is the distributive property important?
+The distributive property is essential in mathematics because it helps us simplify complex expressions and equations, expand and factor expressions, solve linear equations and inequalities, and work with algebraic expressions and equations.
How do I use the distributive property?
+To use the distributive property, identify the expression or equation that you want to simplify, look for any parentheses or groups of terms that can be distributed, apply the distributive property by multiplying the term outside the parentheses to each term inside the parentheses, and simplify the resulting expression.
Related Terms:
- Easy Distributive Property Worksheet PDF