Graphing Linear Equations in Standard Form Made Easy
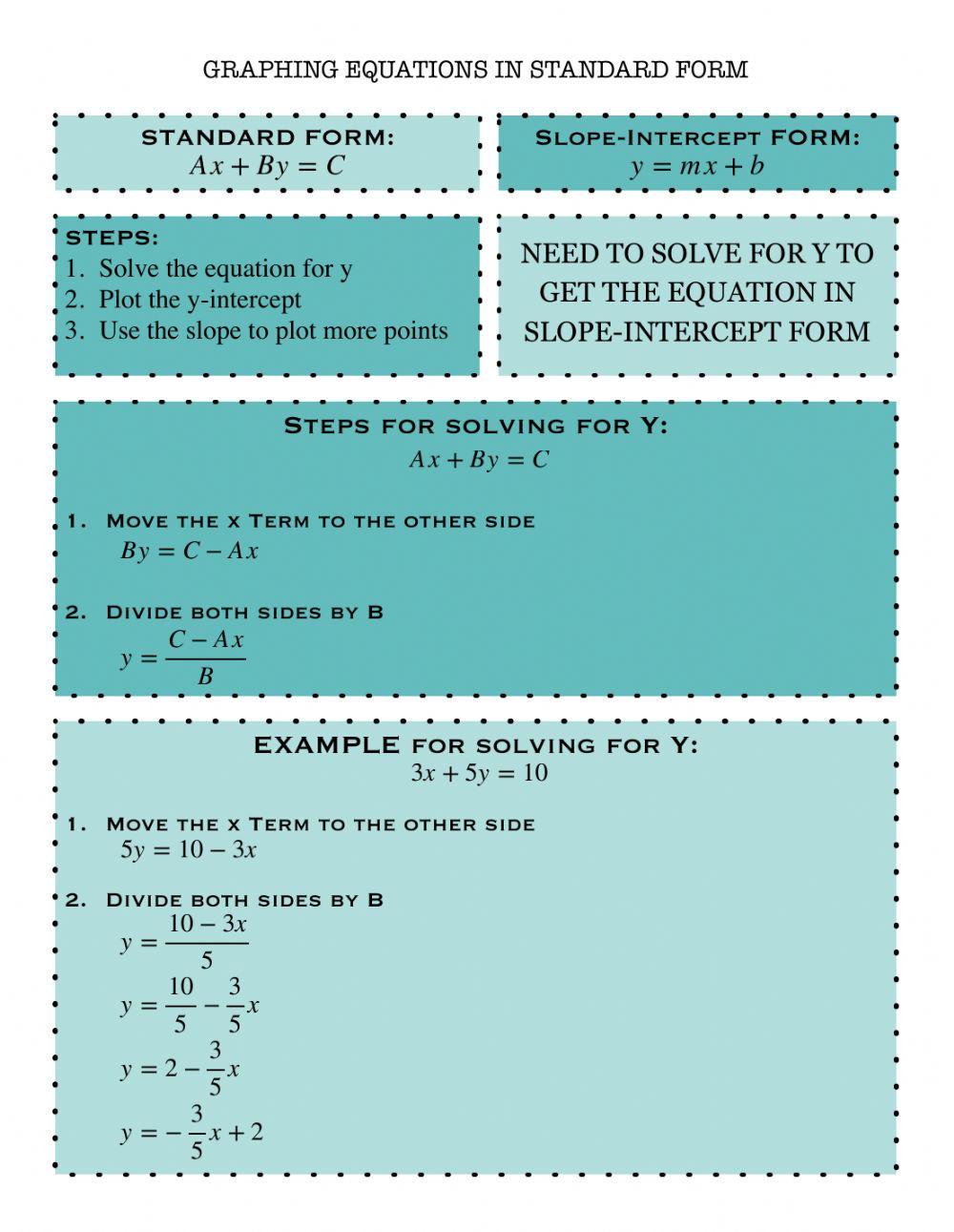
Understanding Standard Form Linear Equations
Linear equations are a fundamental concept in algebra, and understanding how to graph them is essential for problem-solving. One of the most common forms of linear equations is the standard form, which is represented by the equation ax + by = c. In this article, we will explore the concept of standard form linear equations and provide a step-by-step guide on how to graph them.
What is Standard Form?
Standard form linear equations are written in the form ax + by = c, where a, b, and c are constants, and x and y are the variables. The coefficients a and b represent the slope and y-intercept of the line, respectively. The constant term c represents the x-intercept.
How to Graph Standard Form Linear Equations
Graphing standard form linear equations involves finding the x and y intercepts and plotting them on a coordinate plane.
📝 Note: To graph a linear equation in standard form, you need to have a solid understanding of the x and y axes and the concept of slope.
Here’s a step-by-step guide on how to graph standard form linear equations:
- Step 1: Find the x-intercept To find the x-intercept, set y = 0 and solve for x.
- Step 2: Find the y-intercept To find the y-intercept, set x = 0 and solve for y.
- Step 3: Plot the intercepts Plot the x and y intercepts on a coordinate plane.
- Step 4: Draw the line Draw a line through the intercepts to create the graph of the linear equation.
Example 1: Graphing a Linear Equation in Standard Form
Let’s graph the linear equation 2x + 3y = 6.
- Step 1: Find the x-intercept Set y = 0 and solve for x: 2x + 3(0) = 6 → 2x = 6 → x = 3
- Step 2: Find the y-intercept Set x = 0 and solve for y: 2(0) + 3y = 6 → 3y = 6 → y = 2
- Step 3: Plot the intercepts Plot the x-intercept (3, 0) and y-intercept (0, 2) on a coordinate plane.
- Step 4: Draw the line Draw a line through the intercepts to create the graph of the linear equation.
| x | y |
|---|---|
| 3 | 0 |
| 0 | 2 |
Example 2: Graphing a Linear Equation with a Negative Slope
Let’s graph the linear equation -2x + 4y = 8.
- Step 1: Find the x-intercept Set y = 0 and solve for x: -2x + 4(0) = 8 → -2x = 8 → x = -4
- Step 2: Find the y-intercept Set x = 0 and solve for y: -2(0) + 4y = 8 → 4y = 8 → y = 2
- Step 3: Plot the intercepts Plot the x-intercept (-4, 0) and y-intercept (0, 2) on a coordinate plane.
- Step 4: Draw the line Draw a line through the intercepts to create the graph of the linear equation.
| x | y |
|---|---|
| -4 | 0 |
| 0 | 2 |
In conclusion, graphing linear equations in standard form requires finding the x and y intercepts and plotting them on a coordinate plane. By following these steps, you can easily graph any linear equation in standard form.
What is the standard form of a linear equation?
+The standard form of a linear equation is ax + by = c, where a, b, and c are constants, and x and y are the variables.
How do I find the x-intercept of a linear equation?
+To find the x-intercept, set y = 0 and solve for x.
How do I graph a linear equation in standard form?
+To graph a linear equation in standard form, find the x and y intercepts and plot them on a coordinate plane. Then, draw a line through the intercepts to create the graph of the linear equation.