6 Ways to Master Improper Fractions and Mixed Numbers
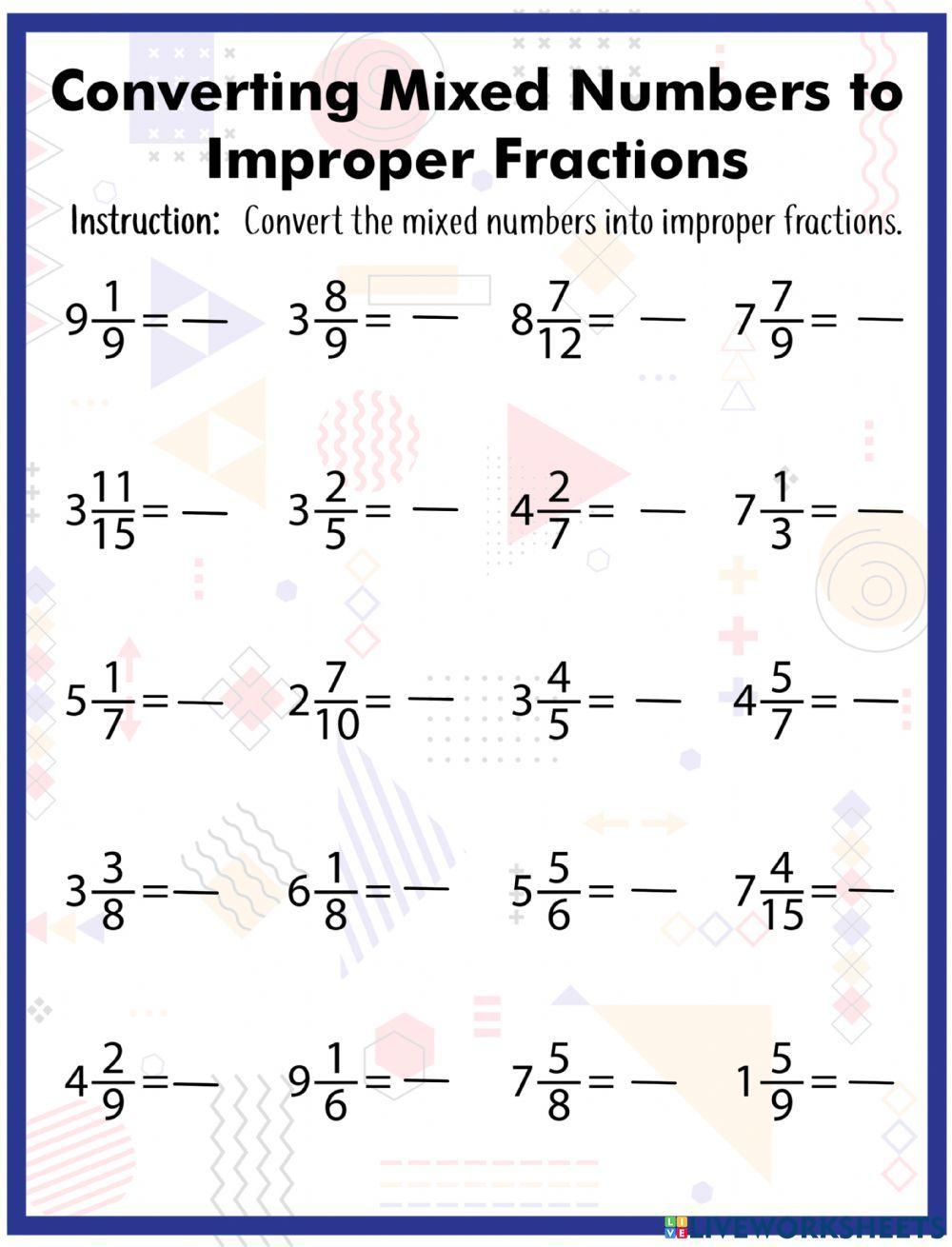
Understanding the Basics of Improper Fractions and Mixed Numbers
Improper fractions and mixed numbers are essential concepts in mathematics, particularly in the realm of fractions. An improper fraction is a type of fraction where the numerator is greater than or equal to the denominator, whereas a mixed number is a combination of a whole number and a proper fraction. Mastering these concepts is crucial for problem-solving and critical thinking in mathematics.
1. Converting Improper Fractions to Mixed Numbers
Converting improper fractions to mixed numbers is a fundamental skill that can be achieved by following a simple process:
- Divide the numerator by the denominator to obtain the quotient and remainder.
- The quotient becomes the whole number part of the mixed number.
- The remainder becomes the new numerator, while the denominator remains the same.
For example, convert the improper fraction 11⁄4 to a mixed number:

| 4 | 2 | 4 |
The result is 2 3⁄4, which is the mixed number equivalent of the improper fraction 11⁄4.
🤔 Note: When converting improper fractions to mixed numbers, it's essential to ensure that the remainder is less than the denominator.
2. Converting Mixed Numbers to Improper Fractions
Converting mixed numbers to improper fractions is a straightforward process that can be achieved by following these steps:
- Multiply the whole number part by the denominator.
- Add the numerator to the product obtained in the previous step.
- Write the result as the new numerator, while keeping the same denominator.
For example, convert the mixed number 2 3⁄4 to an improper fraction:
- Multiply the whole number part (2) by the denominator (4): 2 × 4 = 8
- Add the numerator (3) to the product: 8 + 3 = 11
- Write the result as the new numerator: 11⁄4
The result is 11⁄4, which is the improper fraction equivalent of the mixed number 2 3⁄4.
3. Adding and Subtracting Improper Fractions and Mixed Numbers
Adding and subtracting improper fractions and mixed numbers can be done by following these steps:
- Convert the mixed numbers to improper fractions, if necessary.
- Find the least common multiple (LCM) of the denominators.
- Add or subtract the numerators, while keeping the same denominator.
For example, add the improper fractions 7⁄4 and 3⁄4:
| 7 | + | 3 |
| 10 |
The result is 10⁄4, which can be simplified to 2 2⁄4 or further reduced to 2 1⁄2.
4. Multiplying Improper Fractions and Mixed Numbers
Multiplying improper fractions and mixed numbers can be done by following these steps:
- Convert the mixed numbers to improper fractions, if necessary.
- Multiply the numerators and denominators separately.
- Simplify the result, if possible.
For example, multiply the improper fractions 7⁄4 and 3⁄4:
| 7 | × | 3 |
| 21 |
| 4 | × | 4 |
| 16 |
The result is 21⁄16, which can be simplified to 1 5⁄16.
5. Dividing Improper Fractions and Mixed Numbers
Dividing improper fractions and mixed numbers can be done by following these steps:
- Convert the mixed numbers to improper fractions, if necessary.
- Invert the second fraction (i.e., flip the numerator and denominator).
- Multiply the fractions.
- Simplify the result, if possible.
For example, divide the improper fractions 7⁄4 by 3⁄4:
| 7 | × | 4 |
| 28 |
| 4 | × | 3 |
| 12 |
The result is 28⁄12, which can be simplified to 2 2⁄3.
6. Real-World Applications of Improper Fractions and Mixed Numbers
Improper fractions and mixed numbers have numerous real-world applications, including:
- Measuring ingredients for cooking and baking
- Calculating distances and speeds
- Determining quantities of materials for construction and crafting
- Understanding musical rhythms and time signatures
Mastering improper fractions and mixed numbers can help individuals develop problem-solving skills, critical thinking, and analytical reasoning.
As we master improper fractions and mixed numbers, we can apply these concepts to a wide range of real-world situations, making us more proficient and confident in our mathematical abilities.
What is the difference between an improper fraction and a mixed number?
+An improper fraction is a type of fraction where the numerator is greater than or equal to the denominator, whereas a mixed number is a combination of a whole number and a proper fraction.
How do you convert an improper fraction to a mixed number?
+To convert an improper fraction to a mixed number, divide the numerator by the denominator to obtain the quotient and remainder. The quotient becomes the whole number part of the mixed number, while the remainder becomes the new numerator.
What is the least common multiple (LCM) of two denominators?
+The least common multiple (LCM) of two denominators is the smallest multiple that both denominators share. It is used to add and subtract fractions with different denominators.
Related Terms:
- Subtracting fractions Worksheet
- Fraction Worksheet Grade 5
- Comparing fractions Worksheet grade 4
- Estimate and measure length worksheet