Graphing Inequalities in Two Variables Made Easy

Understanding the Basics of Graphing Inequalities in Two Variables

Graphing inequalities in two variables can be a daunting task for many students. However, with a clear understanding of the basics, it can become a manageable and even enjoyable process. In this article, we will explore the fundamentals of graphing inequalities in two variables, including the different types of inequalities, how to graph them, and some important notes to keep in mind.

Different Types of Inequalities

There are three main types of inequalities in two variables: linear inequalities, quadratic inequalities, and rational inequalities. Each type of inequality has its own unique characteristics and requires a different approach to graphing.

• Linear Inequalities: Linear inequalities are the simplest type of inequality and are represented by a linear equation with a less than, greater than, less than or equal to, or greater than or equal to symbol. For example: 2x + 3y > 5
• Quadratic Inequalities: Quadratic inequalities are represented by a quadratic equation with a less than, greater than, less than or equal to, or greater than or equal to symbol. For example: x^2 + 4y^2 < 16
• Rational Inequalities: Rational inequalities are represented by a rational expression with a less than, greater than, less than or equal to, or greater than or equal to symbol. For example: (x + 2)/(x - 3) > 0

Graphing Linear Inequalities

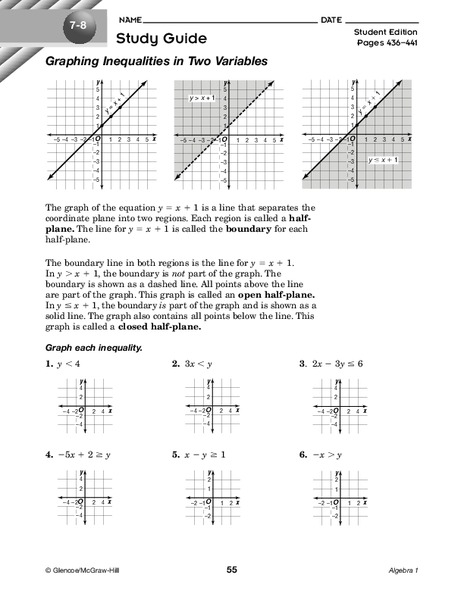
Graphing linear inequalities is a straightforward process that involves graphing the related linear equation and then shading the region that satisfies the inequality.

Step 1: Graph the Related Linear Equation

The first step in graphing a linear inequality is to graph the related linear equation. This can be done using a variety of methods, including the slope-intercept method, the point-slope method, or the standard form method.

Step 2: Choose a Test Point

Once the related linear equation has been graphed, choose a test point that is not on the line. This test point can be any point in the plane, but it is usually easiest to choose a point with integer coordinates.

Step 3: Substitute the Test Point into the Inequality

Substitute the test point into the inequality and simplify. If the resulting statement is true, then the region containing the test point is the solution region. If the resulting statement is false, then the region not containing the test point is the solution region.

Step 4: Shade the Solution Region

Finally, shade the solution region. If the inequality is a less than or greater than inequality, shade the region with a dashed line. If the inequality is a less than or equal to or greater than or equal to inequality, shade the region with a solid line.

🔹 Note: When graphing linear inequalities, it is essential to choose a test point that is not on the line. This ensures that the solution region is accurately shaded.

Graphing Quadratic Inequalities

Graphing quadratic inequalities is more complex than graphing linear inequalities and requires a different approach.

Step 1: Graph the Related Quadratic Equation

The first step in graphing a quadratic inequality is to graph the related quadratic equation. This can be done using a variety of methods, including the factoring method, the quadratic formula, or the graphing method.

Step 2: Identify the Axis of Symmetry

The axis of symmetry is a vertical line that passes through the vertex of the parabola. The axis of symmetry is essential in determining the solution region of the inequality.

Step 3: Choose a Test Point

Choose a test point that is not on the parabola. This test point can be any point in the plane, but it is usually easiest to choose a point with integer coordinates.

Step 4: Substitute the Test Point into the Inequality

Substitute the test point into the inequality and simplify. If the resulting statement is true, then the region containing the test point is the solution region. If the resulting statement is false, then the region not containing the test point is the solution region.

Step 5: Shade the Solution Region

Finally, shade the solution region. If the inequality is a less than or greater than inequality, shade the region with a dashed line. If the inequality is a less than or equal to or greater than or equal to inequality, shade the region with a solid line.

🔹 Note: When graphing quadratic inequalities, it is crucial to identify the axis of symmetry. This helps determine the solution region accurately.

Graphing Rational Inequalities

Graphing rational inequalities is similar to graphing quadratic inequalities and requires a different approach.

Step 1: Graph the Related Rational Expression

The first step in graphing a rational inequality is to graph the related rational expression. This can be done using a variety of methods, including the factoring method or the graphing method.

Step 2: Identify the Vertical Asymptotes

The vertical asymptotes are vertical lines that the rational expression approaches but never touches. The vertical asymptotes are essential in determining the solution region of the inequality.

Step 3: Choose a Test Point

Choose a test point that is not on the rational expression. This test point can be any point in the plane, but it is usually easiest to choose a point with integer coordinates.

Step 4: Substitute the Test Point into the Inequality

Substitute the test point into the inequality and simplify. If the resulting statement is true, then the region containing the test point is the solution region. If the resulting statement is false, then the region not containing the test point is the solution region.

Step 5: Shade the Solution Region

Finally, shade the solution region. If the inequality is a less than or greater than inequality, shade the region with a dashed line. If the inequality is a less than or equal to or greater than or equal to inequality, shade the region with a solid line.

🔹 Note: When graphing rational inequalities, it is crucial to identify the vertical asymptotes. This helps determine the solution region accurately.

To summarize the process of graphing inequalities in two variables:

• Understand the basics of inequalities, including the different types of inequalities.
• Graph the related equation or expression.
• Choose a test point that is not on the line or curve.
• Substitute the test point into the inequality and simplify.
• Shade the solution region.

By following these steps, graphing inequalities in two variables becomes a more manageable and even enjoyable process.

In conclusion, graphing inequalities in two variables requires a clear understanding of the basics and a different approach depending on the type of inequality. By following the steps outlined above and practicing regularly, students can become proficient in graphing inequalities and solve problems with confidence.