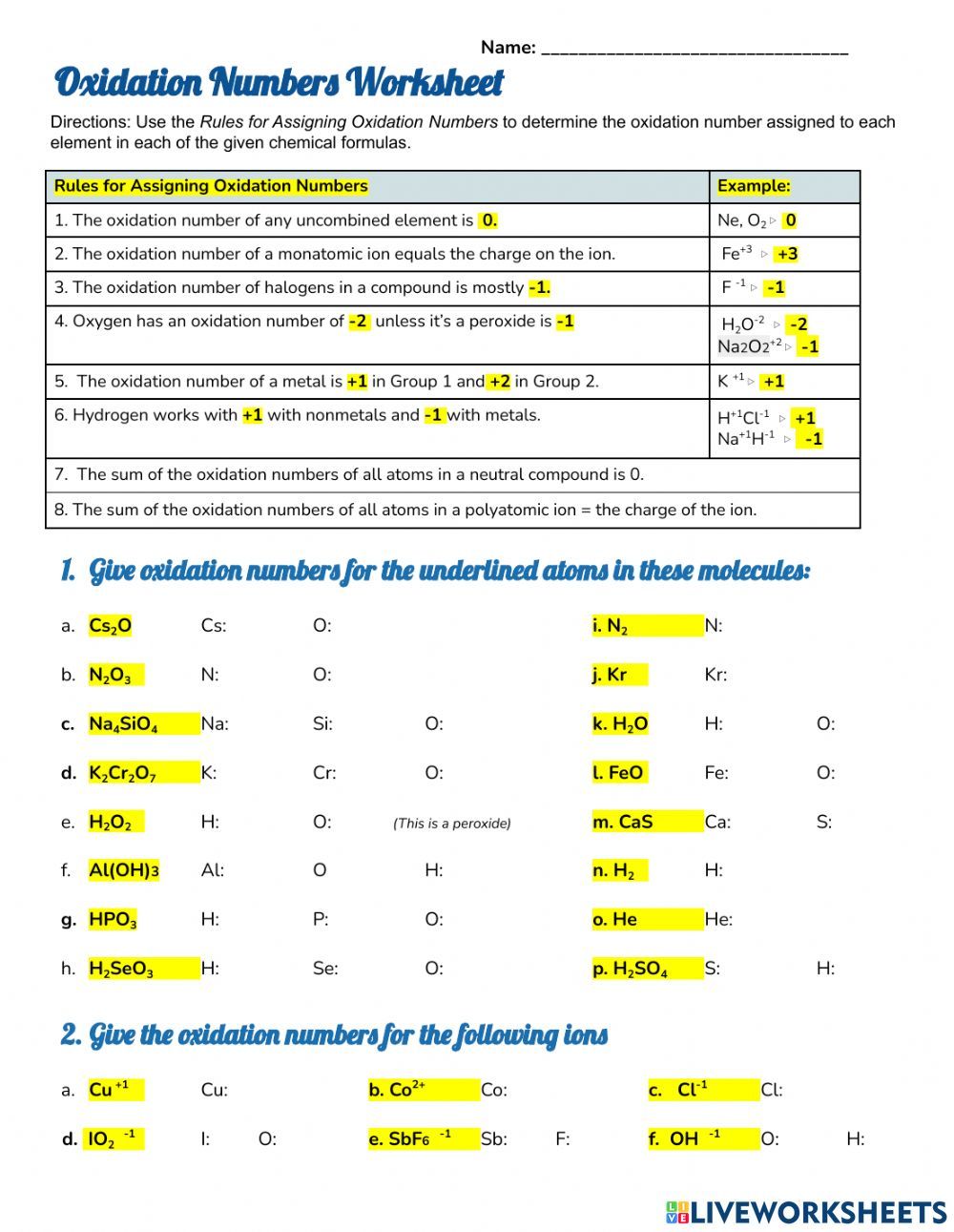
Geometric Mean Worksheet: Calculate and Understand with Ease
Understanding Geometric Mean: A Comprehensive Guide
Calculating geometric mean can be a daunting task, especially for those who are new to statistics and mathematics. However, with the right approach and practice, anyone can master this concept with ease. In this article, we will delve into the world of geometric mean, explore its definition, formula, and examples, and provide a worksheet to help you practice and reinforce your understanding.
What is Geometric Mean?
The geometric mean is a type of average that is used to calculate the average of a set of numbers that are meant to be multiplied together. It is different from the arithmetic mean, which is used to calculate the average of a set of numbers that are meant to be added together. The geometric mean is often used in finance, economics, and engineering to calculate the average return on investment, the average growth rate, and the average scale factor.
Geometric Mean Formula
The formula for calculating the geometric mean is as follows:
Geometric Mean (GM) = (n√(x1 × x2 × x3 ×… × xn))
Where:
- GM is the geometric mean
- n is the number of values
- x1, x2, x3,…, xn are the individual values
How to Calculate Geometric Mean
To calculate the geometric mean, follow these steps:
- Multiply all the values together.
- Take the nth root of the product, where n is the number of values.
- The result is the geometric mean.
📝 Note: The geometric mean can only be calculated for positive numbers. If there are any negative numbers or zeros in the dataset, the geometric mean cannot be calculated.
Examples of Geometric Mean
Here are a few examples to illustrate the concept of geometric mean:
Example 1:
A investment portfolio has the following returns over a period of 4 years:
- Year 1: 10%
- Year 2: 15%
- Year 3: 20%
- Year 4: 25%
To calculate the geometric mean return, we multiply the returns together:
10% × 15% × 20% × 25% = 7.5%
The geometric mean return is the 4th root of 7.5%, which is approximately 14.1%.
Example 2:
A company has the following sales figures over a period of 5 years:
- Year 1: $100,000
- Year 2: $120,000
- Year 3: $150,000
- Year 4: $180,000
- Year 5: $200,000
To calculate the geometric mean sales figure, we multiply the sales figures together:
100,000 × 120,000 × 150,000 × 180,000 × 200,000 = 432,000,000,000
The geometric mean sales figure is the 5th root of 432,000,000,000, which is approximately 149,908.
Geometric Mean Worksheet
Here is a worksheet to help you practice calculating geometric mean:
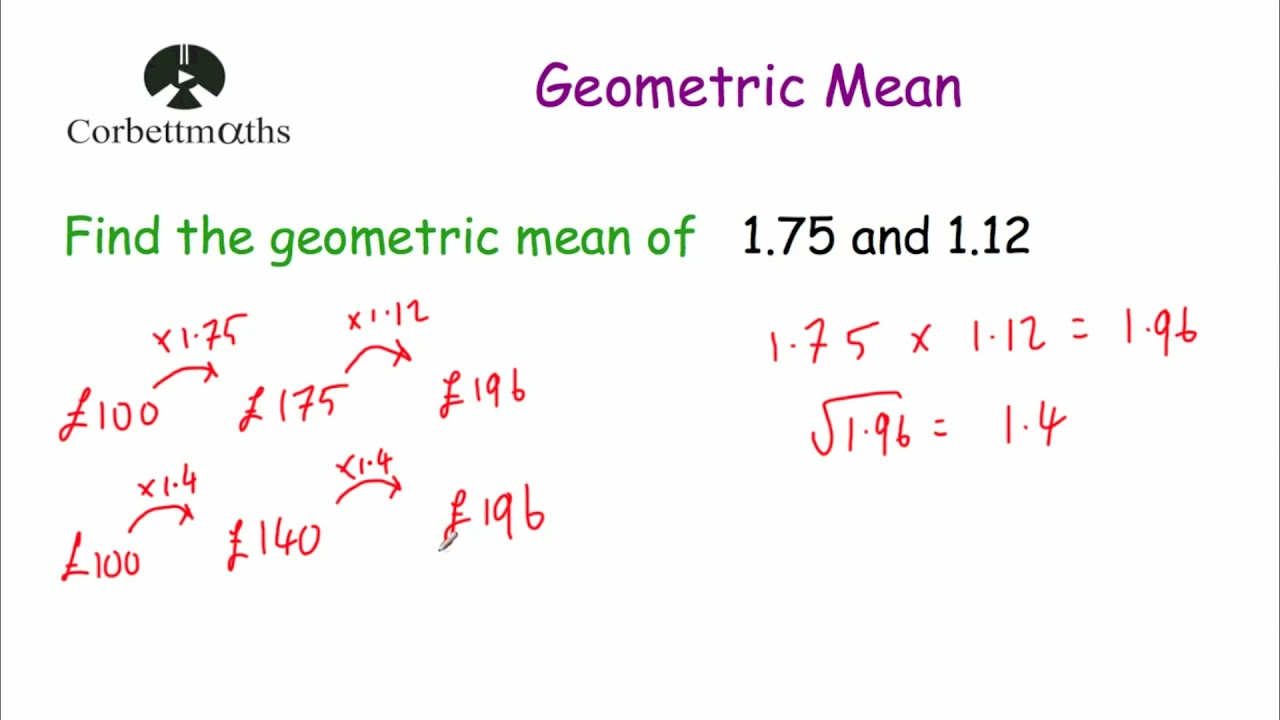
| Set of Numbers | Calculate Geometric Mean |
|---|---|
| 2, 4, 6, 8 | |
| 10, 20, 30, 40 | |
| 100, 200, 300, 400 | |
| 5, 10, 15, 20 | |
| 2, 5, 10, 15 |
Answers:
| Set of Numbers | Geometric Mean |
|---|---|
| 2, 4, 6, 8 | 4.32 |
| 10, 20, 30, 40 | 20.65 |
| 100, 200, 300, 400 | 230.94 |
| 5, 10, 15, 20 | 11.18 |
| 2, 5, 10, 15 | 5.71 |
Conclusion
In conclusion, the geometric mean is an important concept in statistics and mathematics that is used to calculate the average of a set of numbers that are meant to be multiplied together. By understanding the formula and examples, you can master the concept of geometric mean and apply it to real-world problems.
What is the main difference between arithmetic mean and geometric mean?
+The main difference between arithmetic mean and geometric mean is that arithmetic mean is used to calculate the average of a set of numbers that are meant to be added together, while geometric mean is used to calculate the average of a set of numbers that are meant to be multiplied together.
Can geometric mean be calculated for negative numbers?
+No, geometric mean cannot be calculated for negative numbers or zeros.
What is the formula for calculating geometric mean?
+The formula for calculating geometric mean is: GM = (n√(x1 × x2 × x3 ×… × xn))