Geometric Constructions Made Easy
Unlocking the Secrets of Geometric Constructions
Geometric constructions have been a cornerstone of mathematics and architecture for centuries. From the ancient Egyptians to modern-day engineers, the ability to create precise geometric shapes has been a fundamental aspect of building design, artistic expression, and problem-solving. However, for many students and professionals, geometric constructions can seem daunting and inaccessible. In this article, we will demystify the world of geometric constructions and provide a step-by-step guide on how to master this essential skill.
Understanding the Basics of Geometric Constructions
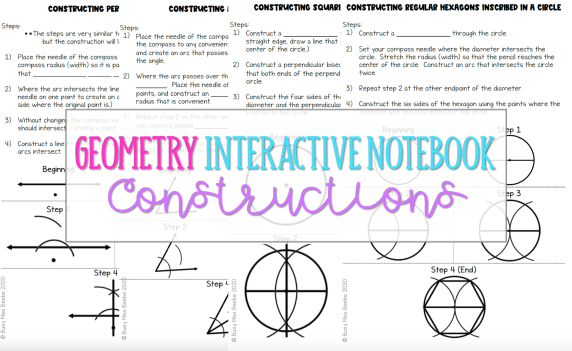
Before we dive into the world of geometric constructions, it’s essential to understand the basic concepts and tools involved. Geometric constructions involve creating geometric shapes using a compass and straightedge. The compass is used to draw circles and arcs, while the straightedge is used to draw lines and create angles.
Key Concepts:
- Points: A point is a location in space, represented by a set of coordinates.
- Lines: A line is a set of points that extend infinitely in two directions.
- Angles: An angle is formed by two lines or planes that intersect at a point.
- Circles: A circle is a set of points that are equidistant from a central point, called the center.
Basic Geometric Constructions
Now that we have a solid understanding of the basic concepts, let’s move on to some fundamental geometric constructions.
Construction 1: Drawing a Line Segment
To draw a line segment, place the point of the compass on one end of the segment and the pencil on the other. Open the compass to the desired length and draw a line.
📝 Note: Make sure to keep the compass steady and the pencil sharp to ensure accuracy.
Construction 2: Drawing a Circle
To draw a circle, place the point of the compass on the center of the circle and the pencil on the circumference. Open the compass to the desired radius and draw a circle.
Construction 3: Constructing an Angle
To construct an angle, place the point of the compass on the vertex of the angle and the pencil on one of the sides. Open the compass to the desired angle and draw an arc.
Advanced Geometric Constructions
Now that we have mastered the basic constructions, let’s move on to some more advanced techniques.
Construction 4: Constructing a Triangle
To construct a triangle, start by drawing two sides of the triangle using the line segment construction. Then, use the angle construction to create the third side.
Construction 5: Constructing a Square
To construct a square, start by drawing a line segment using the line segment construction. Then, use the angle construction to create a perpendicular line. Repeat this process to create the other two sides of the square.
Construction 6: Constructing a Circle with a Given Diameter
To construct a circle with a given diameter, start by drawing a line segment using the line segment construction. Then, use the circle construction to create a circle with the desired diameter.
Real-World Applications of Geometric Constructions
Geometric constructions have numerous real-world applications, from architecture to engineering.
- Building Design: Geometric constructions are used to create precise building designs, ensuring that structures are stable and aesthetically pleasing.
- Engineering: Geometric constructions are used to design and build complex systems, such as bridges and tunnels.
- Art: Geometric constructions are used to create intricate patterns and designs, used in various art forms.
Conclusion
Geometric constructions are a fundamental aspect of mathematics and architecture. By mastering the basic and advanced techniques outlined in this article, you can unlock a world of creative possibilities. Whether you’re a student, professional, or simply a enthusiast, geometric constructions can help you create precise and beautiful shapes, opening up new possibilities in design, art, and problem-solving.
What are the basic tools used in geometric constructions?
+The basic tools used in geometric constructions are a compass and a straightedge.
What is the difference between a line and a line segment?
+A line is a set of points that extend infinitely in two directions, while a line segment is a part of a line that has a definite length.
What are some real-world applications of geometric constructions?
+Geometric constructions have numerous real-world applications, including building design, engineering, and art.
Related Terms:
- Geometric constructions worksheet pdf answers
- Geometry Constructions Worksheet PDF
- Construction Worksheet pdf
- Geometry constructions worksheet answers
- Geometric constructions step-by-step PDF
- Geometric constructions PDF