Balancing Redox Equations Made Easy Worksheet Guide
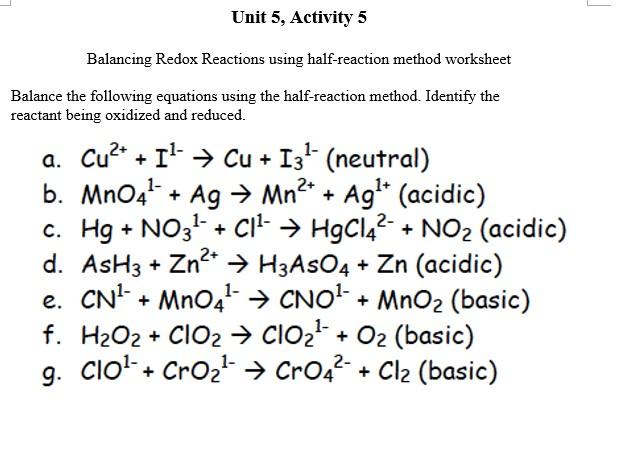
Understanding Redox Reactions
Redox reactions, also known as oxidation-reduction reactions, are a crucial concept in chemistry. These reactions involve the transfer of electrons from one species to another, resulting in a change in oxidation states. To balance redox equations, we need to account for the electrons lost and gained during the reaction.
What is a Redox Equation?
A redox equation is a chemical equation that represents a redox reaction. It typically consists of two half-equations: one for oxidation and one for reduction. The oxidation half-equation involves the loss of electrons, while the reduction half-equation involves the gain of electrons.
Why Balance Redox Equations?
Balancing redox equations is essential to ensure that the number of electrons lost equals the number of electrons gained. This balance is crucial for accurately representing the chemical reaction and predicting the products formed.
Step-by-Step Guide to Balancing Redox Equations
Balancing redox equations can be challenging, but with a step-by-step approach, it becomes more manageable. Here’s a worksheet guide to help you balance redox equations:
Step 1: Write the Unbalanced Equation
Start by writing the unbalanced redox equation. Make sure to include all reactants and products.
Step 2: Separate the Equation into Half-Equations
Separate the equation into two half-equations: one for oxidation and one for reduction.
Step 3: Balance the Half-Equations
Balance each half-equation separately, starting with the atoms and then the charges.
Step 4: Equalize the Electrons
Equalize the number of electrons lost in the oxidation half-equation with the number of electrons gained in the reduction half-equation.
Step 5: Combine the Half-Equations
Combine the balanced half-equations, making sure to cancel out any common terms.
Step 6: Check the Balance
Check the final equation to ensure that the number of atoms and charges are balanced.
📝 Note: When balancing redox equations, it's essential to remember that electrons are neither created nor destroyed, only transferred.
Example: Balancing a Redox Equation
Let’s consider the following unbalanced redox equation:
MnO4- + Fe2+ → Mn2+ + Fe3+
Step 1: Write the Unbalanced Equation
MnO4- + Fe2+ → Mn2+ + Fe3+
Step 2: Separate the Equation into Half-Equations
Oxidation: Fe2+ → Fe3+ Reduction: MnO4- → Mn2+
Step 3: Balance the Half-Equations
Oxidation: Fe2+ → Fe3+ + e- Reduction: MnO4- + 8H+ + 5e- → Mn2+ + 4H2O
Step 4: Equalize the Electrons
To equalize the electrons, we multiply the oxidation half-equation by 5 and the reduction half-equation by 1.
Oxidation: 5(Fe2+ → Fe3+ + e-) Reduction: MnO4- + 8H+ + 5e- → Mn2+ + 4H2O
Step 5: Combine the Half-Equations
Combine the balanced half-equations:
MnO4- + 8H+ + 5Fe2+ → Mn2+ + 4H2O + 5Fe3+
Step 6: Check the Balance
Check the final equation to ensure that the number of atoms and charges are balanced.

| Reactant | Product |
|---|---|
| MnO4- | Mn2+ |
| Fe2+ | Fe3+ |
| H+ | H2O |
| e- | e- |
📝 Note: Remember to check the balance of both atoms and charges.
Common Redox Reactions
Here are some common redox reactions:
- Combustion reactions: These reactions involve the oxidation of a substance in the presence of oxygen, resulting in the release of heat and light.
- Synthesis reactions: These reactions involve the combination of two or more substances to form a new compound.
- Decomposition reactions: These reactions involve the breakdown of a compound into simpler substances.
Tips and Tricks for Balancing Redox Equations
Here are some tips and tricks for balancing redox equations:
- Start with the most complex substance: Begin by balancing the most complex substance in the equation.
- Balance the atoms: Balance the atoms before balancing the charges.
- Use the half-equation method: The half-equation method is a useful technique for balancing redox equations.
- Check the balance: Always check the balance of both atoms and charges.
What is a redox reaction?
+A redox reaction is a chemical reaction that involves the transfer of electrons from one species to another, resulting in a change in oxidation states.
Why is it essential to balance redox equations?
+It's essential to balance redox equations to ensure that the number of electrons lost equals the number of electrons gained, accurately representing the chemical reaction and predicting the products formed.
What is the half-equation method?
+The half-equation method is a technique for balancing redox equations by separating the equation into two half-equations: one for oxidation and one for reduction.
To recap, balancing redox equations requires a step-by-step approach, starting with the unbalanced equation, separating it into half-equations, balancing the half-equations, equalizing the electrons, combining the half-equations, and checking the balance. By following these steps and using the half-equation method, you can master the art of balancing redox equations.



