5 Ways to Master Fraction Division
Understanding the Concept of Fraction Division
Fraction division is a complex mathematical operation that can be challenging for many students to grasp. However, with the right approach and practice, anyone can master it. In this article, we will explore five ways to master fraction division, including understanding the concept, visualizing the process, using real-world examples, practicing with different types of fractions, and applying the rules of fraction division.
1. Understanding the Concept of Fraction Division
To master fraction division, it’s essential to understand the concept behind it. Fraction division is the process of dividing one fraction by another. This can be represented by the following equation:
a/b ÷ c/d = (a/b) × (d/c)
Where a, b, c, and d are integers.
In simpler terms, dividing one fraction by another is equivalent to multiplying the first fraction by the reciprocal of the second fraction. The reciprocal of a fraction is obtained by swapping the numerator and denominator.
For example:
2⁄3 ÷ 3⁄4 = (2⁄3) × (4⁄3)
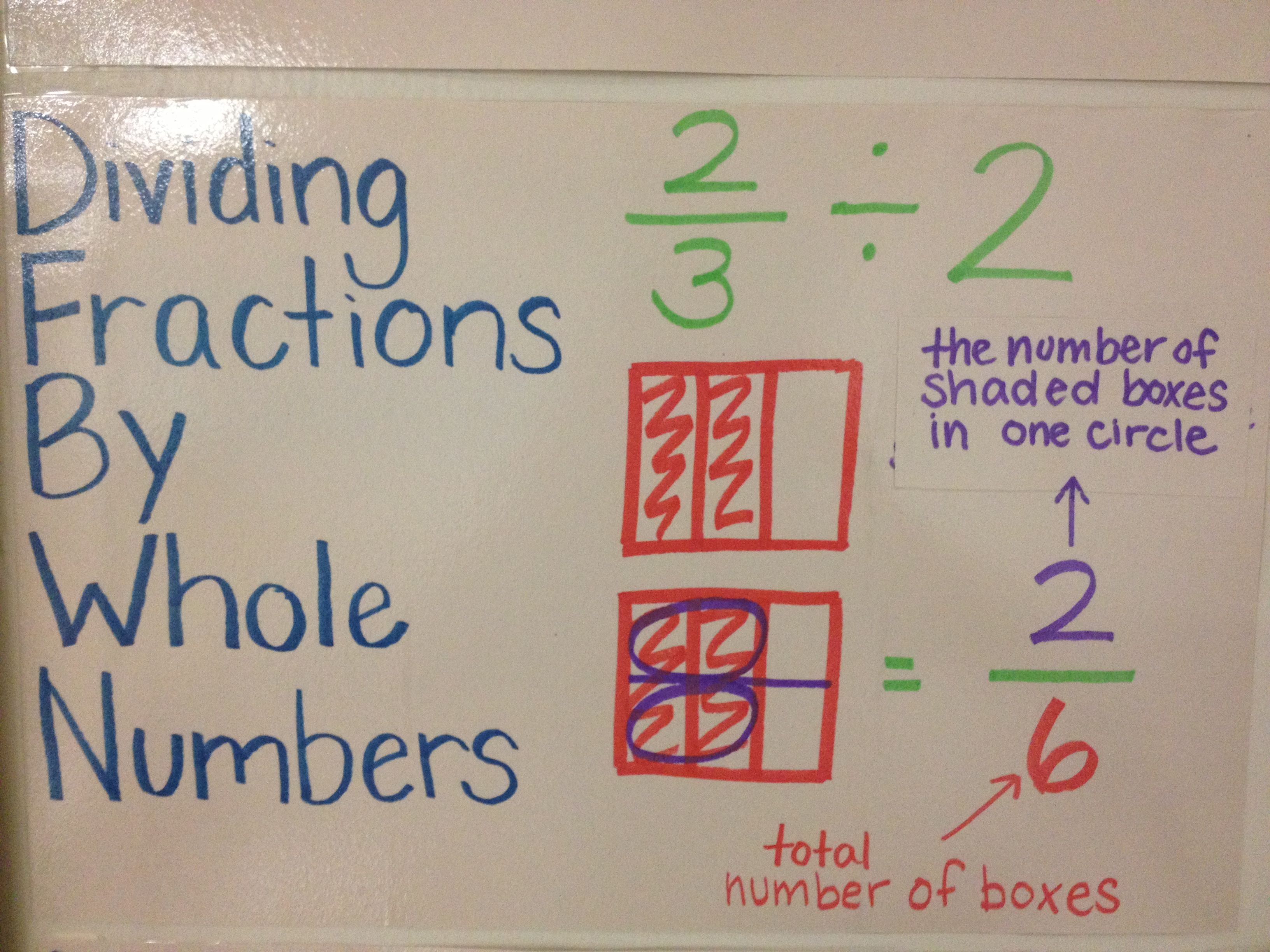
2. Visualizing the Process
Visualizing the process of fraction division can make it easier to understand and master. One way to visualize fraction division is to use a number line or a diagram.
Imagine a pizza that is divided into 12 equal slices. If you eat 2⁄3 of the pizza, and then your friend eats 3⁄4 of the remaining pizza, how much pizza is left?
By using a diagram or number line, you can visualize the process of fraction division and see how the numbers interact with each other.
📝 Note: Visualizing the process can help you understand the concept of fraction division, but it's essential to practice with different types of fractions to master the skill.
3. Using Real-World Examples
Using real-world examples can make fraction division more meaningful and interesting. Here are a few examples:
- If you have 2⁄3 of a tank of gas in your car, and you use 3⁄4 of it to drive to work, how much gas is left in the tank?
- If you have 3⁄4 of a cup of coffee, and you drink 2⁄3 of it, how much coffee is left in the cup?
By using real-world examples, you can see how fraction division applies to everyday situations and make it more relatable.
4. Practicing with Different Types of Fractions
To master fraction division, it’s essential to practice with different types of fractions. Here are a few examples:
- Simple fractions: 1⁄2 ÷ 3⁄4 = (1⁄2) × (4⁄3)
- Complex fractions: 2 3⁄4 ÷ 3 1⁄2 = (2 3⁄4) × (2 2⁄3)
- Mixed numbers: 2 1⁄2 ÷ 3⁄4 = (2 1⁄2) × (4⁄3)
By practicing with different types of fractions, you can become more comfortable with the process and apply it to various situations.
5. Applying the Rules of Fraction Division
To master fraction division, it’s essential to apply the rules of fraction division. Here are a few rules to keep in mind:
- Invert the second fraction: To divide one fraction by another, invert the second fraction and multiply.
- Multiply the numerators and denominators: Multiply the numerators and denominators separately.
- Simplify the result: Simplify the result to its simplest form.
By applying these rules, you can ensure that you are dividing fractions correctly and getting the right answers.
Key Points to Remember
- Fraction division is the process of dividing one fraction by another.
- To divide one fraction by another, invert the second fraction and multiply.
- Multiply the numerators and denominators separately.
- Simplify the result to its simplest form.
- Practice with different types of fractions to master the skill.
By following these five ways to master fraction division, you can become more confident and proficient in your ability to divide fractions.
What is fraction division?
+Fraction division is the process of dividing one fraction by another.
How do you divide one fraction by another?
+To divide one fraction by another, invert the second fraction and multiply.
What are some real-world examples of fraction division?
+Some real-world examples of fraction division include calculating the amount of gas left in a tank or the amount of coffee left in a cup.