5 Simple Steps to Master Fraction Adding and Subtracting

Understanding the Basics of Fractions
Adding and subtracting fractions is a fundamental concept in mathematics that can be a bit challenging for some students. However, with a clear understanding of the basics and a step-by-step approach, anyone can master fraction adding and subtracting. In this article, we will explore the five simple steps to master fraction adding and subtracting.
Step 1: Understand the Concept of Equivalent Fractions
Before adding or subtracting fractions, it’s essential to understand the concept of equivalent fractions. Equivalent fractions are fractions that have the same value but different numerators and denominators. For example, 1⁄2, 2⁄4, and 3⁄6 are all equivalent fractions.
To find equivalent fractions, you can multiply or divide both the numerator and denominator by the same number. This concept is crucial when adding or subtracting fractions with different denominators.
📝 Note: Make sure to find the equivalent fractions before adding or subtracting, as it will make the process much easier.
Step 2: Find the Least Common Multiple (LCM)
When adding or subtracting fractions with different denominators, you need to find the least common multiple (LCM) of the denominators. The LCM is the smallest number that both denominators can divide into evenly.
To find the LCM, you can list the multiples of each denominator and find the smallest number that appears in both lists. Alternatively, you can use the prime factorization method to find the LCM.
| Denominator | Multiples |
|---|---|
| 4 | 4, 8, 12, 16,… |
| 6 | 6, 12, 18, 24,… |
In this example, the LCM of 4 and 6 is 12.
Step 3: Convert Fractions to Have the Same Denominator
Once you have found the LCM, you can convert each fraction to have the same denominator. To do this, you need to multiply the numerator and denominator of each fraction by the same number.
For example, if you want to add 1⁄4 and 1⁄6, you need to convert both fractions to have a denominator of 12.
1⁄4 = (1 x 3)/(4 x 3) = 3⁄12 1⁄6 = (1 x 2)/(6 x 2) = 2⁄12
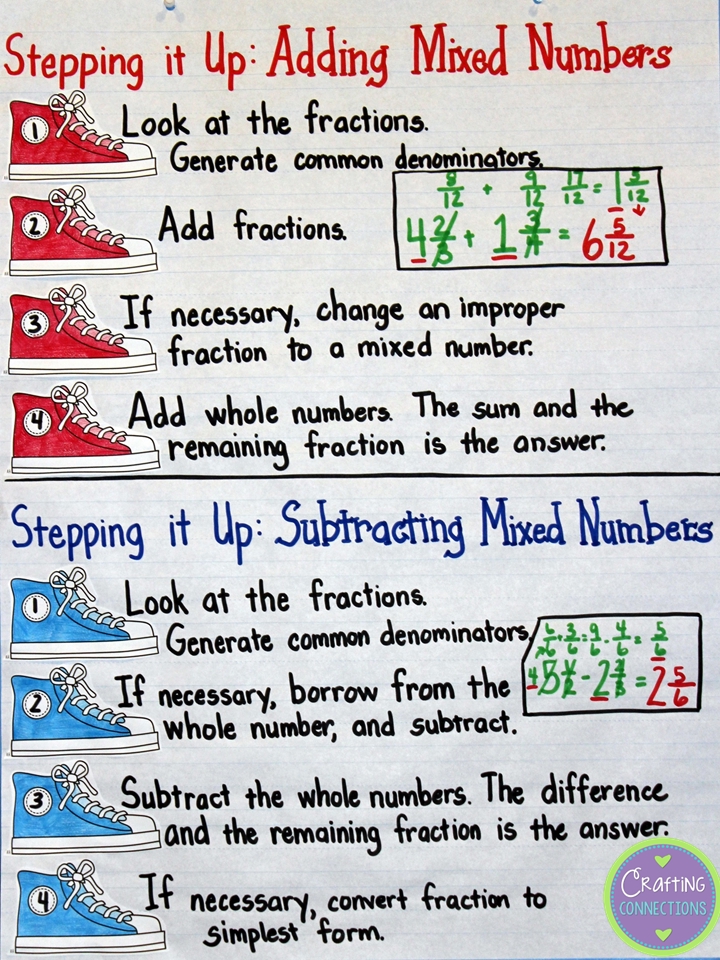
Step 4: Add or Subtract the Fractions
Now that both fractions have the same denominator, you can add or subtract them. To add fractions, simply add the numerators and keep the same denominator.
3⁄12 + 2⁄12 = (3 + 2)/12 = 5⁄12
To subtract fractions, subtract the numerators and keep the same denominator.
5⁄12 - 2⁄12 = (5 - 2)/12 = 3⁄12
Step 5: Simplify the Answer (If Necessary)
Finally, simplify the answer if necessary. To simplify a fraction, divide both the numerator and denominator by the greatest common divisor (GCD).
For example, if the answer is 6⁄12, you can simplify it by dividing both the numerator and denominator by 6.
6⁄12 = (6 ÷ 6)/(12 ÷ 6) = 1⁄2
By following these five simple steps, you can master fraction adding and subtracting. Remember to always find the equivalent fractions, find the LCM, convert fractions to have the same denominator, add or subtract the fractions, and simplify the answer if necessary.
With practice and patience, you will become proficient in adding and subtracting fractions in no time.
What is the difference between adding and subtracting fractions with like denominators and unlike denominators?
+When adding or subtracting fractions with like denominators, you can simply add or subtract the numerators and keep the same denominator. However, when adding or subtracting fractions with unlike denominators, you need to find the LCM and convert both fractions to have the same denominator before adding or subtracting.
How do I find the greatest common divisor (GCD) of two numbers?
+There are several ways to find the GCD of two numbers. One method is to list the factors of each number and find the greatest factor they have in common. Another method is to use the prime factorization method to find the GCD.
What is the importance of simplifying fractions?
+Simplifying fractions is important because it makes the fraction easier to work with and understand. Simplified fractions can also help to avoid errors when adding or subtracting fractions.