5 Essential Angle Relationship Rules to Master
Understanding Angle Relationships: A Comprehensive Guide
Angle relationships are a fundamental concept in geometry, and mastering them is essential for problem-solving and critical thinking. In this article, we will explore five essential angle relationship rules that every student should know.
The Basics of Angle Relationships
Before diving into the rules, let’s review the basics of angle relationships. Angles are formed when two rays share a common endpoint, called the vertex. The measure of an angle is the amount of rotation between the two rays, usually measured in degrees. Angle relationships involve the way angles interact with each other, and understanding these relationships is crucial for solving problems in geometry.
Rule 1: Complementary Angles
Complementary angles are two angles whose measures add up to 90 degrees. This means that if you have one angle that measures 30 degrees, its complementary angle will measure 60 degrees. Complementary angles are important because they can help you solve problems involving right triangles.
| Angle 1 | Angle 2 | Sum |
|---|---|---|
| 30° | 60° | 90° |
| 45° | 45° | 90° |
🔍 Note: Complementary angles do not have to be adjacent or share a common side.
Rule 2: Supplementary Angles
Supplementary angles are two angles whose measures add up to 180 degrees. This means that if you have one angle that measures 120 degrees, its supplementary angle will measure 60 degrees. Supplementary angles are important because they can help you solve problems involving linear pairs.
| Angle 1 | Angle 2 | Sum |
|---|---|---|
| 120° | 60° | 180° |
| 90° | 90° | 180° |
🔍 Note: Supplementary angles do not have to be adjacent or share a common side.
Rule 3: Alternate Interior Angles
Alternate interior angles are two angles that are on opposite sides of a transversal and inside the two lines. When two lines are cut by a transversal, the alternate interior angles are equal. This means that if one angle measures 30 degrees, the other angle will also measure 30 degrees.

🔍 Note: Alternate interior angles are equal only when the two lines are parallel.
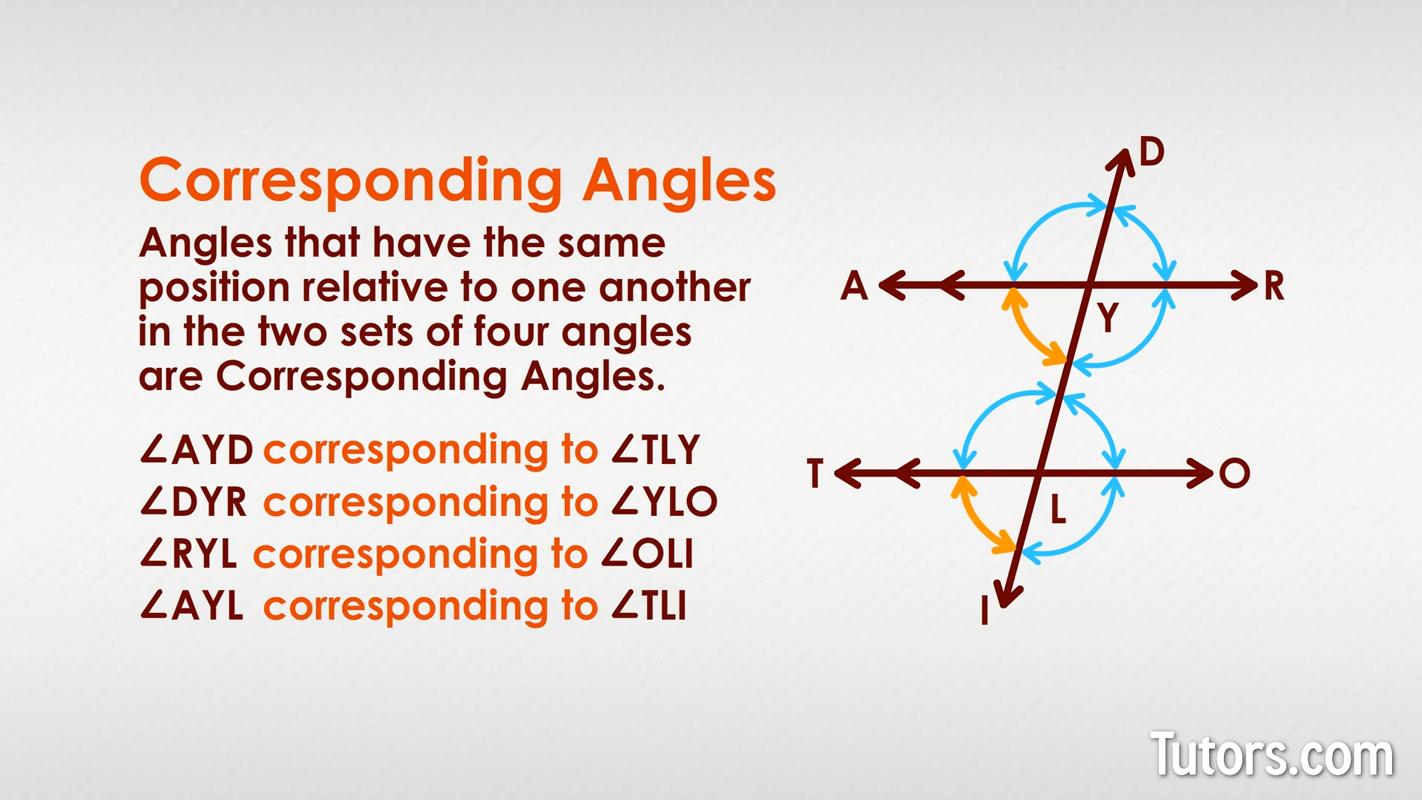
Rule 4: Corresponding Angles
Corresponding angles are two angles that are in the same relative position in two different angles. When two lines are cut by a transversal, the corresponding angles are equal. This means that if one angle measures 45 degrees, the corresponding angle will also measure 45 degrees.

🔍 Note: Corresponding angles are equal only when the two lines are parallel.
Rule 5: Vertical Angles
Vertical angles are two angles that are opposite each other when two lines intersect. Vertical angles are always equal, regardless of the size of the angles. This means that if one angle measures 60 degrees, the vertical angle will also measure 60 degrees.

🔍 Note: Vertical angles are equal because they are formed by two intersecting lines.
Mastering these five essential angle relationship rules will help you solve problems in geometry and develop your critical thinking skills. Remember to practice, practice, practice, and you will become a pro at angle relationships in no time!
In this section, we will summarize the key points discussed in the article.
When it comes to angle relationships, there are five essential rules to master: complementary angles, supplementary angles, alternate interior angles, corresponding angles, and vertical angles. Each of these rules has its own unique characteristics and applications, and understanding them is crucial for problem-solving in geometry.
By mastering these rules, you will be able to solve problems involving angle relationships with confidence and accuracy. Remember to practice regularly and apply these rules to real-world problems to reinforce your understanding.
What are complementary angles?
+Complementary angles are two angles whose measures add up to 90 degrees.
What are supplementary angles?
+Supplementary angles are two angles whose measures add up to 180 degrees.
What are alternate interior angles?
+Alternate interior angles are two angles that are on opposite sides of a transversal and inside the two lines.