10 Proportions Word Problems to Try Today
Mastering Proportions: 10 Word Problems to Try Today
Are you ready to put your proportional reasoning skills to the test? Proportions are an essential concept in mathematics, and word problems are an excellent way to apply your knowledge in real-world scenarios. In this article, we’ll explore 10 proportions word problems to help you improve your understanding and problem-solving skills.
Problem 1: Baking a Cake
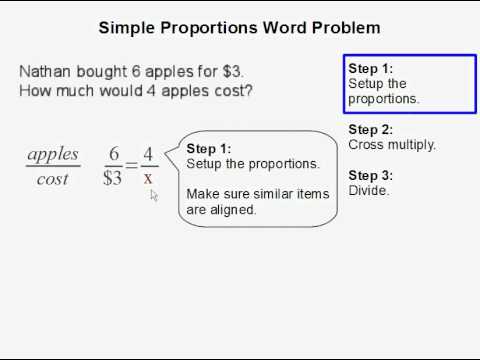
A recipe for baking a cake requires a ratio of 2:3 of flour to sugar. If you need 480 grams of flour, how many grams of sugar should you use?
🍰 Note: Make sure to use the ratio to set up a proportion and solve for the unknown value.
Solution: Let’s set up a proportion using the ratio 2:3 and the given value of flour (480 grams).
2⁄3 = 480/x
Cross-multiply and solve for x:
2x = 3 × 480 2x = 1440 x = 720
You should use 720 grams of sugar.
Problem 2: Planting a Garden
A gardener wants to plant a garden with a ratio of 5:2 of marigolds to zinnias. If she plants 150 marigolds, how many zinnias should she plant?
Solution: Use the ratio 5:2 to set up a proportion and solve for the unknown value.
5⁄2 = 150/x
Cross-multiply and solve for x:
5x = 2 × 150 5x = 300 x = 60
She should plant 60 zinnias.
Problem 3: Designing a Logo
A designer is creating a logo with a ratio of 3:4 of width to height. If the width is 300 pixels, what should the height be?
Solution: Set up a proportion using the ratio 3:4 and the given value of width (300 pixels).
3⁄4 = 300/x
Cross-multiply and solve for x:
3x = 4 × 300 3x = 1200 x = 400
The height should be 400 pixels.
Problem 4: Mixing Paint
A painter needs to mix a batch of paint with a ratio of 2:5 of red to blue. If she uses 240 grams of red paint, how many grams of blue paint should she use?
Solution: Use the ratio 2:5 to set up a proportion and solve for the unknown value.
2⁄5 = 240/x
Cross-multiply and solve for x:
2x = 5 × 240 2x = 1200 x = 600
She should use 600 grams of blue paint.
Problem 5: Building a Bookshelf
A carpenter is building a bookshelf with a ratio of 3:5 of width to height. If the width is 2.5 meters, what should the height be?
Solution: Set up a proportion using the ratio 3:5 and the given value of width (2.5 meters).
3⁄5 = 2.5/x
Cross-multiply and solve for x:
3x = 5 × 2.5 3x = 12.5 x = 4.17
The height should be approximately 4.17 meters.
Problem 6: Cooking a Recipe
A recipe for cooking a meal requires a ratio of 1:2 of rice to vegetables. If you need 300 grams of rice, how many grams of vegetables should you use?
Solution: Use the ratio 1:2 to set up a proportion and solve for the unknown value.
1⁄2 = 300/x
Cross-multiply and solve for x:
x = 2 × 300 x = 600
You should use 600 grams of vegetables.
Problem 7: Designing a Room
An interior designer is designing a room with a ratio of 4:5 of length to width. If the length is 8 meters, what should the width be?
Solution: Set up a proportion using the ratio 4:5 and the given value of length (8 meters).
4⁄5 = 8/x
Cross-multiply and solve for x:
4x = 5 × 8 4x = 40 x = 10
The width should be 10 meters.
Problem 8: Planting a Tree
A farmer wants to plant a tree with a ratio of 2:3 of height to width. If the height is 5 meters, what should the width be?
Solution: Use the ratio 2:3 to set up a proportion and solve for the unknown value.
2⁄3 = 5/x
Cross-multiply and solve for x:
2x = 3 × 5 2x = 15 x = 7.5
The width should be approximately 7.5 meters.
Problem 9: Mixing a Solution
A scientist needs to mix a solution with a ratio of 3:7 of acid to water. If she uses 210 grams of acid, how many grams of water should she use?
Solution: Set up a proportion using the ratio 3:7 and the given value of acid (210 grams).
3⁄7 = 210/x
Cross-multiply and solve for x:
3x = 7 × 210 3x = 1470 x = 490
She should use 490 grams of water.
Problem 10: Building a Fence
A contractor is building a fence with a ratio of 5:3 of length to width. If the length is 15 meters, what should the width be?
Solution: Use the ratio 5:3 to set up a proportion and solve for the unknown value.
5⁄3 = 15/x
Cross-multiply and solve for x:
5x = 3 × 15 5x = 45 x = 9
The width should be 9 meters.
To summarize, we’ve explored 10 proportions word problems to help you improve your understanding and problem-solving skills. Remember to use the ratio to set up a proportion and solve for the unknown value. Practice makes perfect, so try solving these problems on your own to become more confident in your abilities.
What is a proportion?
+A proportion is a statement that two ratios are equal. It can be represented as a:b = c:d, where a and b are the parts of the first ratio, and c and d are the parts of the second ratio.
How do I solve a proportion word problem?
+To solve a proportion word problem, use the ratio to set up a proportion and solve for the unknown value. Cross-multiply and solve for the variable.
What are some real-world applications of proportions?
+Proportions have numerous real-world applications, including cooking, design, architecture, and science. They help us solve problems and make decisions in various fields.