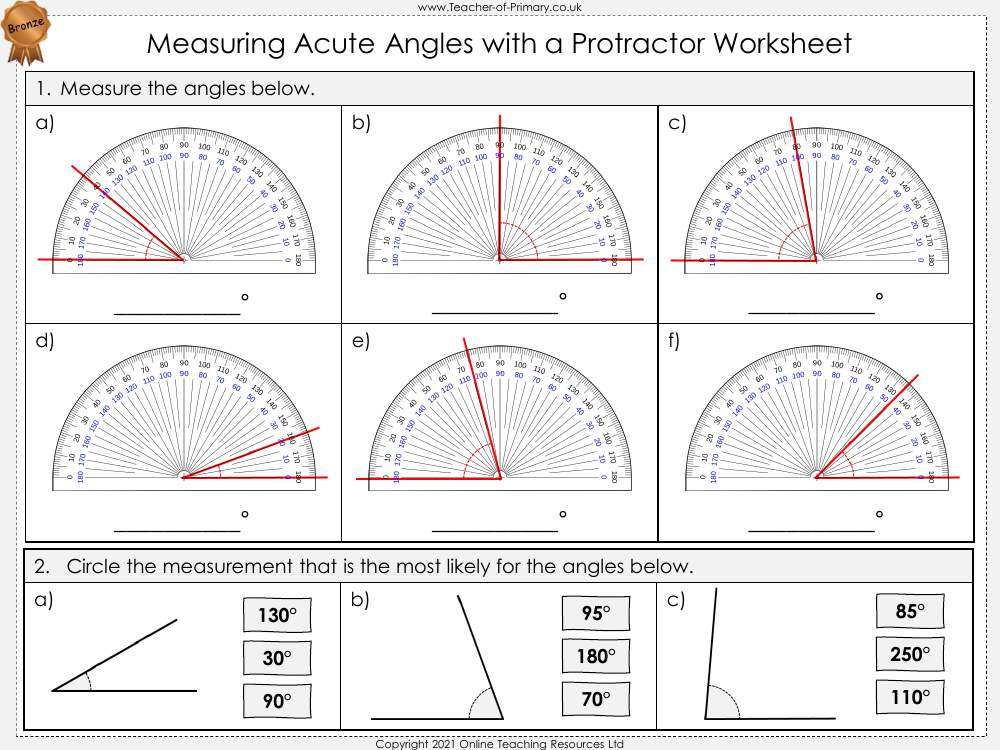
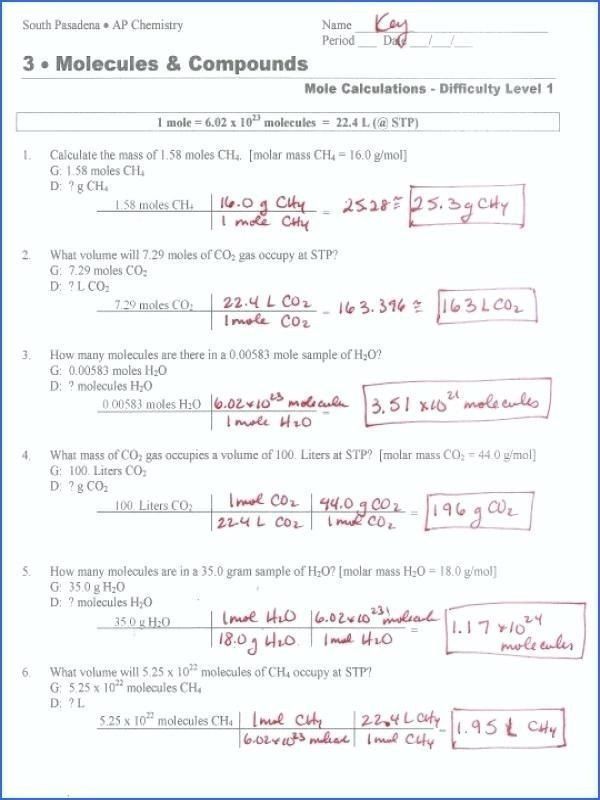
Factoring Quadratic Expressions Worksheet

Factoring Quadratic Expressions: A Comprehensive Guide
Quadratic expressions are a crucial part of algebra, and factoring them is an essential skill to master. In this post, we will delve into the world of quadratic expressions, explore different methods of factoring, and provide a detailed worksheet to help you practice.
What are Quadratic Expressions?
A quadratic expression is a polynomial expression of degree two, which means the highest power of the variable (usually x) is two. It can be written in the general form of ax^2 + bx + c, where a, b, and c are constants. For example, x^2 + 4x + 4 is a quadratic expression.
Why Factor Quadratic Expressions?
Factoring quadratic expressions is important because it helps us to:
- Solve quadratic equations by finding the roots or solutions
- Simplify complex expressions and equations
- Identify the x-intercepts and vertex of a parabola
- Write quadratic expressions in a more convenient form for graphing and analysis
Methods of Factoring Quadratic Expressions
There are several methods to factor quadratic expressions, including:
- Factoring by Greatest Common Factor (GCF): This method involves finding the greatest common factor of all the terms and factoring it out.
- Factoring by Grouping: This method involves grouping the terms into pairs and factoring out common factors from each pair.
- Factoring by Perfect Square Trinomials: This method involves recognizing perfect square trinomials and factoring them into the square of a binomial.
- Factoring by Using the Quadratic Formula: This method involves using the quadratic formula to find the roots of the equation and then factoring the expression.
Factoring Quadratic Expressions Worksheet
Here’s a worksheet to help you practice factoring quadratic expressions:
Section 1: Factoring by GCF
- x^2 + 5x + 6
- x^2 - 7x - 18
- x^2 + 2x - 6
- x^2 - 9x + 20
- x^2 + 10x + 24
Section 2: Factoring by Grouping
- x^2 + 3x + 2x + 6
- x^2 - 2x - 5x + 10
- x^2 + 4x + 3x + 12
- x^2 - 3x - 2x + 6
- x^2 + 2x + 5x + 10
Section 3: Factoring by Perfect Square Trinomials
- x^2 + 6x + 9
- x^2 - 8x + 16
- x^2 + 4x + 4
- x^2 - 10x + 25
- x^2 + 12x + 36
Section 4: Factoring by Using the Quadratic Formula
- x^2 + 2x - 3
- x^2 - 5x - 6
- x^2 + 3x - 4
- x^2 - 2x - 5
- x^2 + 4x - 3
📝 Note: Make sure to check your answers and try to factor the expressions on your own before looking at the solutions.
| Section | Problem | Solution |
|---|---|---|
| Section 1 | 1. x^2 + 5x + 6 | (x + 3)(x + 2) |
| Section 1 | 2. x^2 - 7x - 18 | (x - 9)(x + 2) |
| Section 1 | 3. x^2 + 2x - 6 | (x + 3)(x - 2) |
| Section 1 | 4. x^2 - 9x + 20 | (x - 5)(x - 4) |
| Section 1 | 5. x^2 + 10x + 24 | (x + 6)(x + 4) |
📝 Note: The solutions to the remaining problems can be found in a similar manner.
In conclusion, factoring quadratic expressions is an essential skill in algebra that requires practice and patience. By mastering different methods of factoring, you can simplify complex expressions and equations, solve quadratic equations, and identify the x-intercepts and vertex of a parabola.
What is the difference between factoring and solving a quadratic equation?
+Factoring a quadratic expression involves expressing it as a product of two binomials, while solving a quadratic equation involves finding the values of the variable that satisfy the equation.
Can all quadratic expressions be factored?
+No, not all quadratic expressions can be factored. Some quadratic expressions may have complex roots or may not be able to be factored using simple methods.
How can I check my answers when factoring quadratic expressions?
+You can check your answers by multiplying the factored expression and comparing it to the original expression. If the two expressions are equal, then your factoring is correct.
Related Terms:
- Factoring expressions worksheet
- Factoring quadratic expressions calculator
- Simplifying Quadratic Expressions Worksheet
- Factoring quadratic equations
- Factoring Worksheet with Answers pdf
- Quadratic equation Factoring Worksheet