Division Properties Of Exponents Worksheet
Understanding Division Properties of Exponents
Exponents are shorthand for repeated multiplication of the same number. When we have a division problem involving exponents, it can be solved using certain rules. These rules help simplify the expression and make calculations easier.
Division Property of Exponents
The division property of exponents states that when dividing two exponential expressions with the same base, we subtract the exponents. The formula for this is:
a^m / a^n = a^(m - n)
Where ‘a’ is the base and ’m’ and ‘n’ are the exponents.
Example 1: Simplifying with the Same Base
Let’s use the division property to simplify the following expression:
x^6 / x^3
Applying the formula:
x^6 / x^3 = x^(6 - 3) = x^3
As you can see, subtracting the exponents gives us the simplified expression.
Example 2: Different Bases
What if the bases are different? Let’s look at the expression:
2^4 / 3^2
Since the bases are not the same, we cannot apply the division property directly. Instead, we simplify each term separately:
2^4 = 16 3^2 = 9
Now we can divide:
16 / 9 = 1.78 (rounded to two decimal places)
Example 3: Complex Expression
Here’s a more complex expression involving multiple terms and different bases:
(x^3 * y^2) / (x^2 * y^3)
We can simplify this expression using the division property and combining like terms:
x^3 / x^2 = x^(3 - 2) = x y^2 / y^3 = y^(2 - 3) = y^(-1) = 1/y
Putting it all together:
x * y^(-1) = x / y
Practice Time!
Use the division property of exponents to simplify the following expressions:
• x^8 / x^4 =? • 2^6 / 2^2 =? • (3^4 * x^2) / (3^2 * x^3) =?
📝 Note: When simplifying expressions with exponents, it's essential to apply the rules carefully to avoid errors.
Solving Exponent Division Problems
When solving exponent division problems, we follow these steps:
- Identify the bases and exponents.
- Check if the bases are the same. If they are, apply the division property by subtracting the exponents.
- If the bases are different, simplify each term separately.
- Combine like terms (if applicable).
- Write the final simplified expression.
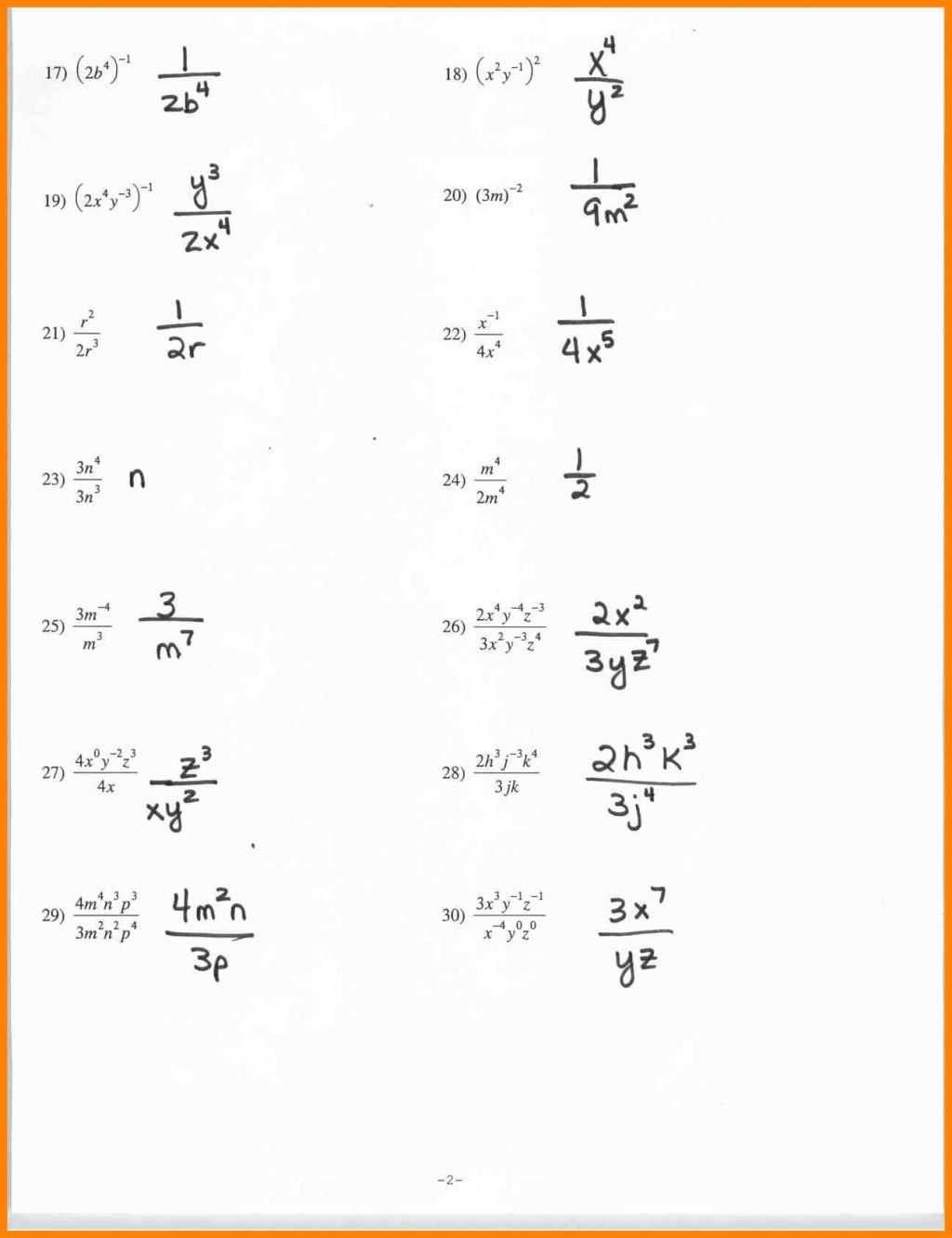
Division Properties of Exponents Worksheet
Practice simplifying exponent expressions with the following worksheet:

| Expression | Simplified Expression |
|---|---|
| x^9 / x^5 | |
| 4^3 / 2^2 | |
| (2^5 * x^3) / (2^3 * x^2) | |
| y^8 / y^4 | |
| 3^6 / 3^2 | |
| (x^2 * y^4) / (x^4 * y^2) |
📝 Note: When completing the worksheet, make sure to apply the division property carefully and simplify expressions step by step.
What is the division property of exponents?
+The division property of exponents states that when dividing two exponential expressions with the same base, we subtract the exponents.
Can I apply the division property to expressions with different bases?
+No, the division property only applies to expressions with the same base. If the bases are different, simplify each term separately.
How do I simplify complex expressions with exponents?
+When simplifying complex expressions, apply the division property and combine like terms carefully. Make sure to simplify each term step by step.
Recap and Key Takeaways
In conclusion, the division property of exponents is a powerful tool for simplifying expressions involving exponents. By applying the property correctly, you can simplify expressions with the same base and make calculations easier. Remember to practice regularly to become more confident in your ability to simplify exponent expressions.
Related Terms:
- Division properties of exponents calculator
- Properties of Exponents Worksheet PDF
- Power Rule exponents worksheet
- Properties of exponents practice