Fraction to Decimal Conversion Made Easy
Understanding Fractions and Decimals
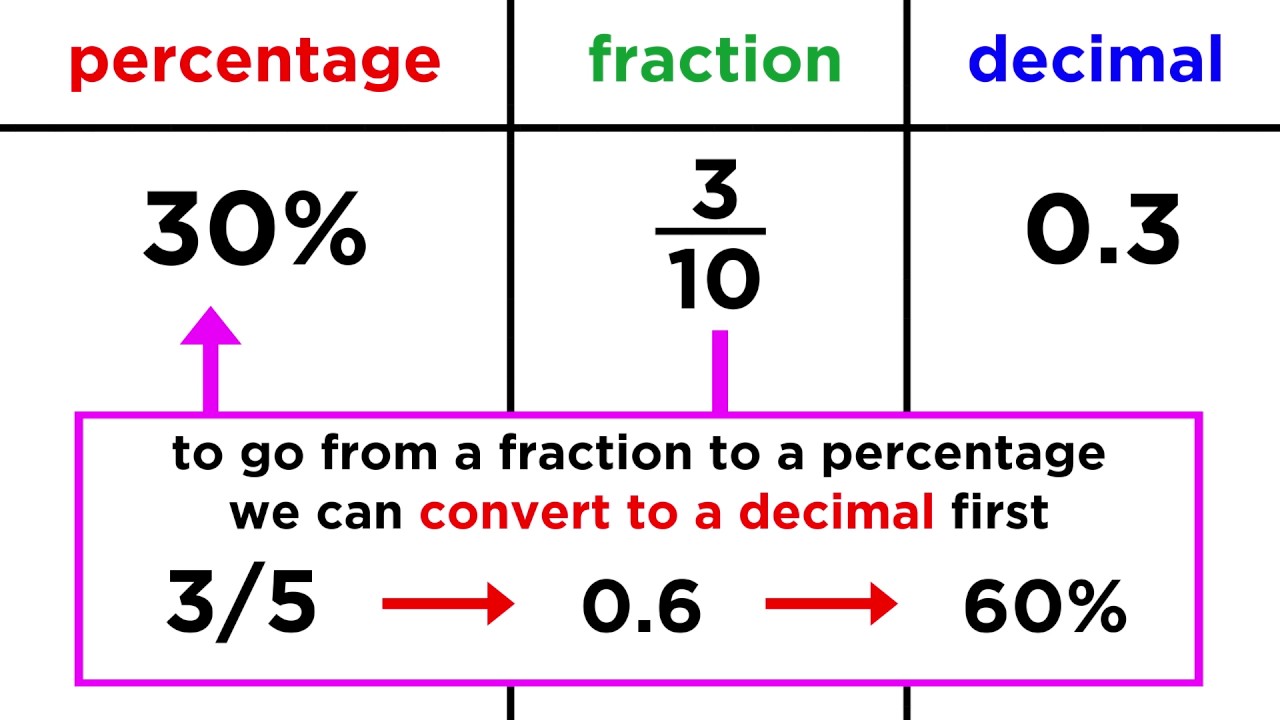
Fractions and decimals are two ways to represent parts of a whole in mathematics. A fraction is a way to express a part of a whole as a ratio of two numbers, with the top number (numerator) telling us how many equal parts we have, and the bottom number (denominator) telling us how many parts the whole is divided into. On the other hand, a decimal is a way to express a part of a whole as a number with a point (or decimal point) separating the whole part from the fractional part.
Why Convert Fractions to Decimals?
Converting fractions to decimals is an essential skill in mathematics, as it allows us to perform arithmetic operations, compare quantities, and solve problems in a more straightforward way. Decimals are often more intuitive and easier to work with than fractions, especially when dealing with real-world applications such as finance, science, and engineering.
Methods for Converting Fractions to Decimals
There are several methods to convert fractions to decimals, each with its own advantages and disadvantages. Here are some of the most common methods:
- Division Method: This is the most straightforward method, where you divide the numerator by the denominator to get the decimal equivalent. For example, to convert 3⁄4 to a decimal, you divide 3 by 4, which gives you 0.75.
- Equivalent Fractions Method: This method involves finding an equivalent fraction with a denominator that is a power of 10 (10, 100, 1000, etc.). For example, to convert 3⁄4 to a decimal, you can multiply both the numerator and denominator by 25 to get 75⁄100, which is equal to 0.75.
- Long Division Method: This method involves using long division to divide the numerator by the denominator. This method is more time-consuming but can be useful for converting fractions with large denominators.
📝 Note: When converting fractions to decimals, it's essential to check if the fraction is in its simplest form to avoid errors.
Examples and Practice Problems
Here are some examples and practice problems to help you master the art of converting fractions to decimals:
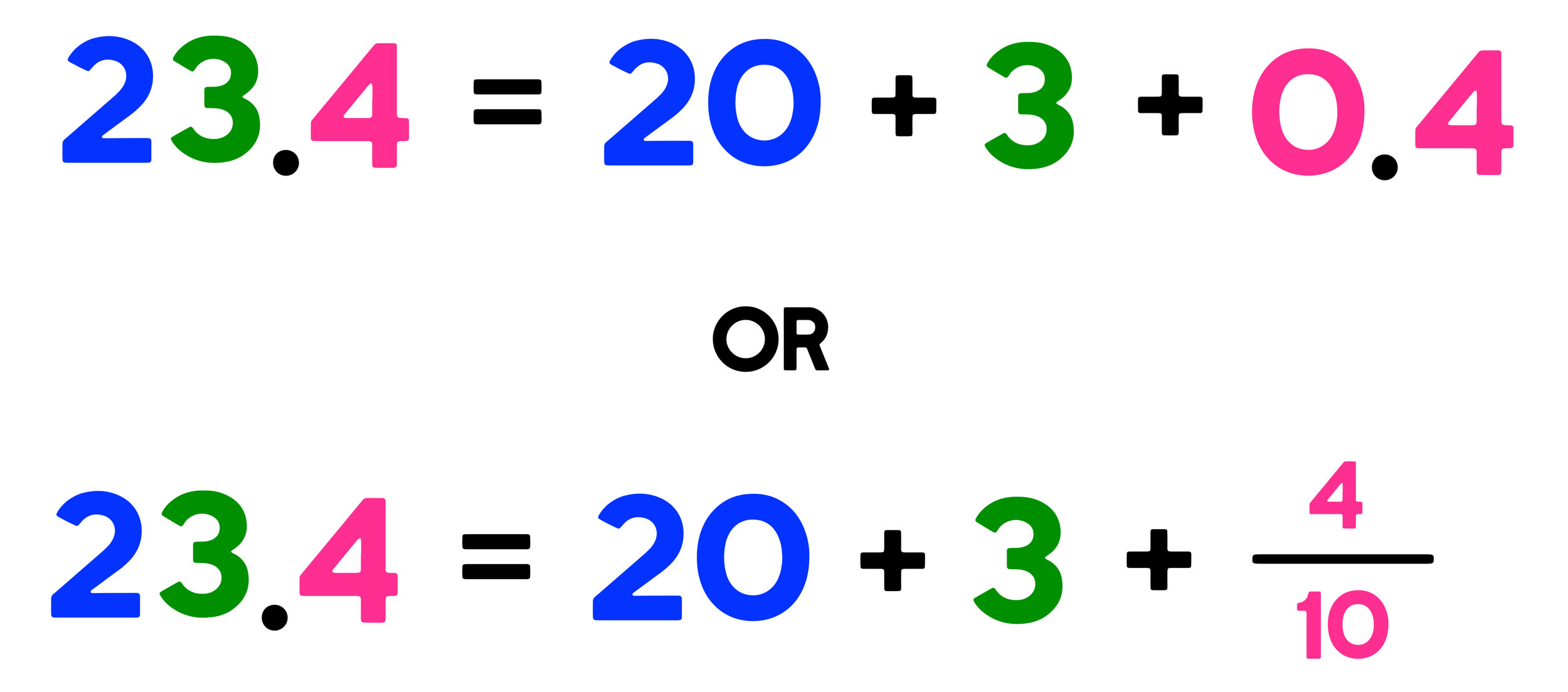
| Fraction | Decimal Equivalent |
|---|---|
| 1⁄2 | 0.5 |
| 3⁄4 | 0.75 |
| 2⁄3 | 0.67 |
| 5⁄6 | 0.83 |
Practice Problems:
- Convert 2⁄5 to a decimal using the division method.
- Convert 3⁄8 to a decimal using the equivalent fractions method.
- Convert 7⁄9 to a decimal using the long division method.
Real-World Applications
Converting fractions to decimals has numerous real-world applications, including:
- Finance: Calculating interest rates, investment returns, and currency exchange rates.
- Science: Measuring quantities such as temperature, mass, and volume.
- Engineering: Designing and building structures, machines, and systems.
Common Mistakes to Avoid
When converting fractions to decimals, here are some common mistakes to avoid:
- Rounding errors: Make sure to round decimals correctly to avoid errors.
- Equivalent fractions: Ensure that the fraction is in its simplest form before converting to a decimal.
- Long division: Be careful when using long division to avoid errors in calculation.
Conclusion
Converting fractions to decimals is an essential skill in mathematics, and with practice and patience, you can master it. By understanding the different methods and avoiding common mistakes, you can become proficient in converting fractions to decimals and apply it to various real-world applications.
What is the easiest method to convert fractions to decimals?
+The easiest method to convert fractions to decimals is the division method, where you divide the numerator by the denominator.
What is the importance of converting fractions to decimals?
+Converting fractions to decimals is essential in mathematics, as it allows us to perform arithmetic operations, compare quantities, and solve problems in a more straightforward way.
What are some common mistakes to avoid when converting fractions to decimals?
+Common mistakes to avoid when converting fractions to decimals include rounding errors, equivalent fractions, and long division errors.