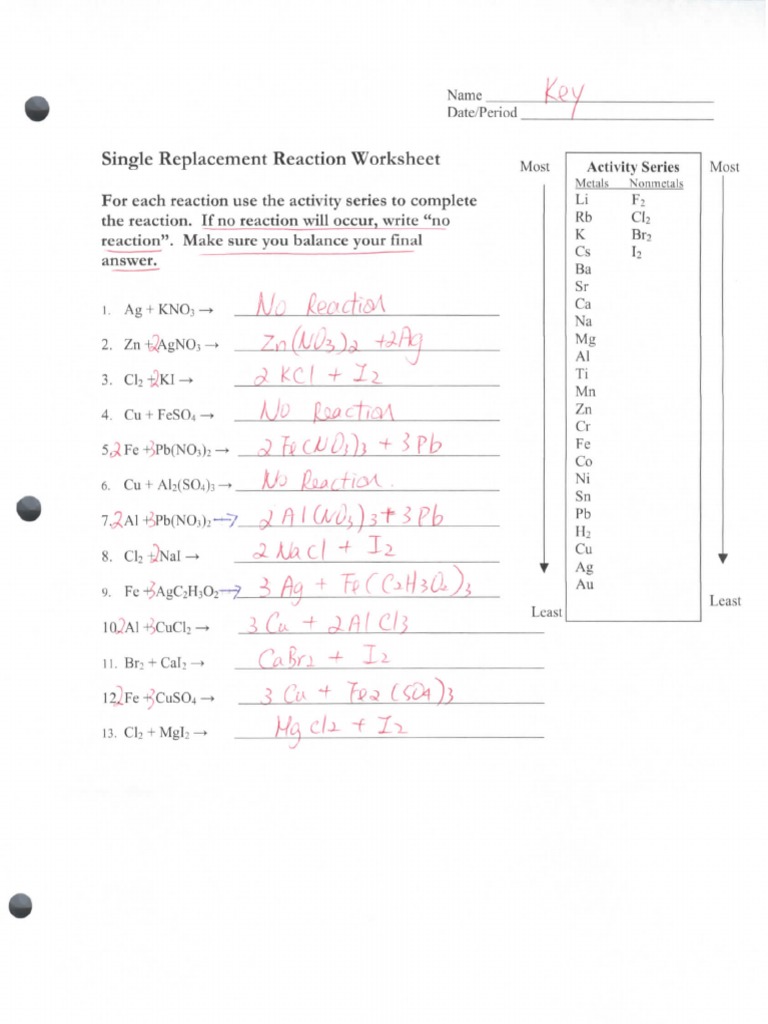
5 Easy Ways to Divide Fractions

Dividing fractions can seem daunting at first, but with a few simple steps, you can master this mathematical operation. In this article, we will explore five easy ways to divide fractions, along with examples and explanations to help you understand the concepts.

Understanding Fractions

Before we dive into dividing fractions, let’s quickly review what fractions are. A fraction is a way to express a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). For example, in the fraction ³⁄₄, the numerator is 3, and the denominator is 4.

Method 1: Inverting and Multiplying

One of the easiest ways to divide fractions is to invert the second fraction (i.e., flip the numerator and denominator) and then multiply.

Step-by-Step Instructions:

1. Write the division problem with the fractions.
2. Invert the second fraction by swapping the numerator and denominator.
3. Multiply the two fractions.

Example:

Divide ¹⁄₂ by ³⁄₄

1. Write the division problem: ¹⁄₂ ÷ ³⁄₄
2. Invert the second fraction: ³⁄₄ becomes ⁴⁄₃
3. Multiply: ¹⁄₂ × ⁴⁄₃ = ⁴⁄₆

Simplifying the Answer

The result, ⁴⁄₆, can be simplified by dividing both the numerator and denominator by 2. This gives us the final answer: ²⁄₃.

Method 2: Using the Reciprocal

Another way to divide fractions is to use the reciprocal of the divisor (the second fraction).

Step-by-Step Instructions:

1. Write the division problem with the fractions.
2. Find the reciprocal of the divisor (i.e., flip the numerator and denominator).
3. Multiply the dividend (the first fraction) by the reciprocal.

Example:

Divide ²⁄₃ by ³⁄₄

1. Write the division problem: ²⁄₃ ÷ ³⁄₄
2. Find the reciprocal of the divisor: ³⁄₄ becomes ⁴⁄₃
3. Multiply: ²⁄₃ × ⁴⁄₃ = ⁸⁄₉

Method 3: Using a Diagram

For a more visual approach, you can use a diagram to divide fractions.

Step-by-Step Instructions:

1. Draw a diagram representing the dividend (the first fraction).
2. Divide the diagram into equal parts to represent the divisor (the second fraction).
3. Count the number of parts that represent the dividend.

Example:

Divide ¹⁄₂ by ¹⁄₄

1. Draw a diagram representing ¹⁄₂ (a circle divided into 2 equal parts).
2. Divide the diagram into 4 equal parts to represent ¹⁄₄.
3. Count the number of parts that represent ¹⁄₂: 2 parts out of 4.

Method 4: Using Equivalent Fractions

You can also divide fractions by finding equivalent fractions.

Step-by-Step Instructions:

1. Write the division problem with the fractions.
2. Find an equivalent fraction for the divisor (the second fraction) with a denominator that is a multiple of the dividend’s numerator.
3. Multiply the dividend by the equivalent fraction.

Example:

Divide ³⁄₄ by ²⁄₃

1. Write the division problem: ³⁄₄ ÷ ²⁄₃
2. Find an equivalent fraction for ²⁄₃ with a denominator that is a multiple of 3: ⁴⁄₆
3. Multiply: ³⁄₄ × ⁴⁄₆ = ¹²⁄₂₄

Method 5: Using Real-World Applications

Finally, you can use real-world applications to divide fractions.

Step-by-Step Instructions:

1. Write the division problem with the fractions.
2. Think of a real-world scenario that represents the division problem.
3. Solve the problem using the scenario.

Example:

Divide ¹⁄₂ by ¹⁄₄

1. Write the division problem: ¹⁄₂ ÷ ¹⁄₄
2. Think of a real-world scenario: baking a cake that requires ¹⁄₂ cup of sugar, and you have a ¹⁄₄ cup measuring cup.
3. Solve the problem: to get ¹⁄₂ cup, you need to fill the ¹⁄₄ cup measuring cup 2 times.

Important Notes:

• When dividing fractions, it’s essential to simplify the answer to its lowest terms.
• Dividing fractions is the inverse operation of multiplying fractions.
• Practice dividing fractions regularly to become more comfortable with the concept.

Common Mistakes to Avoid:

• Inverting the wrong fraction
• Forgetting to multiply after inverting
• Not simplifying the answer

By following these five easy methods, you’ll become more confident in dividing fractions. Remember to practice regularly and use real-world applications to reinforce your understanding.

Without further ado, here is the conclusion:

Dividing fractions may seem intimidating at first, but with these five easy methods, you can master this mathematical operation. Whether you’re using the invert-and-multiply method, the reciprocal method, or one of the other approaches, you’ll be able to divide fractions with ease. Just remember to simplify your answers and practice regularly to become more comfortable with the concept.