Divide Fractions Made Easy with This Practice Worksheet
Understanding Fractions and Division
Fractions are a fundamental concept in mathematics, and dividing them can be a challenging task for many students. However, with practice and the right approach, dividing fractions can become a straightforward process. In this article, we will explore the basics of fractions, how to divide them, and provide a practice worksheet to help you master this skill.
What are Fractions?
A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.
How to Divide Fractions
To divide fractions, we need to follow a simple rule:
Invert the second fraction and multiply
In other words, to divide a fraction by another fraction, we need to flip the second fraction (i.e., swap the numerator and denominator) and then multiply the two fractions.
For example, to divide 1⁄2 by 3⁄4, we would:
- Invert the second fraction: 3⁄4 becomes 4⁄3
- Multiply the fractions: (1⁄2) × (4⁄3) = 4⁄6
Practice Worksheet: Dividing Fractions
Now it’s time to practice dividing fractions! Here’s a worksheet with 10 exercises to help you master this skill.
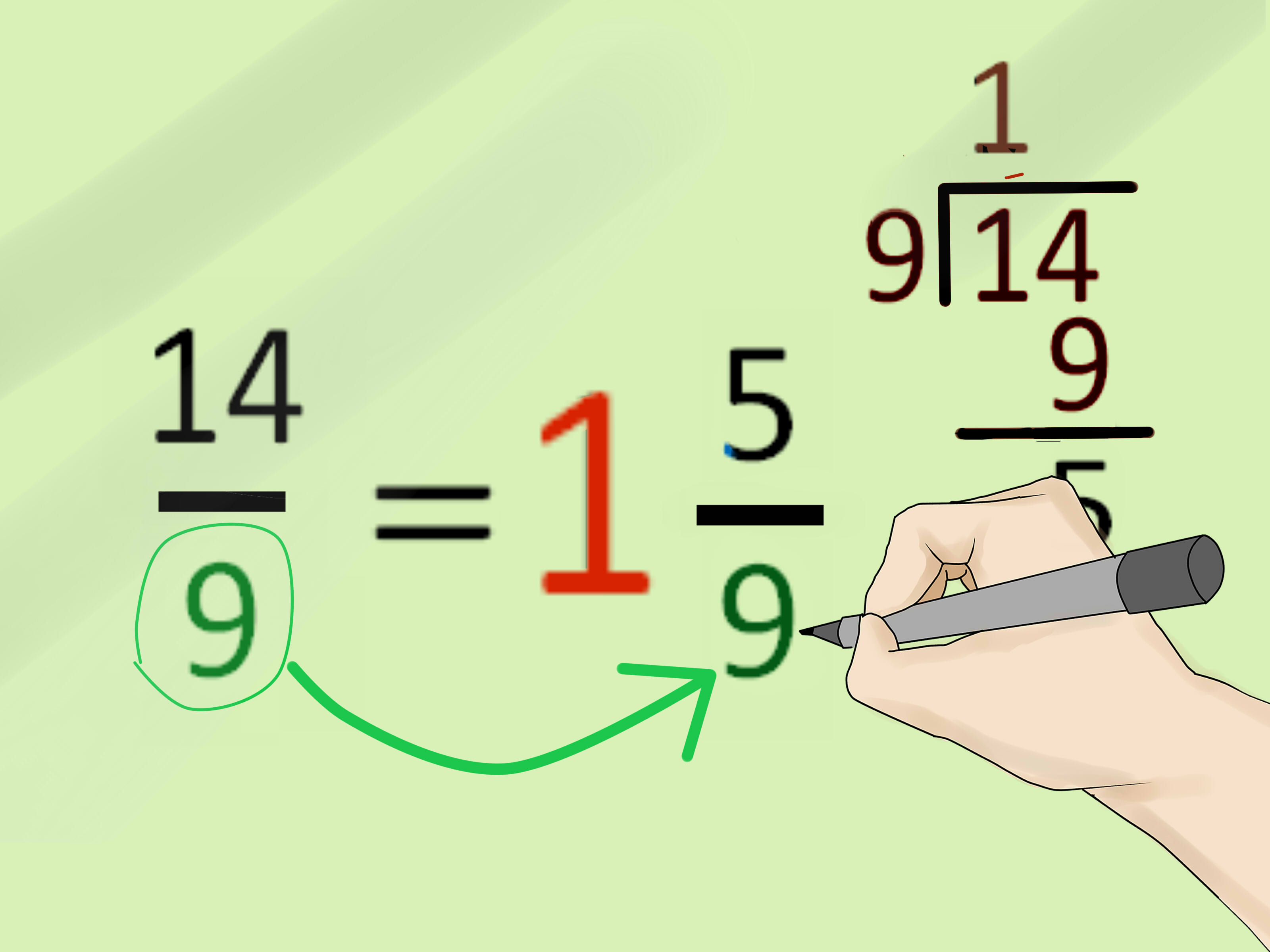
| Exercise | Problem | Solution |
|---|---|---|
| 1 | 1⁄2 ÷ 3⁄4 | |
| 2 | 2⁄3 ÷ 5⁄6 | |
| 3 | 3⁄4 ÷ 2⁄5 | |
| 4 | 1⁄3 ÷ 2⁄7 | |
| 5 | 4⁄5 ÷ 3⁄4 | |
| 6 | 2⁄5 ÷ 3⁄4 | |
| 7 | 1⁄4 ÷ 2⁄3 | |
| 8 | 3⁄5 ÷ 4⁄5 | |
| 9 | 2⁄3 ÷ 5⁄6 | |
| 10 | 1⁄2 ÷ 3⁄4 |
To solve each problem, simply invert the second fraction and multiply.
🤔 Note: Remember to simplify your answers by dividing both the numerator and denominator by the greatest common divisor (GCD), if possible.
Tips and Tricks for Dividing Fractions
Here are some additional tips to help you become a pro at dividing fractions:
- Always invert the second fraction before multiplying.
- Simplify your answers by dividing both the numerator and denominator by the GCD, if possible.
- Use visual aids, such as diagrams or number lines, to help you understand the concept of dividing fractions.
- Practice, practice, practice! The more you practice, the more comfortable you’ll become with dividing fractions.
Real-World Applications of Dividing Fractions
Dividing fractions is an essential skill in many real-world applications, including:
- Cooking and recipe scaling
- Measuring ingredients and mixing substances
- Financial calculations, such as interest rates and investments
- Science and engineering, where fractions are used to represent proportions and ratios
By mastering the skill of dividing fractions, you’ll be able to tackle a wide range of problems with confidence and accuracy.
What is the rule for dividing fractions?
+To divide fractions, invert the second fraction (i.e., swap the numerator and denominator) and then multiply the two fractions.
Why do I need to simplify my answers?
+Simplifying your answers helps to ensure that your solution is in its simplest form, making it easier to understand and work with.
What are some real-world applications of dividing fractions?
+Dividing fractions is used in cooking, finance, science, and engineering, among other fields, to represent proportions and ratios.
In conclusion, dividing fractions is a straightforward process that requires inverting the second fraction and multiplying. With practice and the right approach, you can master this skill and apply it to a wide range of real-world problems. Remember to simplify your answers and use visual aids to help you understand the concept of dividing fractions. Happy practicing!