Distributive Property of Multiplication Made Easy

Understanding the Distributive Property of Multiplication

The distributive property of multiplication is a fundamental concept in mathematics that can be a bit tricky to grasp at first, but with practice and the right explanations, it can become second nature. In this article, we will delve into the world of distributive property, explore its definition, and provide examples and exercises to help you master this essential math concept.

What is the Distributive Property of Multiplication?

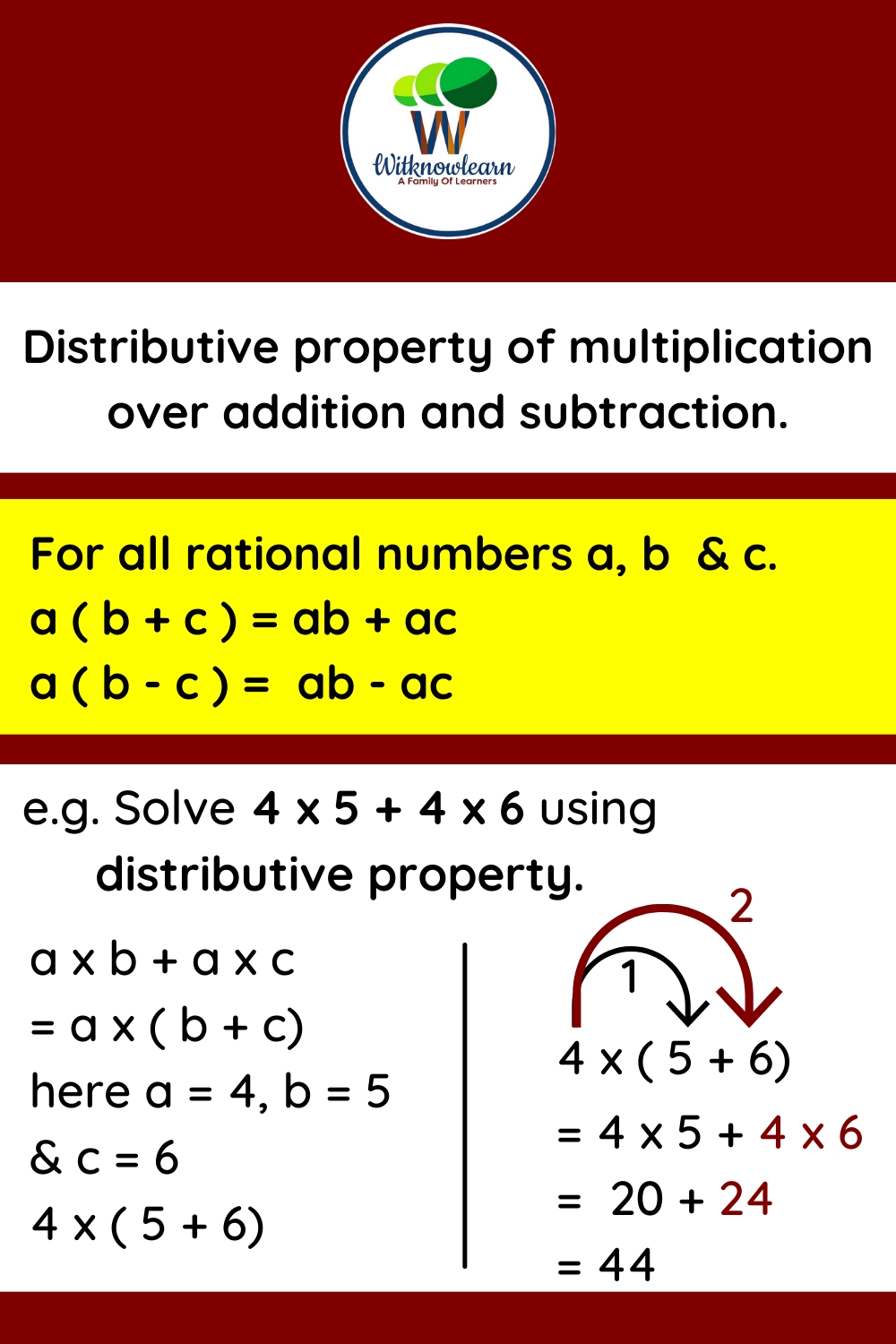
The distributive property of multiplication states that for any numbers a, b, and c:

a(b + c) = ab + ac

In simpler terms, when you multiply a number (a) by the sum of two other numbers (b + c), it is the same as multiplying the number (a) by each of the two numbers (b and c) separately and then adding the results.

Why is the Distributive Property Important?

The distributive property is a powerful tool in mathematics, and it has numerous applications in various fields, including algebra, geometry, and calculus. It helps simplify complex expressions, making it easier to solve equations and problems. By applying the distributive property, you can:

• Simplify expressions with multiple terms
• Expand and factorize expressions
• Solve equations and inequalities
• Graph functions and relations

Examples of the Distributive Property

Let’s look at some examples to illustrate the distributive property in action:

• 2(3 + 4) = 2(3) + 2(4) = 6 + 8 = 14
• 5(2 + 6) = 5(2) + 5(6) = 10 + 30 = 40
• 3(4 + 2) = 3(4) + 3(2) = 12 + 6 = 18

In each of these examples, we can see how the distributive property helps us simplify the expression by multiplying the number outside the parentheses by each of the numbers inside the parentheses and then adding the results.

Practice Exercises

Now that you’ve seen some examples, it’s time to practice applying the distributive property. Try simplifying the following expressions:

• 4(2 + 5)
• 3(1 + 6)
• 2(7 + 3)

Take your time, and remember to multiply the number outside the parentheses by each of the numbers inside the parentheses and then add the results.

Solving Equations with the Distributive Property

The distributive property is also useful when solving equations. Let’s consider an example:

2(x + 3) = 12

To solve for x, we can use the distributive property to expand the left side of the equation:

2x + 6 = 12

Subtracting 6 from both sides gives us:

2x = 6

Dividing both sides by 2 yields:

x = 3

Real-World Applications of the Distributive Property

The distributive property has numerous real-world applications, including:

• Cost calculation: When calculating the total cost of items, you can use the distributive property to simplify the calculation.
• Area calculation: In geometry, the distributive property helps calculate the area of complex shapes by breaking them down into simpler shapes.
• Science and engineering: The distributive property is used in various scientific and engineering applications, such as calculating force, velocity, and acceleration.

📝 Note: The distributive property is not limited to multiplication. It also applies to addition and subtraction, but the concept is the same.

Conclusion

In conclusion, the distributive property of multiplication is a fundamental concept in mathematics that helps simplify complex expressions and solve equations. By understanding and applying the distributive property, you can improve your math skills and tackle more challenging problems. Remember to practice regularly and apply the distributive property to real-world problems to become more proficient.