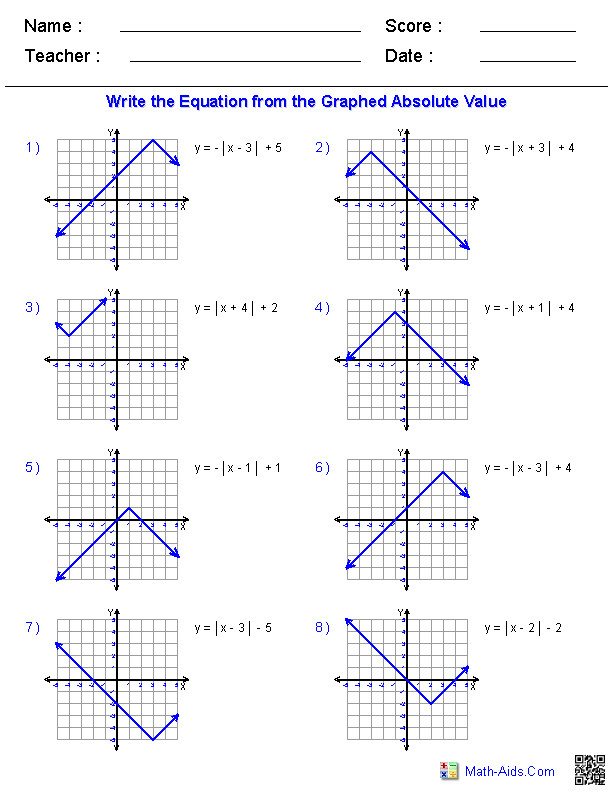
Mastering Dilations on the Coordinate Plane Made Easy

Understanding Dilations on the Coordinate Plane
Dilations are a fundamental concept in geometry, and mastering them can help you solve various problems on the coordinate plane. A dilation is a transformation that changes the size of a figure, but not its shape. In this article, we will delve into the world of dilations, exploring what they are, how to perform them, and some real-world applications.
What is a Dilation?
A dilation is a transformation that enlarges or reduces a figure by a scale factor. The scale factor is a numerical value that determines how much the figure will be enlarged or reduced. For example, if you have a scale factor of 2, the figure will be twice as large as the original. If the scale factor is 1⁄2, the figure will be half the size of the original.
How to Perform a Dilation on the Coordinate Plane
To perform a dilation on the coordinate plane, follow these steps:
- Identify the center of dilation: The center of dilation is the point from which the figure will be enlarged or reduced. It is usually represented by the coordinates (h, k).
- Determine the scale factor: The scale factor will tell you how much the figure will be enlarged or reduced.
- Apply the scale factor: To apply the scale factor, multiply the x and y coordinates of the original figure by the scale factor.
Example:
Suppose you want to dilate a triangle with vertices at (2, 3), (4, 5), and (6, 7) by a scale factor of 2, with the center of dilation at (0, 0).
| Original Triangle | Dilated Triangle |
|---|---|
| (2, 3) | (4, 6) |
| (4, 5) | (8, 10) |
| (6, 7) | (12, 14) |
As you can see, the dilated triangle is twice as large as the original triangle.
Types of Dilations
There are two main types of dilations:
- Enlargement: An enlargement is a dilation that increases the size of a figure. It has a scale factor greater than 1.
- Reduction: A reduction is a dilation that decreases the size of a figure. It has a scale factor less than 1.
Real-World Applications of Dilations
Dilations have numerous real-world applications, including:
- Architecture: Architects use dilations to design buildings and other structures. They can create scale models of their designs and then enlarge or reduce them as needed.
- Graphic Design: Graphic designers use dilations to create visual effects, such as enlarging or reducing images.
- Computer-Aided Design (CAD): CAD software uses dilations to create scale models of objects and designs.
📝 Note: Dilations can also be used to solve problems involving similar figures, where the scale factor is used to determine the ratio of corresponding sides.
Common Mistakes to Avoid
When working with dilations, there are several common mistakes to avoid:
- Incorrect scale factor: Make sure to use the correct scale factor when performing a dilation.
- Incorrect center of dilation: Ensure that you identify the correct center of dilation.
- Not applying the scale factor to all coordinates: Make sure to apply the scale factor to all x and y coordinates of the original figure.
Conclusion
Mastering dilations on the coordinate plane can help you solve a variety of problems in geometry and other fields. By understanding what dilations are, how to perform them, and some real-world applications, you can become proficient in using dilations to solve problems. Remember to avoid common mistakes and always double-check your work.
What is the difference between an enlargement and a reduction?
+An enlargement is a dilation that increases the size of a figure, with a scale factor greater than 1. A reduction is a dilation that decreases the size of a figure, with a scale factor less than 1.
How do I identify the center of dilation?
+The center of dilation is usually represented by the coordinates (h, k). It is the point from which the figure will be enlarged or reduced.
What is the scale factor, and how do I use it?
+The scale factor is a numerical value that determines how much the figure will be enlarged or reduced. To apply the scale factor, multiply the x and y coordinates of the original figure by the scale factor.
Related Terms:
- teacher synergy llc
- Khan Academy
- IXL
- BrainPOP
- Udacity
- Duolingo