Mastering Composition of Transformations with Practice Worksheet

Unlocking the Power of Transformations: A Comprehensive Guide
Transformations are a fundamental concept in mathematics, particularly in geometry and algebra. They involve changing the position, size, or shape of a figure, and are essential for problem-solving and critical thinking. Mastering transformations requires practice, patience, and a deep understanding of the underlying concepts. In this article, we will delve into the world of transformations, exploring the different types, their compositions, and providing a practice worksheet to help you reinforce your understanding.
Types of Transformations
There are four main types of transformations: translations, rotations, reflections, and dilations. Each type has its unique characteristics and applications.
- Translations: A translation is a transformation that moves a figure from one location to another without changing its size or shape. It is equivalent to sliding the figure along a straight line.
- Rotations: A rotation is a transformation that turns a figure around a fixed point, called the center of rotation. The size and shape of the figure remain unchanged.
- Reflections: A reflection is a transformation that flips a figure over a line, called the line of reflection. The figure is mirrored on the opposite side of the line.
- Dilations: A dilation is a transformation that changes the size of a figure, making it larger or smaller. The shape of the figure remains the same.
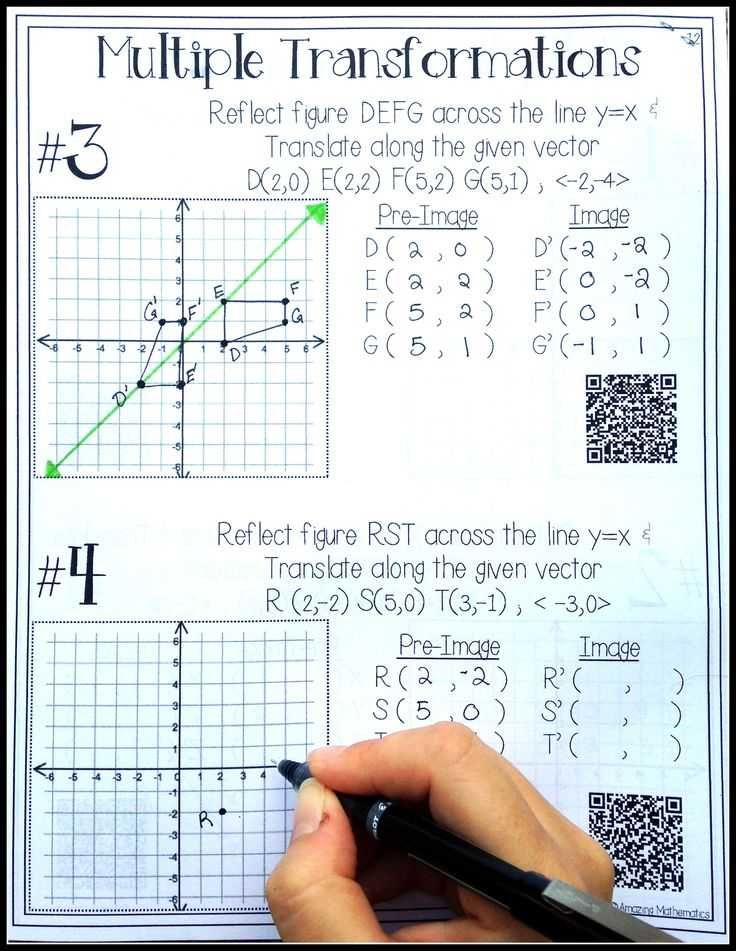
Composition of Transformations
When two or more transformations are combined, the result is called a composition of transformations. The order in which the transformations are applied is crucial, as it can affect the final outcome.
- Translation + Rotation: When a translation is followed by a rotation, the figure is first moved to a new location and then turned around a fixed point.
- Reflection + Dilation: When a reflection is followed by a dilation, the figure is first flipped over a line and then resized.
- Rotation + Translation: When a rotation is followed by a translation, the figure is first turned around a fixed point and then moved to a new location.
Practice Worksheet
To help you master the composition of transformations, we have prepared a practice worksheet with exercises and examples.
Exercise 1: Translation + Rotation
- Draw a triangle with vertices A, B, and C.
- Translate the triangle 5 units to the right.
- Rotate the translated triangle 90° clockwise around the origin.
Exercise 2: Reflection + Dilation
- Draw a rectangle with vertices A, B, C, and D.
- Reflect the rectangle over the x-axis.
- Dilate the reflected rectangle by a scale factor of 2.
Exercise 3: Rotation + Translation
- Draw a circle with center O.
- Rotate the circle 180° counterclockwise around the origin.
- Translate the rotated circle 3 units to the left.
Notes
📝 Note: When composing transformations, it is essential to follow the correct order of operations. The order in which the transformations are applied can significantly affect the final outcome.
📝 Note: To solve transformation problems, use graph paper to visualize the transformations and ensure accuracy.
Table of Transformation Notations
| Transformation | Notation |
|---|---|
| Translation | T(x, y) = (x + a, y + b) |
| Rotation | R(θ) = (x cos θ - y sin θ, x sin θ + y cos θ) |
| Reflection | F(x, y) = (x, -y) |
| Dilation | D(k) = (kx, ky) |
In Conclusion
Mastering the composition of transformations requires practice, patience, and a deep understanding of the underlying concepts. By following the exercises and examples in this article, you will develop a solid foundation in transformations and be able to tackle more complex problems with confidence.
What is the difference between a translation and a rotation?
+A translation moves a figure from one location to another without changing its size or shape, while a rotation turns a figure around a fixed point without changing its size or shape.
How do you compose two transformations?
+To compose two transformations, apply the first transformation to the original figure, and then apply the second transformation to the result.
What is the notation for a dilation?
+The notation for a dilation is D(k) = (kx, ky), where k is the scale factor.