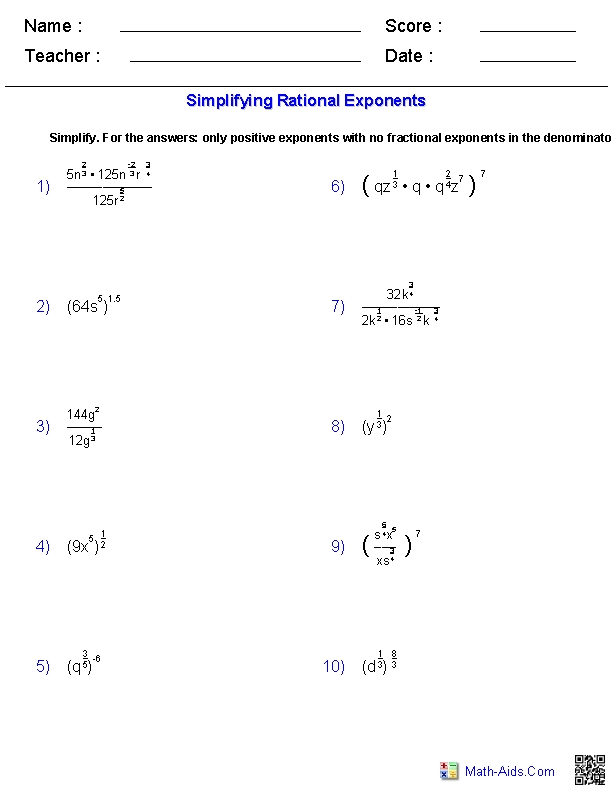
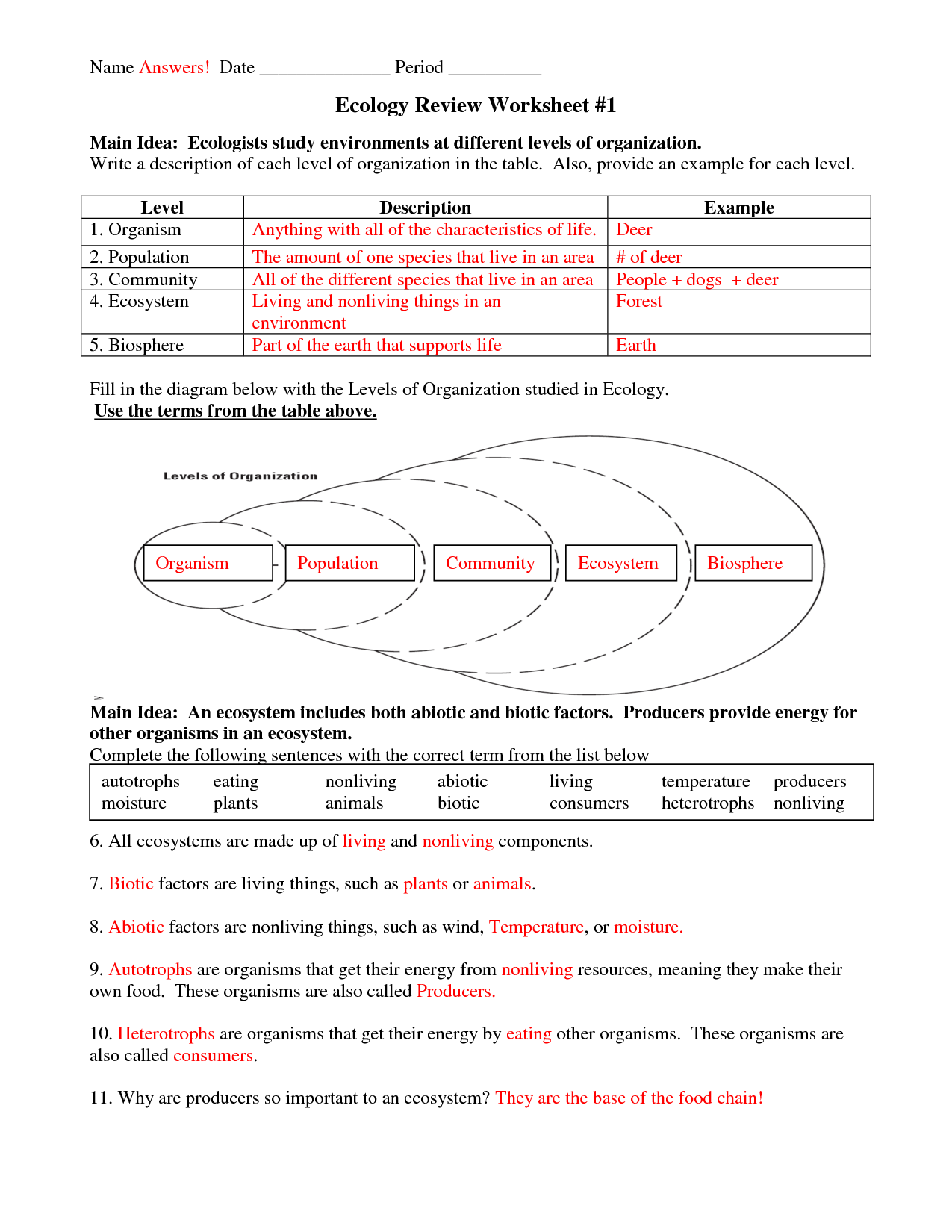
7 Ways to Master Composite Area Problems
Understanding Composite Area Problems
Composite area problems involve finding the total area of a shape that is composed of multiple parts, such as rectangles, triangles, and circles. These problems require the ability to visualize and break down complex shapes into simpler components, calculate the area of each component, and then sum the areas to find the total area. Mastering composite area problems is essential for success in geometry and other areas of mathematics.
1. Break Down Complex Shapes
The first step in solving composite area problems is to break down the complex shape into simpler components. This involves identifying the individual parts of the shape, such as rectangles, triangles, and circles, and determining their dimensions. By breaking down the shape into smaller parts, you can calculate the area of each part and then sum the areas to find the total area.
Example:
Find the area of the following composite shape:
Image of a composite shape with a rectangle and two triangles
To solve this problem, break down the shape into its individual parts:
- Rectangle with dimensions 4 cm x 6 cm
- Two triangles with base 4 cm and height 3 cm each
Calculate the area of each part and then sum the areas to find the total area.
📝 Note: When breaking down complex shapes, make sure to identify all the individual parts and their dimensions.
2. Calculate the Area of Each Part
Once you have broken down the complex shape into simpler components, calculate the area of each part. The formula for the area of a rectangle is length x width, the formula for the area of a triangle is 1⁄2 x base x height, and the formula for the area of a circle is πr^2.
Example:
Calculate the area of each part of the composite shape:
- Rectangle: 4 cm x 6 cm = 24 cm^2
- Triangle 1: 1⁄2 x 4 cm x 3 cm = 6 cm^2
- Triangle 2: 1⁄2 x 4 cm x 3 cm = 6 cm^2
3. Sum the Areas to Find the Total Area
Once you have calculated the area of each part, sum the areas to find the total area of the composite shape.
Example:
Sum the areas of the rectangle and two triangles:
24 cm^2 + 6 cm^2 + 6 cm^2 = 36 cm^2
The total area of the composite shape is 36 cm^2.
4. Use Formulas to Calculate the Area of Irregular Shapes
Some composite area problems involve irregular shapes, such as trapezoids and parallelograms. To calculate the area of these shapes, use the following formulas:
- Trapezoid: 1⁄2 x (base1 + base2) x height
- Parallelogram: base x height
Example:
Calculate the area of a trapezoid with bases 5 cm and 7 cm and height 4 cm:
1⁄2 x (5 cm + 7 cm) x 4 cm = 24 cm^2
5. Practice with Different Types of Composite Area Problems
To master composite area problems, practice with different types of problems, including:
- Rectangles and triangles
- Circles and semicircles
- Trapezoids and parallelograms
- Irregular shapes with multiple parts
Practice with different types of problems to develop your skills and build your confidence.
6. Check Your Units
When solving composite area problems, make sure to check your units. Ensure that all the units are consistent, such as square centimeters or square meters.
7. Visualize the Shape
Finally, visualize the shape to ensure that you have broken it down correctly and calculated the area of each part correctly. Visualizing the shape can help you identify any mistakes and ensure that you have found the correct total area.
Conclusion:
Mastering composite area problems requires breaking down complex shapes into simpler components, calculating the area of each part, and summing the areas to find the total area. By following these steps and practicing with different types of problems, you can develop your skills and build your confidence in solving composite area problems.
What is a composite area problem?
+A composite area problem is a type of geometry problem that involves finding the total area of a shape that is composed of multiple parts, such as rectangles, triangles, and circles.
How do I break down a complex shape into simpler components?
+To break down a complex shape into simpler components, identify the individual parts of the shape, such as rectangles, triangles, and circles, and determine their dimensions.
What formulas do I use to calculate the area of each part?
+The formulas for calculating the area of each part are:
- Rectangle: length x width
- Triangle: 1⁄2 x base x height
- Circle: πr^2
Related Terms:
- Area of compound shapes worksheet
- Compound shape area worksheet
- Area Composite Figures worksheet PDF