Simplifying Radicals and Rational Exponents Made Easy

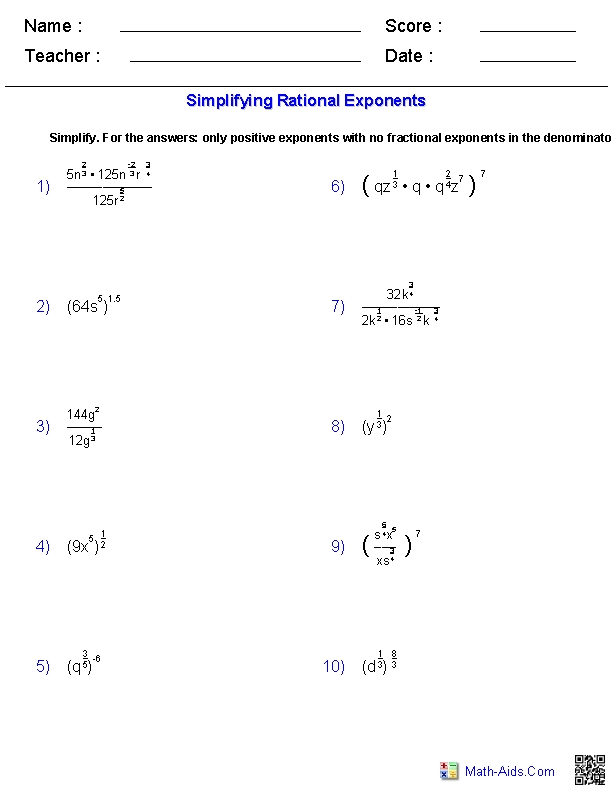
Introduction to Radicals and Rational Exponents

When it comes to dealing with radicals and rational exponents, many students find themselves struggling to understand the concepts. However, with a few simple rules and techniques, simplifying radicals and rational exponents can be made easy.

Understanding Radicals

A radical is a symbol used to represent a root of a number. For example, √(2) represents the square root of 2. There are different types of radicals, including square roots, cube roots, and fourth roots, each represented by a different symbol. Radicals can be simplified by finding the largest perfect square or perfect cube that divides the radicand (the number inside the radical).

Simplifying Radicals

To simplify a radical, follow these steps:

1. Factor the radicand: Factor the number inside the radical into prime factors.
2. Find the largest perfect square or perfect cube: Identify the largest perfect square or perfect cube that divides the radicand.
3. Write the simplified radical: Write the simplified radical with the largest perfect square or perfect cube as the coefficient and the remaining factor as the radicand.

Example:

√(48) = √(16 × 3) = 4√(3)

Understanding Rational Exponents

Rational exponents are exponents that are fractions. They can be used to represent radicals in a more compact form. For example, 2^(¹⁄₂) represents the square root of 2.

Simplifying Rational Exponents

To simplify a rational exponent, follow these steps:

1. Write the rational exponent as a radical: Convert the rational exponent to a radical using the formula: a^(m/n) = √(a^m).
2. Simplify the radical: Simplify the radical using the steps outlined above.

Example:

2^(¹⁄₂) = √(2)

Rules for Simplifying Radicals and Rational Exponents

Here are some important rules to keep in mind when simplifying radicals and rational exponents:

• Product rule: √(ab) = √(a) × √(b)
• Quotient rule: √(a/b) = √(a) / √(b)
• Power rule: (a^m)^(n) = a^(mn)

Example:

√(16 × 2) = √(16) × √(2) = 4√(2)

Common Mistakes to Avoid

When simplifying radicals and rational exponents, it’s easy to make mistakes. Here are some common mistakes to avoid:

• Incorrectly simplifying radicals: Make sure to simplify radicals by finding the largest perfect square or perfect cube that divides the radicand.
• Forgetting to simplify rational exponents: Don’t forget to simplify rational exponents by writing them as radicals and then simplifying the radical.

🤔 Note: Simplifying radicals and rational exponents can be tricky, but with practice, you'll become more confident and proficient.

Conclusion

Simplifying radicals and rational exponents can be made easy with a few simple rules and techniques. By understanding the concepts and practicing regularly, you’ll become more confident and proficient in your ability to simplify radicals and rational exponents. Remember to avoid common mistakes and always simplify radicals and rational exponents by following the rules and steps outlined above.

Related Terms:

• Exponents and Radicals Worksheet pdf
• Simplify Rational Exponents Worksheet