5 Ways to Calculate Net Force Easily
Understanding Net Force and Its Importance
When dealing with objects in motion, one of the fundamental concepts in physics is net force. The net force acting on an object is the vector sum of all the forces acting on it, and it plays a crucial role in determining the object’s acceleration, velocity, and overall motion. Calculating net force can seem daunting, especially when multiple forces are involved, but with the right approaches, it can be simplified.
Method 1: Using the Vector Addition Method
One of the most straightforward methods for calculating net force is by using vector addition. This method involves breaking down each force into its x and y components, adding these components together, and then finding the resultant vector.
For example, consider a box being pulled by two ropes, one with a force of 20 N at an angle of 30° and the other with a force of 15 N at an angle of 60°. To calculate the net force, you would:
- Break down each force into its x and y components using trigonometry
- Add the x components and y components separately
- Use the Pythagorean theorem to find the magnitude of the resultant vector
📝 Note: When using the vector addition method, make sure to pay close attention to the signs of the components, as they can affect the direction of the resultant vector.
Method 2: Using the Concept of Equilibrium
Another approach to calculating net force is by using the concept of equilibrium. When an object is in equilibrium, the net force acting on it is zero. By setting the sum of all forces equal to zero, you can solve for the unknown forces.
For instance, consider a tug-of-war game between two teams. If the teams are evenly matched and the rope is stationary, the net force acting on the rope is zero. You can set up an equation using the forces exerted by each team and solve for the unknown forces.
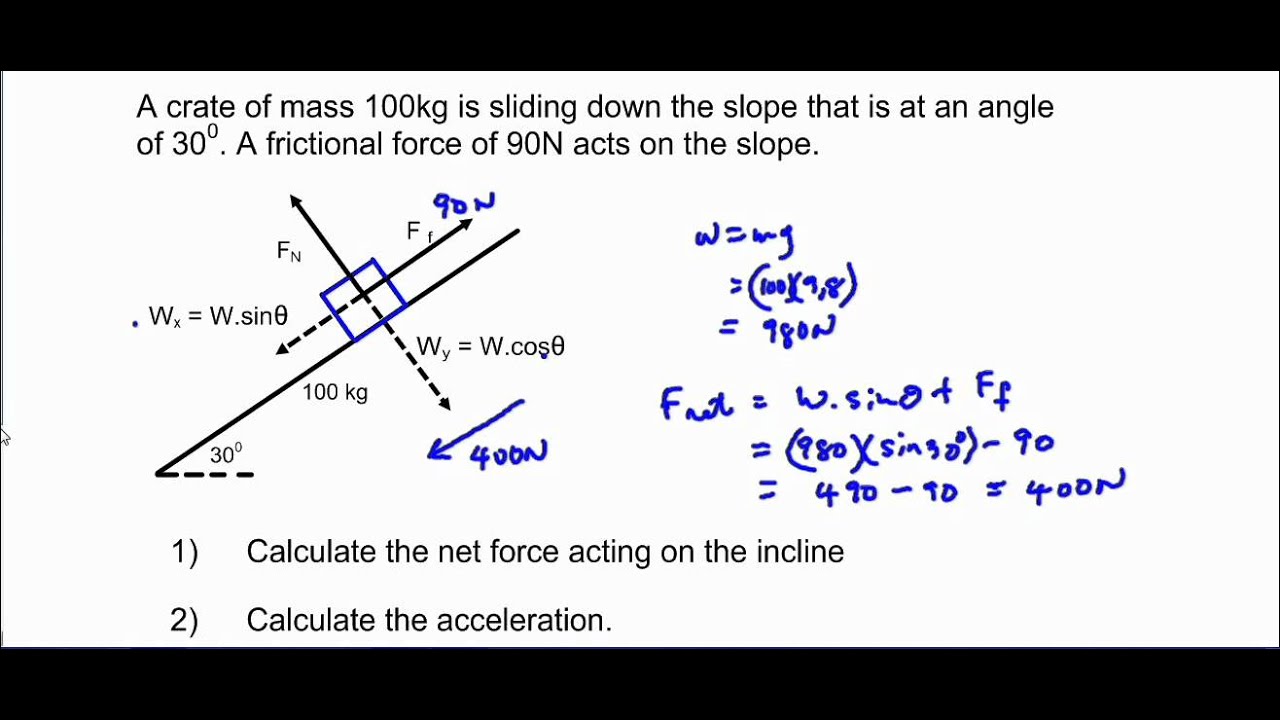
Method 3: Using Free Body Diagrams
Free body diagrams (FBDs) are a powerful tool for visualizing and calculating net force. An FBD is a diagram that shows all the forces acting on an object, including the magnitude and direction of each force.
To use FBDs, start by drawing a diagram of the object and labeling all the forces acting on it. Then, use the diagram to determine the net force by adding up the forces in each direction.
For example, consider a car driving uphill on a frictionless road. The forces acting on the car include the force of gravity, the normal force, and the force exerted by the engine. By drawing an FBD, you can visualize the forces and calculate the net force acting on the car.
Method 4: Using Newton's Second Law
Newton’s Second Law states that the net force acting on an object is equal to its mass multiplied by its acceleration (F = ma). By rearranging this equation, you can solve for net force.
For example, consider a car accelerating from 0 to 60 mph in 10 seconds. If the car’s mass is 1500 kg, you can use Newton’s Second Law to calculate the net force acting on it.
Method 5: Using a Force Table or Chart
A force table or chart is a graphical representation of the forces acting on an object. By using a force table, you can visualize the forces and calculate the net force by adding up the forces in each direction.
For example, consider a box being pulled by multiple ropes. By drawing a force table, you can label each force and calculate the net force by adding up the forces in each direction.
| Force | Magnitude (N) | Direction (°) |
|---|---|---|
| Force 1 | 20 | 30° |
| Force 2 | 15 | 60° |
| Force 3 | 10 | 90° |
By using one or a combination of these methods, you can easily calculate the net force acting on an object. Remember to always pay close attention to the units and signs of the forces, as they can affect the direction and magnitude of the net force.
To summarize, calculating net force is a crucial step in understanding an object’s motion. By using the vector addition method, the concept of equilibrium, free body diagrams, Newton’s Second Law, or a force table, you can simplify the calculation and gain a deeper understanding of the forces at play. Whether you’re a student or a professional, mastering the art of calculating net force is essential for success in physics and engineering.