Grade 6 Order of Operations Worksheet Made Easy
Grade 6 Order of Operations Worksheet Made Easy
Are you a Grade 6 student struggling with the order of operations in mathematics? Do you find it difficult to remember the correct sequence of operations when solving mathematical expressions? Worry no more! This blog post is designed to help you understand and master the order of operations, also known as PEMDAS.
What is the Order of Operations?
The order of operations is a set of rules that tells you which operations to perform first when you have multiple operations in an expression. The acronym PEMDAS is commonly used to remember the order of operations:
- Parentheses: Evaluate expressions inside parentheses first.
- Exponents: Evaluate any exponential expressions next (for example, 2^3).
- Multiplication and Division: Evaluate any multiplication and division operations from left to right.
- Addition and Subtraction: Finally, evaluate any addition and subtraction operations from left to right.
Why is the Order of Operations Important?
The order of operations is crucial in mathematics because it helps to avoid confusion and ensures that mathematical expressions are evaluated consistently. Without a standard order of operations, different people might interpret mathematical expressions differently, leading to incorrect answers.
Examples to Illustrate the Order of Operations
Let’s consider a few examples to illustrate how the order of operations works:
Example 1: 2 × 3 + 10 - 5
Using the order of operations, we evaluate this expression as follows:
- Multiply 2 and 3: 2 × 3 = 6
- Add 10: 6 + 10 = 16
- Subtract 5: 16 - 5 = 11
Therefore, the final answer is 11.
Example 2: 12 - 3 + 2^2
Using the order of operations, we evaluate this expression as follows:
- Evaluate the exponent: 2^2 = 4
- Subtract 3: 12 - 3 = 9
- Add 4: 9 + 4 = 13
Therefore, the final answer is 13.
Tips for Mastering the Order of Operations
Here are a few tips to help you master the order of operations:
- Always follow the order of operations when evaluating mathematical expressions.
- Use parentheses to group expressions and make them easier to evaluate.
- Evaluate expressions inside parentheses first.
- Evaluate exponential expressions next.
- Evaluate multiplication and division operations from left to right.
- Finally, evaluate addition and subtraction operations from left to right.
Common Mistakes to Avoid
Here are a few common mistakes to avoid when working with the order of operations:
- Forgetting to evaluate expressions inside parentheses first.
- Evaluating exponential expressions after multiplication and division operations.
- Evaluating addition and subtraction operations before multiplication and division operations.
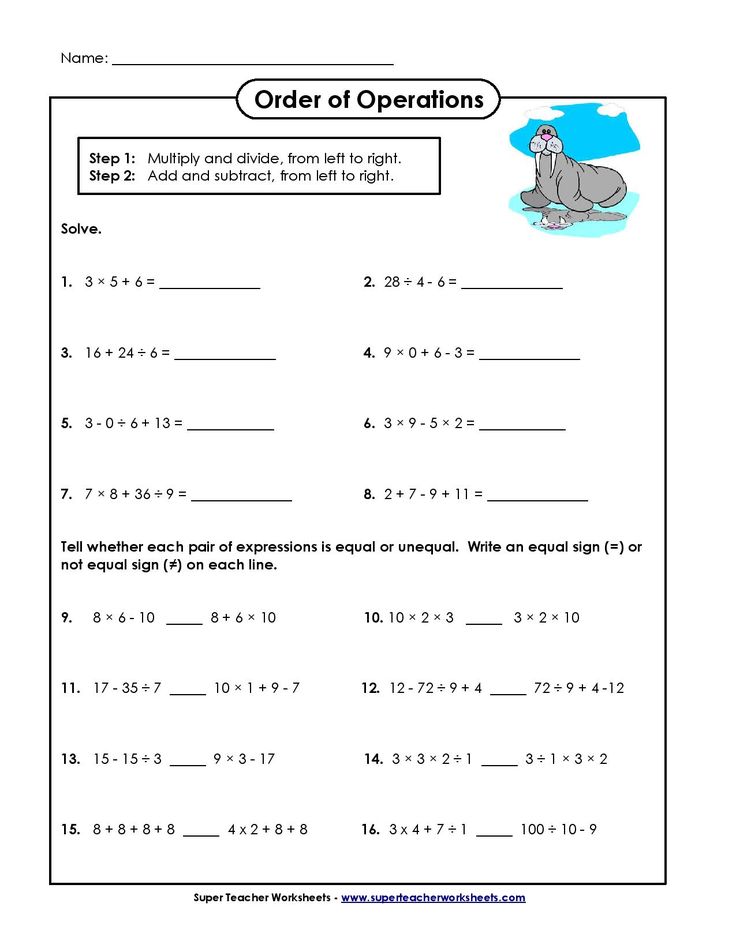
Practice Time!
Now that you have a good understanding of the order of operations, it’s time to practice! Here are a few exercises to help you reinforce your understanding:
- 3 × 2 + 10 - 4 =
- 15 - 3 + 2^2 =
- 24 ÷ 3 + 2 - 1 =
Answers
- 3 × 2 + 10 - 4 = 12
- 15 - 3 + 2^2 = 16
- 24 ÷ 3 + 2 - 1 = 9
Conclusion
Mastering the order of operations is essential for success in mathematics. By following the order of operations, you can ensure that you evaluate mathematical expressions correctly and avoid confusion. Remember to practice regularly and use the tips and examples provided in this blog post to help you reinforce your understanding.
Here is a table of some more examples and their answers
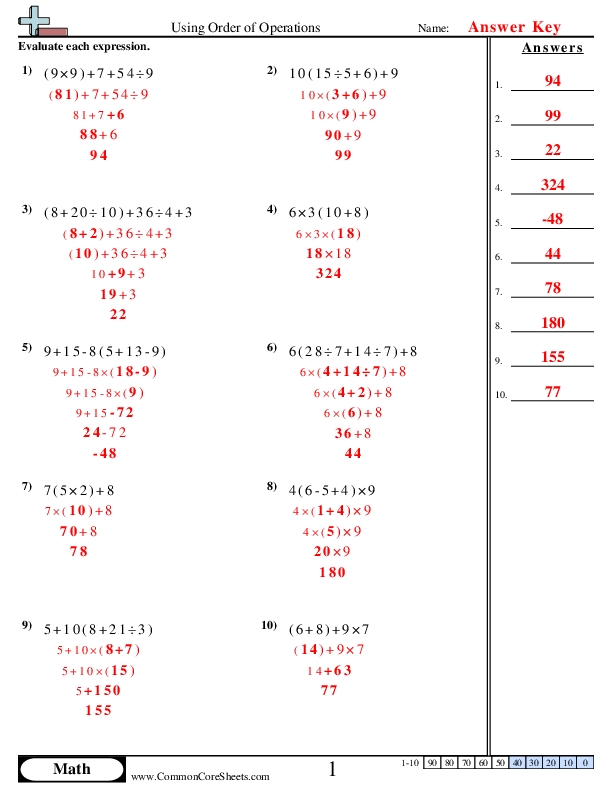
| Expression | Answer |
|---|---|
| 2 × 3 + 10 - 5 | 11 |
| 12 - 3 + 2^2 | 13 |
| 3 × 2 + 10 - 4 | 12 |
| 15 - 3 + 2^2 | 16 |
| 24 ÷ 3 + 2 - 1 | 9 |
FAQ Section
What is the order of operations?
+The order of operations is a set of rules that tells you which operations to perform first when you have multiple operations in an expression. The acronym PEMDAS is commonly used to remember the order of operations: Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction.
Why is the order of operations important?
+The order of operations is crucial in mathematics because it helps to avoid confusion and ensures that mathematical expressions are evaluated consistently. Without a standard order of operations, different people might interpret mathematical expressions differently, leading to incorrect answers.
How can I master the order of operations?
+To master the order of operations, always follow the order of operations when evaluating mathematical expressions. Use parentheses to group expressions and make them easier to evaluate. Practice regularly and use the tips and examples provided in this blog post to help you reinforce your understanding.