5 Ways to Master Angles in Triangles

Understanding Angles in Triangles

Angles are a fundamental aspect of triangles, and mastering them is crucial for success in geometry and trigonometry. A triangle is a polygon with three sides and three angles, and the sum of these angles is always 180 degrees. In this article, we will explore five ways to master angles in triangles, including understanding the different types of angles, using the angle sum property, and applying trigonometric ratios.

Type of Angles in Triangles

There are several types of angles in triangles, including:

• Acute angles: Angles less than 90 degrees.
• Right angles: Angles equal to 90 degrees.
• Obtuse angles: Angles greater than 90 degrees.
• Straight angles: Angles equal to 180 degrees.

Understanding the different types of angles is essential for solving problems involving triangles.

Angle Sum Property

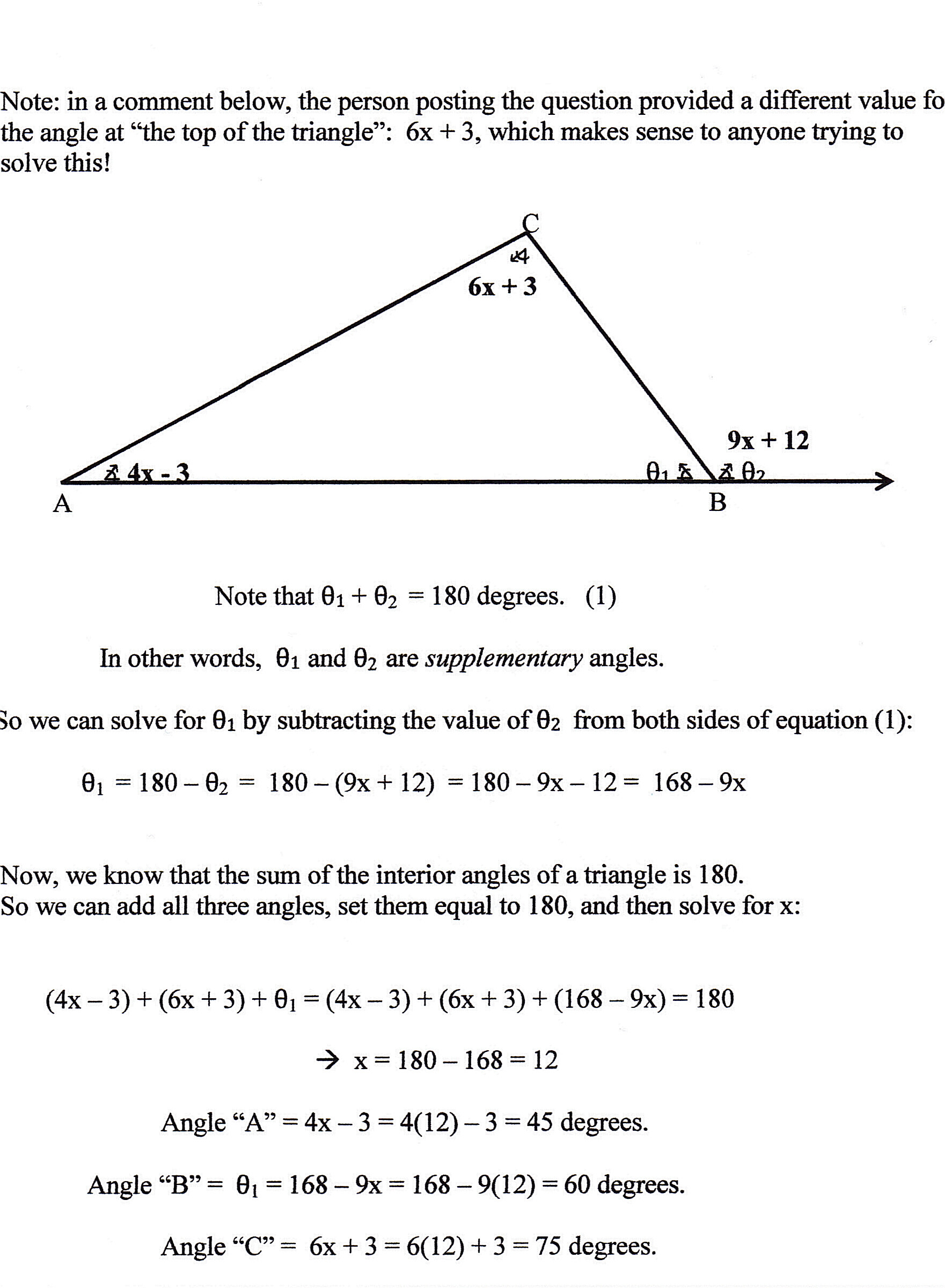
The angle sum property states that the sum of the angles in a triangle is always 180 degrees. This property can be used to find the measure of an unknown angle in a triangle.

For example, if we have a triangle with two angles measuring 60 degrees and 80 degrees, we can use the angle sum property to find the measure of the third angle:

📝 Note: The angle sum property can be used to find the measure of an unknown angle in a triangle.

60 + 80 + x = 180

Solving for x, we get:

x = 40

Therefore, the measure of the third angle is 40 degrees.

Trigonometric Ratios

Trigonometric ratios are used to relate the angles of a triangle to the lengths of its sides. The three main trigonometric ratios are:

• Sine (sin): The ratio of the length of the side opposite an angle to the length of the hypotenuse.
• Cosine (cos): The ratio of the length of the side adjacent to an angle to the length of the hypotenuse.
• Tangent (tan): The ratio of the length of the side opposite an angle to the length of the side adjacent to an angle.

Trigonometric ratios can be used to find the measure of an angle in a triangle, given the lengths of its sides.

Using the Law of Sines

The law of sines is a fundamental concept in trigonometry that relates the angles of a triangle to the lengths of its sides. The law of sines states that:

a / sin(A) = b / sin(B) = c / sin©

where a, b, and c are the lengths of the sides opposite angles A, B, and C, respectively.

The law of sines can be used to find the measure of an angle in a triangle, given the lengths of its sides.

Using the Law of Cosines

The law of cosines is another fundamental concept in trigonometry that relates the angles of a triangle to the lengths of its sides. The law of cosines states that:

c² = a² + b² - 2ab * cos©

where a, b, and c are the lengths of the sides opposite angles A, B, and C, respectively.

The law of cosines can be used to find the measure of an angle in a triangle, given the lengths of its sides.

In conclusion, mastering angles in triangles requires a deep understanding of the different types of angles, the angle sum property, and trigonometric ratios. By applying these concepts, you can solve problems involving triangles with confidence.