Angle of Elevation and Depression Problems and Solutions
Understanding Angles of Elevation and Depression
Angles of elevation and depression are fundamental concepts in trigonometry and geometry, often encountered in various real-world applications, such as physics, engineering, and architecture. These angles are used to describe the position of an object or a line relative to the horizontal plane. In this post, we will delve into the world of angles of elevation and depression, exploring their definitions, formulas, and problem-solving strategies.
Definitions and Formulas
Angle of Elevation
The angle of elevation is the angle between a horizontal plane and a line connecting the observer’s eye to an object above the horizon. It is measured clockwise from the horizontal plane.
Formula: tan(θ) = opposite side (height) / adjacent side (distance)
Angle of Depression
The angle of depression is the angle between a horizontal plane and a line connecting the observer’s eye to an object below the horizon. It is measured counterclockwise from the horizontal plane.
Formula: tan(θ) = opposite side (depth) / adjacent side (distance)
Problem-Solving Strategies
To solve problems involving angles of elevation and depression, follow these steps:
- Read the problem carefully: Understand the given information, including the angle, distance, height, or depth.
- Draw a diagram: Visualize the situation using a sketch or diagram.
- Identify the angle: Determine whether the angle is an angle of elevation or depression.
- Apply the formula: Use the tangent function to relate the angle, opposite side, and adjacent side.
- Solve for the unknown: Use algebraic manipulations to find the desired value.
Example Problems and Solutions
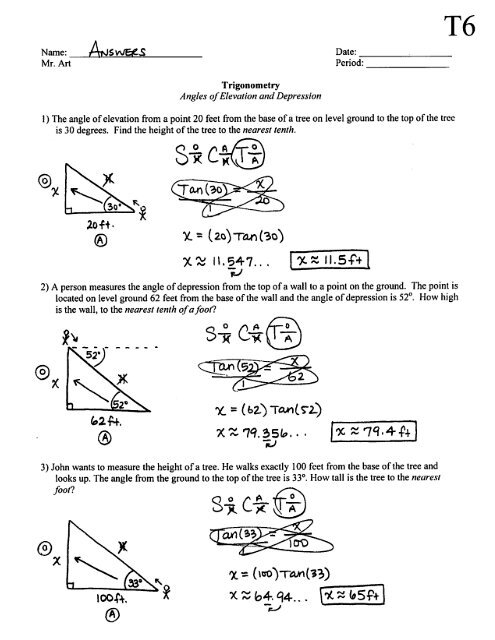
Problem 1: Angle of Elevation
A surveyor measures the angle of elevation to the top of a building as 30°. If the distance from the surveyor to the building is 50 meters, find the height of the building.
Solution:
tan(30°) = height / 50 height = 50 × tan(30°) height ≈ 28.86 meters
📝 Note: Make sure to use the correct angle and formula for the problem.
Problem 2: Angle of Depression
A diver is 20 meters below the surface of the water. If the angle of depression from the surface to the diver is 40°, find the distance from the point directly above the diver to the surface.
Solution:
tan(40°) = 20 / distance distance = 20 / tan(40°) distance ≈ 24.65 meters
Table of Formulas and Definitions
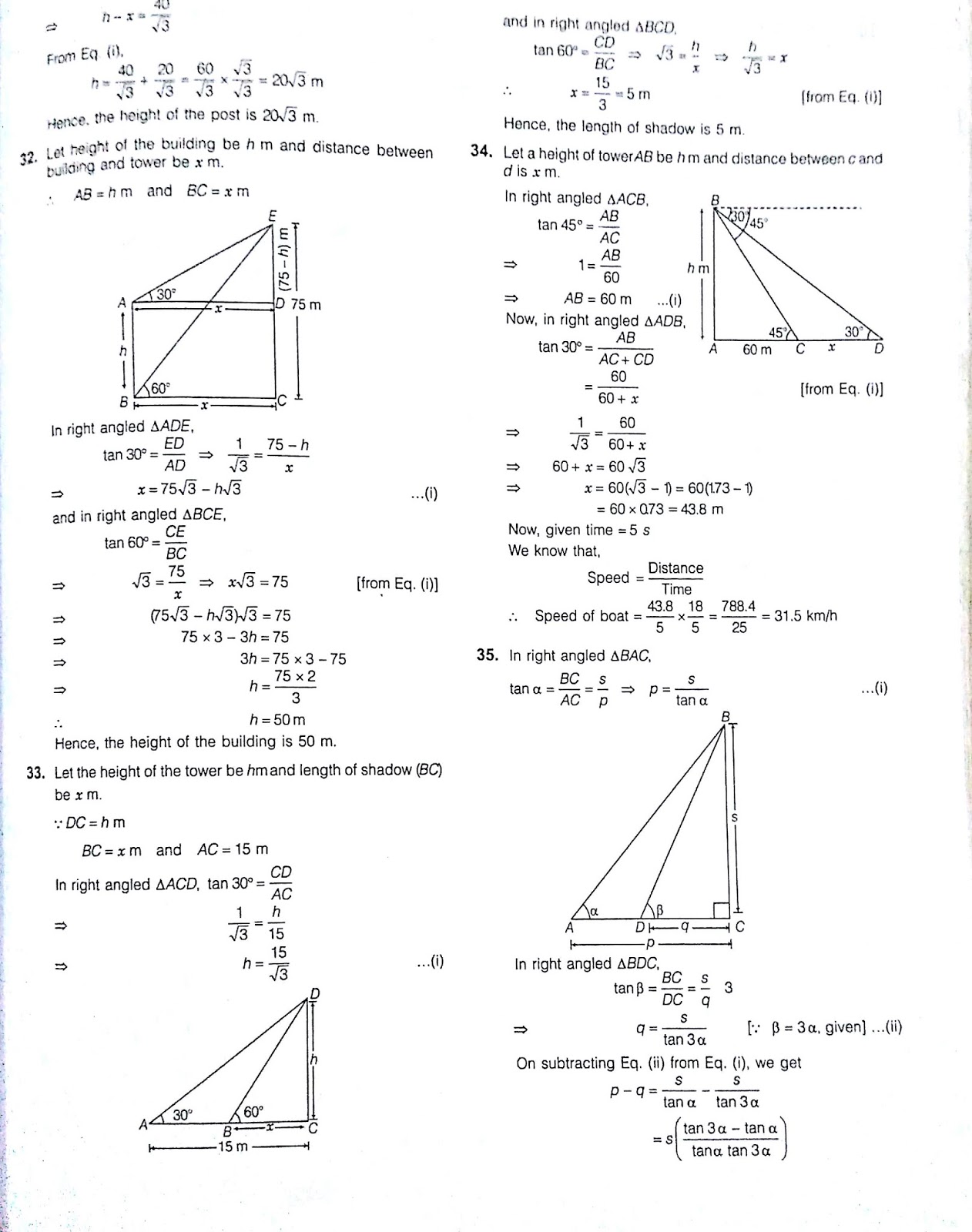
| Concept | Formula | Definition |
|---|---|---|
| Angle of Elevation | tan(θ) = height / distance | Angle between horizontal plane and line to object above horizon |
| Angle of Depression | tan(θ) = depth / distance | Angle between horizontal plane and line to object below horizon |
Conclusion
In this post, we explored the world of angles of elevation and depression, including their definitions, formulas, and problem-solving strategies. By mastering these concepts, you’ll be better equipped to tackle problems in trigonometry, geometry, and various real-world applications. Remember to carefully read the problem, draw a diagram, and apply the correct formula to solve for the unknown value.
What is the difference between an angle of elevation and an angle of depression?
+An angle of elevation is measured clockwise from the horizontal plane to an object above the horizon, while an angle of depression is measured counterclockwise from the horizontal plane to an object below the horizon.
How do I determine which formula to use for an angle of elevation or depression problem?
+Use the tangent function for both angles of elevation and depression. The formula is tan(θ) = opposite side / adjacent side. For angles of elevation, the opposite side is the height, and for angles of depression, the opposite side is the depth.
What are some real-world applications of angles of elevation and depression?
+Angles of elevation and depression are used in various fields, including physics, engineering, architecture, and surveying. They are essential for calculating distances, heights, and depths in construction, navigation, and environmental monitoring.