7 Ways to Solve Equations with Fractions Easily
Equations with Fractions: Simplifying the Complex
Equations with fractions can be intimidating, but with the right strategies, they can be solved with ease. In this article, we will explore 7 ways to solve equations with fractions easily. Whether you’re a student struggling with math or a professional looking to refresh your skills, these methods will help you tackle even the most complex equations.
Method 1: Clearing Fractions by Multiplying by the LCD
One of the most common methods for solving equations with fractions is to multiply both sides of the equation by the Least Common Denominator (LCD). This will eliminate the fractions, allowing you to solve the equation as you would with whole numbers.
Example:
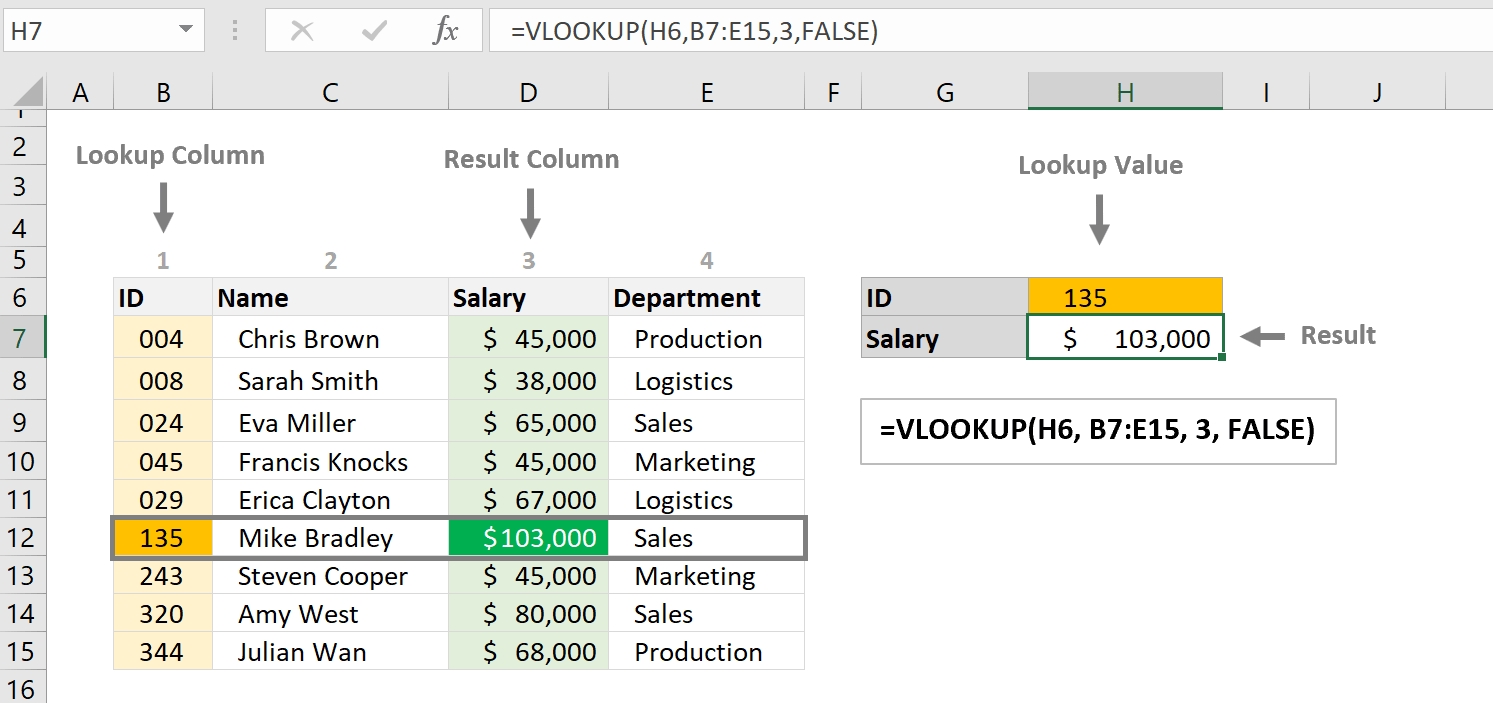
Solve for x: 1/2x + 1⁄4 = 1⁄2
To solve this equation, multiply both sides by 4, the LCD:
4(1/2x + 1⁄4) = 4(1⁄2)
2x + 1 = 2
Subtract 1 from both sides:
2x = 1
Divide by 2:
x = 1⁄2
📝 Note: The LCD is the smallest multiple that both denominators (2 and 4) can divide into evenly.
Method 2: Using Inverse Operations
Another way to solve equations with fractions is to use inverse operations. This involves adding, subtracting, multiplying, or dividing both sides of the equation by the same value to isolate the variable.
Example:
Solve for x: x/3 - 2 = 1⁄2
Add 2 to both sides:
x/3 = 1⁄2 + 2
x/3 = 5⁄2
Multiply both sides by 3:
x = 15⁄2
Method 3: Finding a Common Denominator
When dealing with multiple fractions in an equation, finding a common denominator can simplify the process. This involves identifying the smallest multiple that all denominators can divide into evenly.
Example:
Solve for x: 1/2x + 1⁄6 = 1⁄3
The common denominator is 6. Multiply both sides by 6:
3x + 1 = 2
Subtract 1 from both sides:
3x = 1
Divide by 3:
x = 1⁄3
Method 4: Using Visual Models
Visual models, such as diagrams or charts, can help you understand the relationship between fractions in an equation. This method is particularly useful for visual learners.
Example:
Solve for x: 1/2x = 1⁄4 + 1⁄8
Draw a diagram to represent the fractions:
[Visual diagram of 1/2x = 1⁄4 + 1⁄8]
From the diagram, you can see that 1/2x is equal to 3⁄8. Multiply both sides by 2:
x = 3⁄4
Method 5: Simplifying Complex Fractions
Complex fractions are fractions that contain other fractions in the numerator or denominator. Simplifying these fractions can make it easier to solve equations.
Example:
Solve for x: (1/2x + 1⁄4) / (3⁄4 - 1⁄2) = 1
Simplify the complex fraction:
(1/2x + 1⁄4) / (1⁄4) = 1
Multiply both sides by 1⁄4:
1/2x + 1⁄4 = 1⁄4
Subtract 1⁄4 from both sides:
1/2x = 0
Multiply both sides by 2:
x = 0
Method 6: Using Algebraic Properties
Algebraic properties, such as the distributive property and the commutative property, can help you simplify equations with fractions.
Example:
Solve for x: 1⁄2(x + 2) = 1⁄4
Distribute the 1⁄2:
1/2x + 1 = 1⁄4
Subtract 1 from both sides:
1/2x = -3⁄4
Multiply both sides by 2:
x = -3⁄2
Method 7: Checking Your Work
Finally, it’s essential to check your work when solving equations with fractions. Plug your solution back into the original equation to ensure it’s true.
Example:
Solve for x: 1/2x + 1⁄4 = 1⁄2
Solution: x = 1⁄2
Check:
1⁄2(1⁄2) + 1⁄4 = 1⁄2
1⁄4 + 1⁄4 = 1⁄2
1⁄2 = 1⁄2
True!
What is the Least Common Denominator (LCD)?
+The LCD is the smallest multiple that both denominators can divide into evenly.
Why is it essential to check your work when solving equations with fractions?
+Checking your work ensures that your solution is true and helps you identify any mistakes.
Can I use visual models to solve all types of equations with fractions?
+Visual models are particularly useful for visual learners, but they may not be suitable for all types of equations with fractions.
In conclusion, solving equations with fractions can be challenging, but with the right strategies, it can be done with ease. By mastering these 7 methods, you’ll become proficient in solving even the most complex equations. Remember to always check your work and use the method that works best for you.