Division Exponents Worksheet

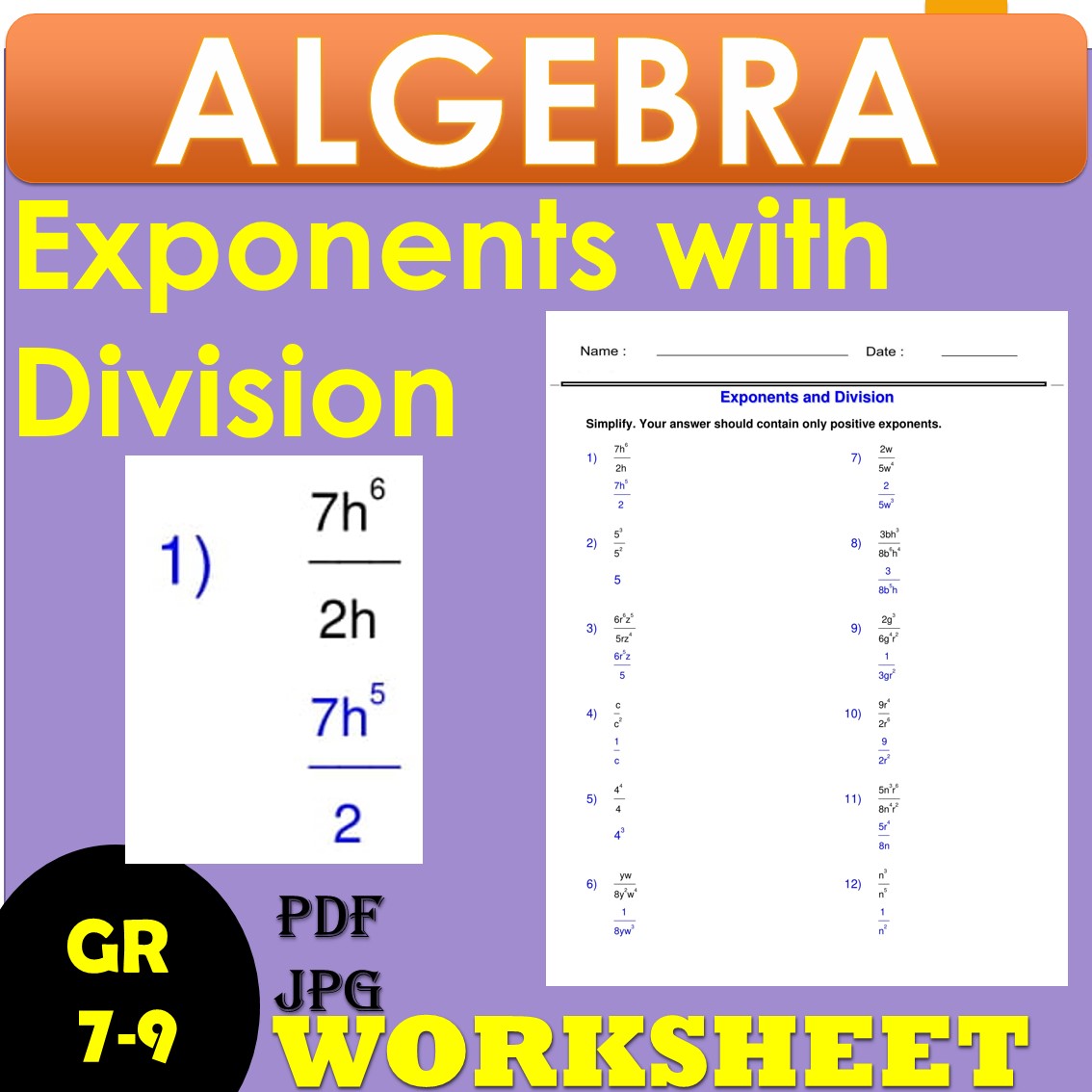
Understanding Division Exponents

When dealing with exponents, division is a crucial operation that can be simplified using specific rules. In this worksheet, we will explore the concept of division exponents and provide examples to help solidify understanding.

Rules for Division Exponents

There are two main rules for dividing exponents:

1. Quotient Rule: When dividing two powers with the same base, subtract the exponents. That is, ( \frac{a^m}{a^n} = a^{m-n} ).
2. Zero Exponent Rule: Any number raised to the power of zero is equal to 1. That is, ( a^0 = 1 ).

Examples of Division Exponents

Example 1: Simplifying Division Exponents

Simplify the expression: ( \frac{2^4}{2^2} )

Using the Quotient Rule, we subtract the exponents:

( \frac{2^4}{2^2} = 2^{4-2} = 2^2 = 4 )

Example 2: Division Exponents with Different Bases

Simplify the expression: ( \frac{3^2}{2^2} )

Since the bases are different, we cannot simplify further using the Quotient Rule.

( \frac{3^2}{2^2} = \frac{9}{4} )

Example 3: Division Exponents with Zero Exponents

Simplify the expression: ( \frac{2^3}{2^0} )

Using the Zero Exponent Rule, we know that ( 2^0 = 1 ).

( \frac{2^3}{2^0} = \frac{2^3}{1} = 2^3 = 8 )

Practice Problems

Here are some practice problems to help reinforce understanding:

• Simplify the expression: ( \frac{4^2}{4^1} )
• Simplify the expression: ( \frac{3^5}{3^2} )
• Simplify the expression: ( \frac{2^6}{2^4} )

Solutions

Solution 1

Using the Quotient Rule, we subtract the exponents:

( \frac{4^2}{4^1} = 4^{2-1} = 4^1 = 4 )

Solution 2

Using the Quotient Rule, we subtract the exponents:

( \frac{3^5}{3^2} = 3^{5-2} = 3^3 = 27 )

Solution 3

Using the Quotient Rule, we subtract the exponents:

( \frac{2^6}{2^4} = 2^{6-4} = 2^2 = 4 )

Important Notes

• When dividing exponents with the same base, always subtract the exponents.
• When dividing exponents with different bases, simplify the expression as much as possible, but do not simplify further using the Quotient Rule.
• Remember that any number raised to the power of zero is equal to 1.

In conclusion, division exponents can be simplified using the Quotient Rule and the Zero Exponent Rule. By understanding and applying these rules, you can simplify complex expressions and improve your math skills.