Algebra Expressions Worksheet for Students and Teachers
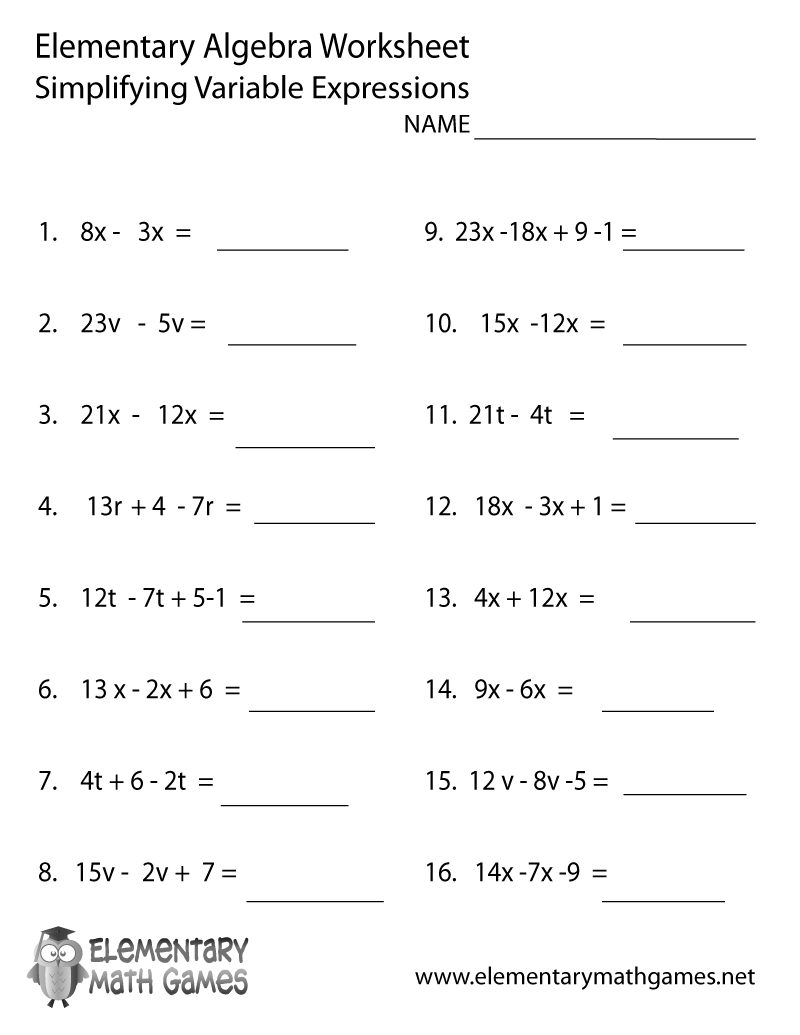
Understanding Algebra Expressions
Algebra expressions are a fundamental concept in mathematics, and mastering them is crucial for students to succeed in various mathematical disciplines. In this blog post, we will delve into the world of algebra expressions, exploring what they are, their components, and how to simplify them.
What are Algebra Expressions?
Algebra expressions are mathematical statements that consist of variables, constants, and mathematical operations. They are used to represent unknown values or relationships between variables. Algebra expressions can be simple or complex, depending on the number of variables, constants, and operations involved.
Components of Algebra Expressions
Algebra expressions consist of three main components:
- Variables: These are letters or symbols that represent unknown values. Common variables used in algebra expressions include x, y, z, and a.
- Constants: These are numerical values that do not change. Examples of constants include 2, 5, and 10.
- Mathematical Operations: These are symbols that represent addition (+), subtraction (-), multiplication (×), and division (÷).
Types of Algebra Expressions
There are several types of algebra expressions, including:
- Monomials: These are expressions that consist of a single term. Examples include 2x and 5y.
- Binomials: These are expressions that consist of two terms. Examples include 2x + 3 and x^2 + 4.
- Polynomials: These are expressions that consist of multiple terms. Examples include 2x^2 + 3x - 4 and x^3 - 2x^2 + x + 1.
Simplifying Algebra Expressions
Simplifying algebra expressions involves combining like terms, removing parentheses, and rearranging terms to make the expression more manageable. Here are some steps to follow:
- Combine Like Terms: Combine terms that have the same variable and exponent. For example, 2x + 3x = 5x.
- Remove Parentheses: Remove parentheses by distributing the terms inside the parentheses to the terms outside. For example, 2(x + 3) = 2x + 6.
- Rearrange Terms: Rearrange terms to make the expression more manageable. For example, x^2 + 3x - 4 can be rearranged to x^2 + 3x - 4 = (x + 2)(x - 2).
Examples of Simplifying Algebra Expressions

| Expression | Simplified Expression |
|---|---|
| 2x + 3x | 5x |
| x^2 + 4x - 3 | x^2 + 4x - 3 = (x + 3)(x - 1) |
| 3(2x - 1) | 6x - 3 |
📝 Note: When simplifying algebra expressions, it is essential to follow the order of operations (PEMDAS) to ensure accuracy.
Practical Applications of Algebra Expressions
Algebra expressions have numerous practical applications in various fields, including:
- Science: Algebra expressions are used to describe the laws of physics, such as Newton’s laws of motion and the laws of thermodynamics.
- Engineering: Algebra expressions are used to design and optimize systems, such as electronic circuits and mechanical systems.
- Economics: Algebra expressions are used to model economic systems, such as supply and demand curves.
Conclusion
Algebra expressions are a fundamental concept in mathematics, and mastering them is crucial for students to succeed in various mathematical disciplines. By understanding the components of algebra expressions, simplifying them, and applying them to real-world problems, students can develop a deeper appreciation for the beauty and power of mathematics.
What is the difference between a variable and a constant in an algebra expression?
+A variable is a letter or symbol that represents an unknown value, while a constant is a numerical value that does not change.
How do I simplify an algebra expression?
+To simplify an algebra expression, combine like terms, remove parentheses, and rearrange terms to make the expression more manageable.
What are some practical applications of algebra expressions?
+Algebra expressions have numerous practical applications in various fields, including science, engineering, and economics.