5 Ways to Add Fractions With Same Denominator Easily

Understanding Fractions and Denominators

Fractions are a way to express a part of a whole as a ratio of two numbers. The bottom number, or denominator, tells us how many equal parts the whole is divided into, while the top number, or numerator, tells us how many of those parts we are considering. When adding fractions, it’s essential to have the same denominator to make the process easier. In this article, we will explore five simple ways to add fractions with the same denominator.

Why Same Denominator Matters

When adding fractions, having the same denominator simplifies the process. If the denominators are different, you would need to find the least common multiple (LCM) of the two denominators, which can be time-consuming. With the same denominator, you can directly add the numerators without worrying about finding the LCM.

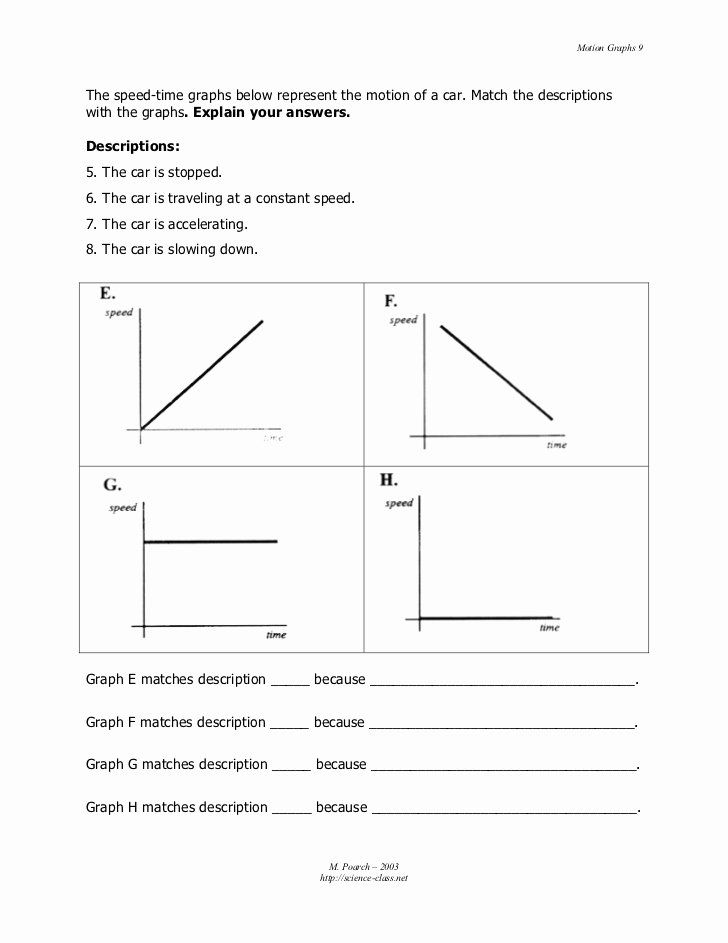
Method 1: Simple Addition

The simplest way to add fractions with the same denominator is to add the numerators directly. For example:

Fraction 1: ¹⁄₈ Fraction 2: ³⁄₈

To add these fractions, simply add the numerators:

1 + 3 = 4

The denominator remains the same, so the result is:

⁴⁄₈

You can simplify this fraction by dividing both the numerator and denominator by their greatest common divisor (GCD), which is 4.

4 ÷ 4 = 1 8 ÷ 4 = 2

So, the simplified result is:

¹⁄₂

🤔 Note: Always simplify your result to its lowest terms to make it easier to work with.

Method 2: Using Visual Aids

Visual aids like diagrams or blocks can help you understand the concept of adding fractions with the same denominator. Let’s consider the same example:

Fraction 1: ¹⁄₈ Fraction 2: ³⁄₈

Imagine you have a pizza divided into 8 equal slices. Fraction 1 represents 1 slice, and Fraction 2 represents 3 slices. To add these fractions, you can combine the slices:

1 slice + 3 slices = 4 slices

Since the pizza is still divided into 8 slices, the denominator remains the same. The result is:

⁴⁄₈

📝 Note: Visual aids can help you understand complex concepts, but make sure to practice the calculations to become more comfortable with the process.

Method 3: Using Number Lines

Number lines are another visual aid that can help you add fractions with the same denominator. Let’s consider the same example:

Fraction 1: ¹⁄₈ Fraction 2: ³⁄₈

Draw a number line with 8 equal parts, representing the denominator. Mark the numerator of each fraction on the number line:

Fraction 1: ¹⁄₈ Fraction 2: ³⁄₈

To add these fractions, move 1 unit from the starting point, then move another 3 units. The result will be:

⁴⁄₈

Number lines can help you visualize the process, but make sure to practice the calculations to become more comfortable with the process.

Method 4: Using Equivalent Ratios

Equivalent ratios can help you add fractions with the same denominator. Let’s consider the same example:

Fraction 1: ¹⁄₈ Fraction 2: ³⁄₈

You can rewrite each fraction as an equivalent ratio:

Fraction 1: ²⁄₁₆ Fraction 2: ⁶⁄₁₆

Since the denominators are the same, you can add the numerators:

2 + 6 = 8

The denominator remains the same, so the result is:

⁸⁄₁₆

You can simplify this fraction by dividing both the numerator and denominator by their GCD, which is 8.

8 ÷ 8 = 1 16 ÷ 8 = 2

So, the simplified result is:

¹⁄₂

📝 Note: Equivalent ratios can help you add fractions with different denominators, but in this case, we are focusing on same-denominator fractions.

Method 5: Using Real-World Examples

Real-world examples can help you understand the concept of adding fractions with the same denominator. Let’s consider a recipe that requires ¹⁄₄ cup of sugar and another recipe that requires ³⁄₄ cup of sugar. To add these fractions, you can simply add the numerators:

1 + 3 = 4

The denominator remains the same (cups), so the result is:

⁴⁄₄

You can simplify this fraction by dividing both the numerator and denominator by their GCD, which is 4.

4 ÷ 4 = 1 4 ÷ 4 = 1

So, the simplified result is:

¹⁄₁ or 1

📝 Note: Real-world examples can help you understand complex concepts, but make sure to practice the calculations to become more comfortable with the process.

To summarize, adding fractions with the same denominator is a straightforward process that requires simply adding the numerators. The five methods described above can help you understand and practice this concept.

In conclusion, adding fractions with the same denominator is a fundamental concept in mathematics that requires a simple yet logical approach. By mastering this concept, you can build a strong foundation for more complex mathematical operations.