Mastering Fractions: Adding and Subtracting Made Easy

Mastering Fractions: A Comprehensive Guide

Fractions are a fundamental concept in mathematics, and mastering them is essential for success in various mathematical operations, including adding and subtracting. In this article, we will delve into the world of fractions, exploring the basics, and providing a step-by-step guide on how to add and subtract fractions with ease.

Understanding Fractions

A fraction is a way to represent a part of a whole. It consists of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.

For example, in the fraction ³⁄₄, the numerator is 3, and the denominator is 4. This means we have 3 equal parts out of a total of 4 parts.

Types of Fractions

There are several types of fractions, including:

• Proper fractions: These are fractions where the numerator is less than the denominator. Example: ³⁄₄
• Improper fractions: These are fractions where the numerator is greater than or equal to the denominator. Example: ⁵⁄₄
• Mixed numbers: These are fractions that consist of a whole number and a proper fraction. Example: 2 ³⁄₄

Adding Fractions

Adding fractions is a straightforward process, but it requires a few simple steps:

1. Check the denominators: Make sure the denominators of both fractions are the same. If they are not, you will need to find the least common multiple (LCM) of both denominators.
2. Find the LCM: The LCM is the smallest number that both denominators can divide into evenly. For example, if the denominators are 4 and 6, the LCM would be 12.
3. Convert both fractions: Convert both fractions to have the LCM as the denominator.
4. Add the numerators: Add the numerators of both fractions.
5. Simplify the result: Simplify the result by dividing both the numerator and denominator by their greatest common divisor (GCD).

Example:

Add ¹⁄₄ and ¹⁄₆:

1. Check the denominators: 4 and 6 are not the same.
2. Find the LCM: The LCM of 4 and 6 is 12.
3. Convert both fractions: ¹⁄₄ = ³⁄₁₂ and ¹⁄₆ = ²⁄₁₂
4. Add the numerators: 3 + 2 = 5
5. Simplify the result: ⁵⁄₁₂

📝 Note: When adding fractions, make sure to simplify the result to its simplest form.

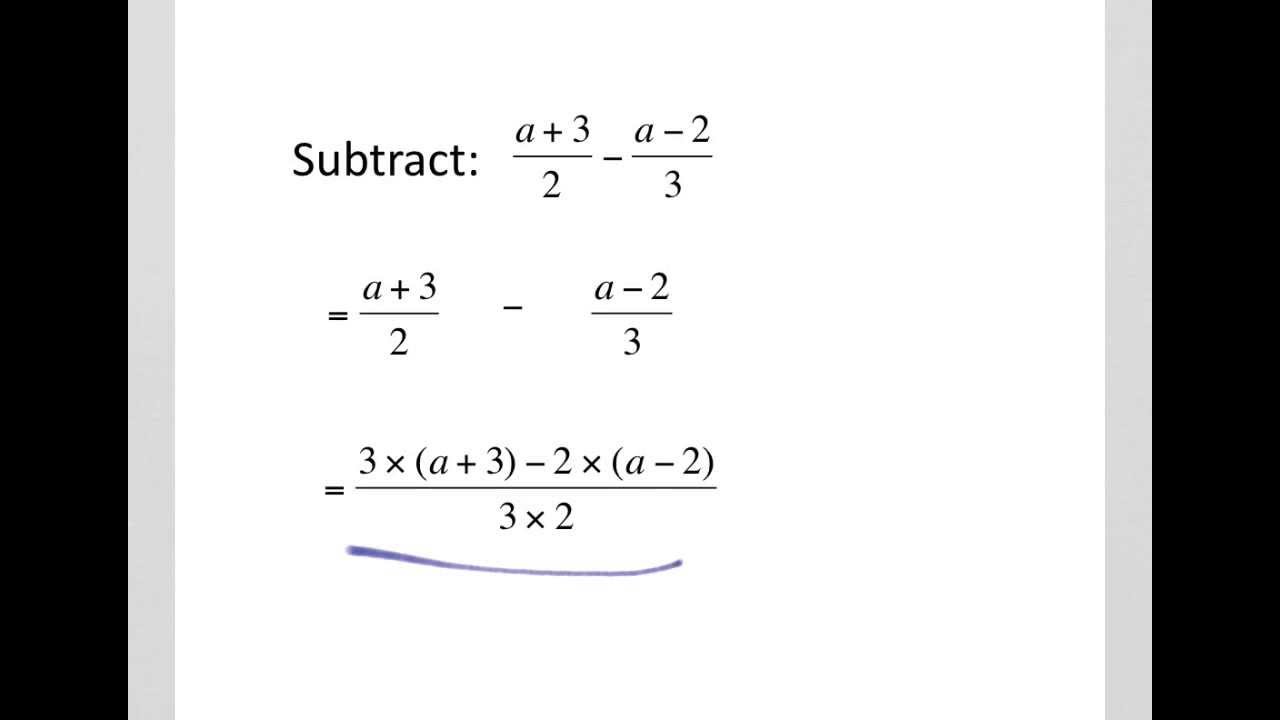
Subtracting Fractions

Subtracting fractions is similar to adding fractions, with a few simple steps:

1. Check the denominators: Make sure the denominators of both fractions are the same. If they are not, you will need to find the LCM of both denominators.
2. Find the LCM: The LCM is the smallest number that both denominators can divide into evenly.
3. Convert both fractions: Convert both fractions to have the LCM as the denominator.
4. Subtract the numerators: Subtract the numerators of both fractions.
5. Simplify the result: Simplify the result by dividing both the numerator and denominator by their GCD.

Example:

Subtract ¹⁄₄ from ¹⁄₆:

1. Check the denominators: 4 and 6 are not the same.
2. Find the LCM: The LCM of 4 and 6 is 12.
3. Convert both fractions: ¹⁄₄ = ³⁄₁₂ and ¹⁄₆ = ²⁄₁₂
4. Subtract the numerators: 2 - 3 = -1
5. Simplify the result: -¹⁄₁₂

📝 Note: When subtracting fractions, make sure to simplify the result to its simplest form.

Mixed Numbers and Improper Fractions

When working with mixed numbers and improper fractions, it’s essential to convert them to proper fractions before adding or subtracting.

Example:

Add 2 ³⁄₄ and 1 ¹⁄₂:

1. Convert both mixed numbers to improper fractions: 2 ³⁄₄ = ¹¹⁄₄ and 1 ¹⁄₂ = ³⁄₂
2. Find the LCM: The LCM of 4 and 2 is 4.
3. Convert both fractions: ¹¹⁄₄ = ¹¹⁄₄ and ³⁄₂ = ⁶⁄₄
4. Add the numerators: 11 + 6 = 17
5. Simplify the result: ¹⁷⁄₄ = 4 ¹⁄₄

Real-World Applications

Fractions have numerous real-world applications, including:

• Cooking: Measuring ingredients for recipes
• Shopping: Comparing prices and discounts
• Building: Measuring materials and calculating quantities

Conclusion

Mastering fractions is an essential skill for success in mathematics and various real-world applications. By following the simple steps outlined in this article, you can add and subtract fractions with ease. Remember to always simplify your results and convert mixed numbers and improper fractions to proper fractions before performing operations.