10 Essential Facts About 45-45-90 Special Right Triangles

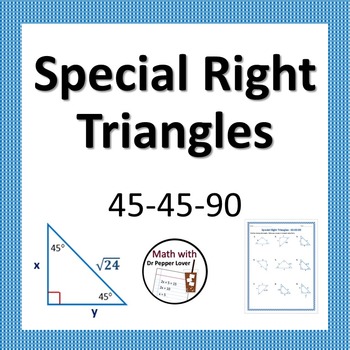
Understanding the Basics of 45-45-90 Special Right Triangles

In geometry, special right triangles are a fundamental concept that helps students and mathematicians alike to solve complex problems with ease. Among these, the 45-45-90 triangle is one of the most commonly encountered and useful special right triangles. This triangle is characterized by its unique property of having two sides of equal length, which are also the legs of the triangle, and a hypotenuse that is √2 times the length of each leg.

What are 45-45-90 Triangles?

A 45-45-90 triangle is a special right triangle with two acute angles of 45 degrees each and a right angle of 90 degrees. This triangle is also known as an isosceles right triangle, due to the two sides being of equal length. The two legs of the triangle are the sides that meet at a right angle, while the hypotenuse is the side opposite the right angle.

Properties of 45-45-90 Triangles

There are several key properties of 45-45-90 triangles that make them useful in a wide range of mathematical applications. These include:

• The two legs of the triangle are of equal length.
• The hypotenuse is √2 times the length of each leg.
• The triangle is isosceles, meaning that the two legs are congruent.
• The triangle is a right triangle, meaning that one angle is 90 degrees.

Identifying 45-45-90 Triangles

Identifying 45-45-90 triangles can be done by looking for the following characteristics:

• Two sides of equal length
• A right angle of 90 degrees
• Two acute angles of 45 degrees each

Real-World Applications of 45-45-90 Triangles

45-45-90 triangles have a wide range of real-world applications, including:

• Building design: 45-45-90 triangles are often used in building design to create right angles and ensure stability.
• Physics and engineering: 45-45-90 triangles are used to describe the motion of objects and forces in physics and engineering.
• Computer graphics: 45-45-90 triangles are used in computer graphics to create 3D models and animations.

Examples of 45-45-90 Triangles

Here are a few examples of 45-45-90 triangles:

• A square with two adjacent sides extended to create a right triangle with two acute angles of 45 degrees each.
• A rectangle with a diagonal that creates a right triangle with two acute angles of 45 degrees each.

How to Solve 45-45-90 Triangles

Solving 45-45-90 triangles involves using the properties of the triangle to find the length of the sides. Here are the steps:

1. Identify the length of one leg of the triangle.
2. Use the property that the hypotenuse is √2 times the length of each leg to find the length of the hypotenuse.
3. Use the property that the two legs are of equal length to find the length of the other leg.

🤔 Note: The length of the hypotenuse can also be found using the Pythagorean theorem, which states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs.

Common Mistakes to Avoid

When working with 45-45-90 triangles, there are several common mistakes to avoid:

• Forgetting that the hypotenuse is √2 times the length of each leg. This property is unique to 45-45-90 triangles and is essential for solving these triangles.
• Confusing the legs and hypotenuse. Make sure to identify the legs and hypotenuse correctly to avoid mistakes.
• Not checking the angles. Make sure to check that the triangle has two acute angles of 45 degrees each and a right angle of 90 degrees.

Conclusion

In conclusion, 45-45-90 triangles are a fundamental concept in geometry and have a wide range of real-world applications. By understanding the properties and characteristics of these triangles, you can solve complex problems with ease. Remember to avoid common mistakes and always check your work to ensure accuracy.

Related Terms:

• 30-60-90 and 45-45-90 triangle worksheet