Dilations and Scale Factors Worksheet Answers and Solutions
Understanding Dilations and Scale Factors
Dilations are a fundamental concept in geometry, involving the transformation of a figure by enlarging or shrinking it with respect to a fixed point. This process involves using a scale factor, which determines the extent of the transformation. In this blog post, we’ll delve into the world of dilations and scale factors, providing you with a comprehensive worksheet and answers to reinforce your understanding.
What are Dilations?
A dilation is a transformation that changes the size of a figure, but not its shape. It’s like zooming in or out of a picture, where the image gets larger or smaller, but its proportions remain the same. Dilations can be either enlargements (making the figure bigger) or reductions (making the figure smaller).
What is a Scale Factor?
The scale factor is a ratio that determines how much a figure is enlarged or reduced during a dilation. It’s usually represented by a number, which tells you how many times larger or smaller the new figure will be compared to the original. For example, if you have a scale factor of 2, the new figure will be twice as large as the original.
Types of Scale Factors
There are two main types of scale factors:
- Enlargement scale factor: Greater than 1 (e.g., 2, 3, 4, etc.)
- Reduction scale factor: Less than 1 (e.g., 1⁄2, 1⁄3, 1⁄4, etc.)
How to Perform a Dilation
To perform a dilation, you need to follow these steps:
- Identify the center of dilation (the fixed point).
- Determine the scale factor.
- Measure the distance from the center of dilation to each vertex of the original figure.
- Multiply each distance by the scale factor.
- Plot the new vertices using the scaled distances.
📝 Note: If the scale factor is greater than 1, the figure will be enlarged. If it's less than 1, the figure will be reduced.
Worksheet Answers and Solutions
Here are some sample problems to help you practice dilations and scale factors:
Problem 1: A triangle has vertices at (0, 0), (3, 0), and (0, 4). Perform a dilation with a scale factor of 2 and a center of dilation at (0, 0).
Solution:
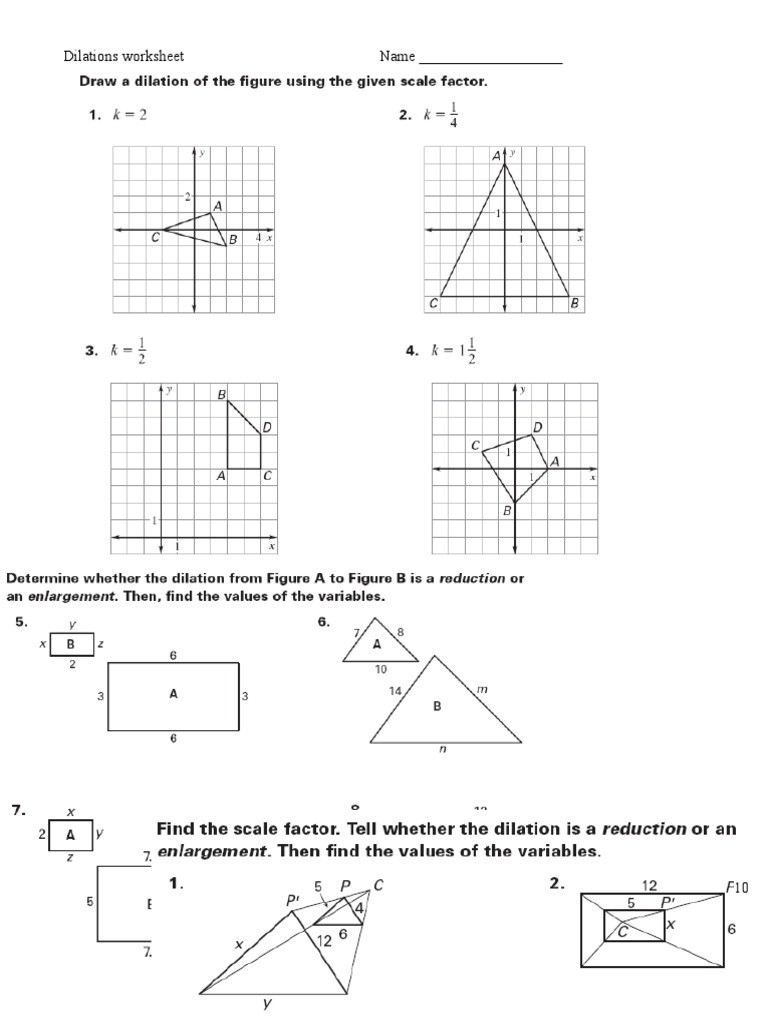
| Vertex | Original Coordinates | Scaled Coordinates |
|---|---|---|
| A | (0, 0) | (0, 0) |
| B | (3, 0) | (6, 0) |
| C | (0, 4) | (0, 8) |
Problem 2: A rectangle has vertices at (2, 2), (6, 2), (6, 6), and (2, 6). Perform a dilation with a scale factor of 1⁄2 and a center of dilation at (4, 4).
Solution:
| Vertex | Original Coordinates | Scaled Coordinates |
|---|---|---|
| A | (2, 2) | (3, 3) |
| B | (6, 2) | (5, 3) |
| C | (6, 6) | (5, 5) |
| D | (2, 6) | (3, 5) |
Problem 3: A circle has a radius of 4 units and a center at (0, 0). Perform a dilation with a scale factor of 3 and a center of dilation at (0, 0).
Solution:
The radius of the new circle will be 3 times the original radius: 4 × 3 = 12 units.
Conclusion
Dilations and scale factors are fundamental concepts in geometry, allowing you to transform figures by enlarging or shrinking them. By understanding how to perform dilations and using scale factors, you’ll be able to solve a wide range of problems in mathematics and real-world applications.
What is the difference between a dilation and a translation?
+A dilation changes the size of a figure, while a translation moves the figure to a new location without changing its size or shape.
Can a scale factor be negative?
+No, a scale factor cannot be negative. If you multiply a distance by a negative number, you’ll change its direction, not its magnitude.
How do you determine the center of dilation?
+The center of dilation is usually given in the problem or can be inferred from the context. It’s the fixed point around which the dilation occurs.
Related Terms:
- Scale factor worksheet answer key
- 4.5 dilations answer key
- Scale factor Worksheet PDF
- Identifying scale factors
- Translation and dilation Worksheet