Parabolas Worksheets: Master the Concept with Easy Practice
Understanding Parabolas: A Comprehensive Guide
Parabolas are a fundamental concept in mathematics, particularly in algebra and geometry. They are U-shaped curves that are essential in understanding various real-world phenomena, such as the trajectory of projectiles, satellite dishes, and even the shape of a suspension bridge. Mastering parabolas requires a solid grasp of their properties, equations, and applications. In this article, we will delve into the world of parabolas, exploring their definition, types, equations, and practice exercises to help you become proficient in this concept.
What is a Parabola?
A parabola is a quadratic curve that is U-shaped and symmetrical about its axis. It is defined as the set of all points that are equidistant from a fixed point, known as the focus, and a fixed line, known as the directrix. The parabola’s shape is determined by the distance between the focus and the directrix. Parabolas can be oriented in various ways, including upwards, downwards, leftwards, and rightwards.
Types of Parabolas
There are several types of parabolas, including:
- Vertical Parabolas: These parabolas are oriented upwards or downwards and have an equation of the form y = ax^2 + bx + c.
- Horizontal Parabolas: These parabolas are oriented leftwards or rightwards and have an equation of the form x = ay^2 + by + c.
- Sideways Parabolas: These parabolas are oriented at an angle and have an equation of the form y = a(x - h)^2 + k, where (h, k) is the vertex.
Equations of Parabolas
The standard equation of a parabola is:
y = ax^2 + bx + c
where a, b, and c are constants, and a ≠ 0. The value of a determines the parabola’s shape and orientation. If a > 0, the parabola opens upwards; if a < 0, it opens downwards.
Key Properties of Parabolas
Here are some essential properties of parabolas:
- Vertex: The vertex is the lowest or highest point on the parabola, depending on its orientation.
- Axis of Symmetry: The axis of symmetry is the line that passes through the vertex and is perpendicular to the directrix.
- Focus: The focus is a fixed point that is equidistant from the vertex and the directrix.
- Directrix: The directrix is a fixed line that is perpendicular to the axis of symmetry.
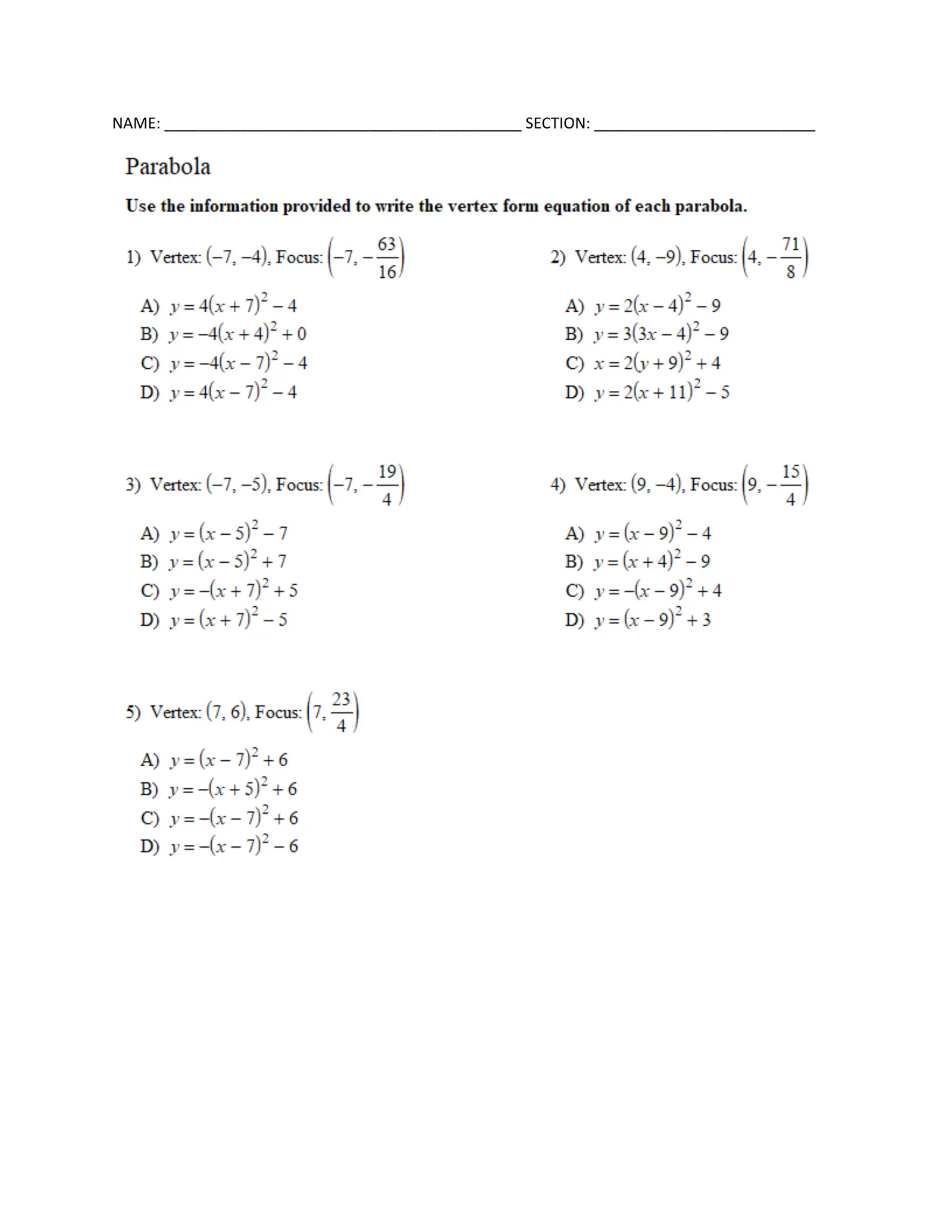
Practice Exercises
To reinforce your understanding of parabolas, try the following exercises:
- Find the equation of the parabola with vertex (2, 3) and axis of symmetry x = 2.
- Determine the focus and directrix of the parabola y = x^2 + 2x + 1.
- Sketch the graph of the parabola x = y^2 - 4y + 3.
📝 Note: For more practice exercises and worksheets, refer to the resources section at the end of this article.
Real-World Applications of Parabolas
Parabolas have numerous real-world applications, including:
- Projectile Motion: The trajectory of a projectile, such as a thrown ball or a launched rocket, follows a parabolic path.
- Satellite Dishes: Satellite dishes are shaped like parabolas to focus incoming signals onto a single point.
- Suspension Bridges: The cables of a suspension bridge form a parabolic shape to distribute the weight evenly.
Conclusion
Parabolas are a fundamental concept in mathematics, and mastering them requires a solid grasp of their properties, equations, and applications. With practice and patience, you can become proficient in working with parabolas and appreciate their significance in real-world phenomena.
What is the standard equation of a parabola?
+
The standard equation of a parabola is y = ax^2 + bx + c, where a, b, and c are constants, and a ≠ 0.
What is the axis of symmetry of a parabola?
+
The axis of symmetry is the line that passes through the vertex and is perpendicular to the directrix.
What is the focus of a parabola?
+
The focus is a fixed point that is equidistant from the vertex and the directrix.