Worksheet On Simplifying Rational Expressions

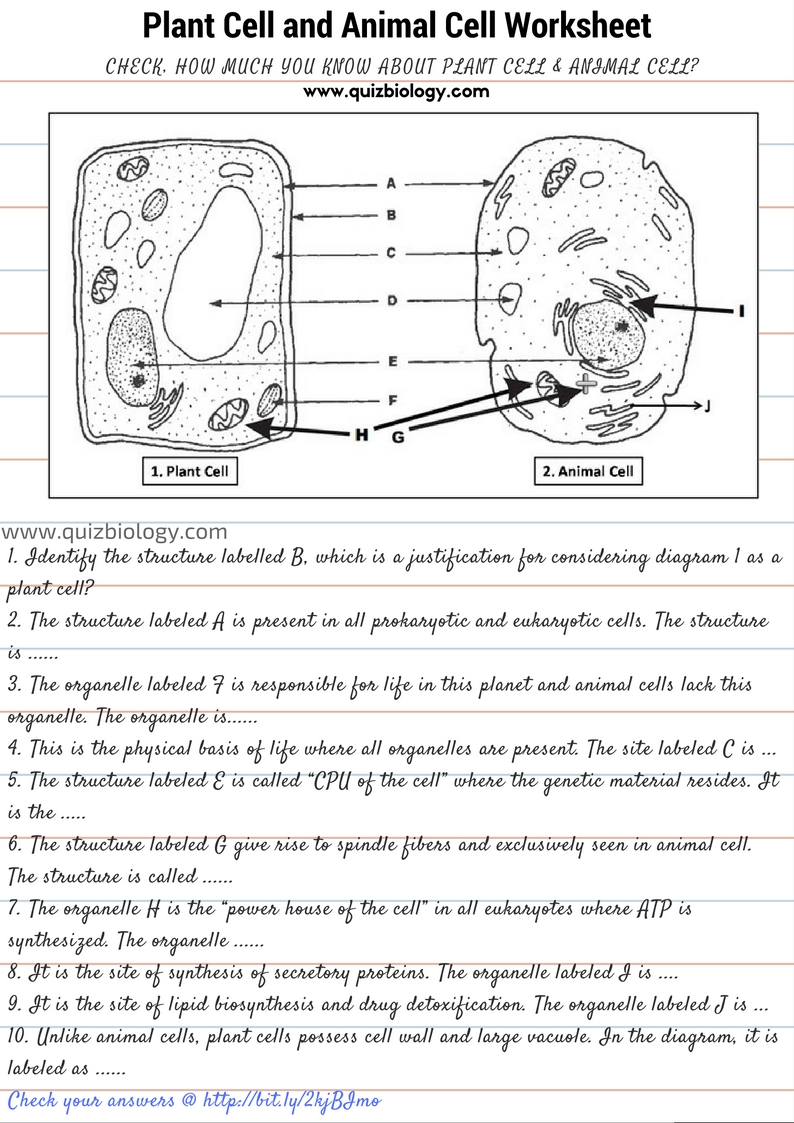
Understanding Rational Expressions

Rational expressions are fractions that contain polynomials in both the numerator and denominator. To simplify these expressions, we need to factor the polynomials and cancel out any common factors. This process is crucial in algebra and is used extensively in various mathematical operations.

Factors and Greatest Common Factors (GCF)

Before we dive into simplifying rational expressions, let’s quickly review factors and greatest common factors (GCF).

Factors: A factor is a polynomial that divides another polynomial exactly without leaving a remainder.

Greatest Common Factor (GCF): The GCF of two or more polynomials is the largest polynomial that divides each of them exactly without leaving a remainder.

To find the GCF of two polynomials, we need to list the factors of each polynomial and identify the largest common factor.

Simplifying Rational Expressions

Now that we have reviewed factors and GCF, let’s move on to simplifying rational expressions.

Step 1: Factor the numerator and denominator

To simplify a rational expression, we need to factor the numerator and denominator. This will help us identify any common factors that can be canceled out.

Step 2: Identify common factors

Once we have factored the numerator and denominator, we need to identify any common factors. These are factors that appear in both the numerator and denominator.

Step 3: Cancel out common factors

If we have identified any common factors, we can cancel them out. This will simplify the rational expression.

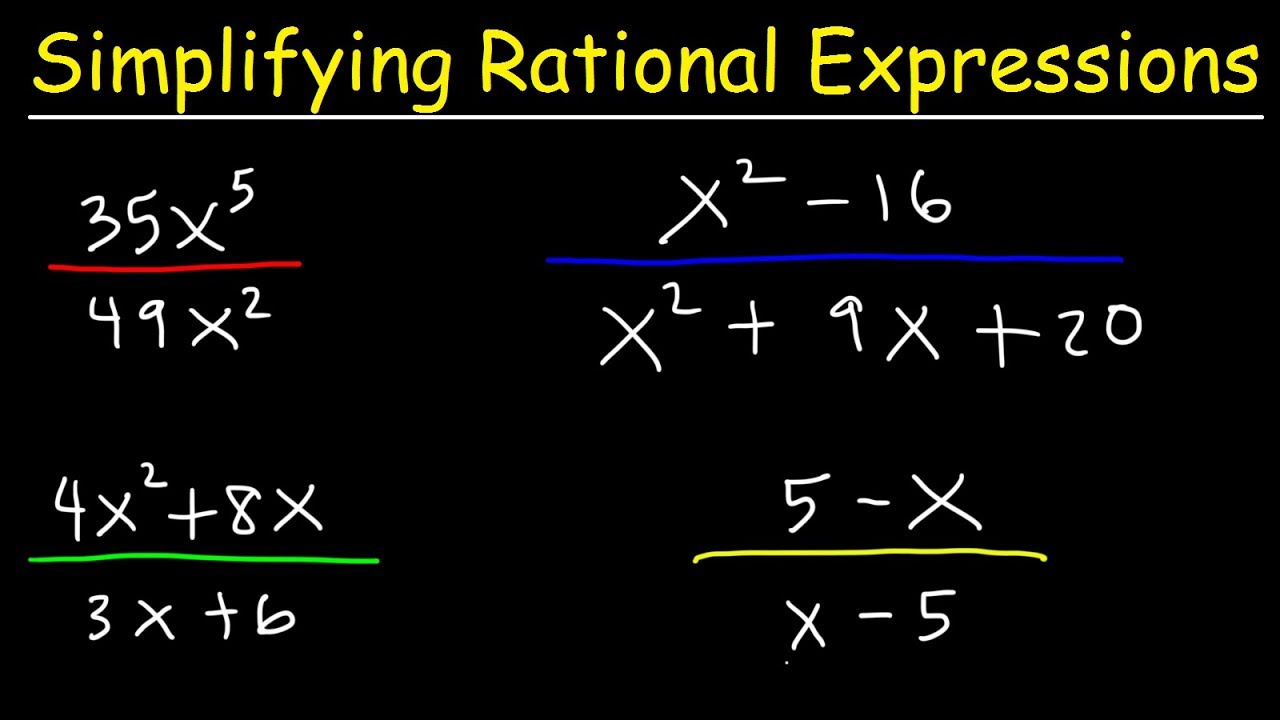
Example 1: Simplify the rational expression

To simplify this rational expression, we can factor the numerator and denominator:

We can see that there are no common factors between the numerator and denominator, so the rational expression is already simplified.

Example 2: Simplify the rational expression

To simplify this rational expression, we can factor the numerator and denominator:

In this example, we were able to cancel out the common factor $x+3$ from the numerator and denominator.

Notes

💡 Note: When simplifying rational expressions, it's essential to check for any restrictions on the domain. For example, if the denominator is zero, the expression is undefined.

💡 Note: To simplify a rational expression, we need to factor the numerator and denominator. If there are any common factors, we can cancel them out.

Conclusion

Simplifying rational expressions is an essential skill in algebra. By factoring the numerator and denominator and canceling out any common factors, we can simplify complex rational expressions. Remember to always check for any restrictions on the domain and to factor the numerator and denominator before simplifying.