Understanding Negative Exponents
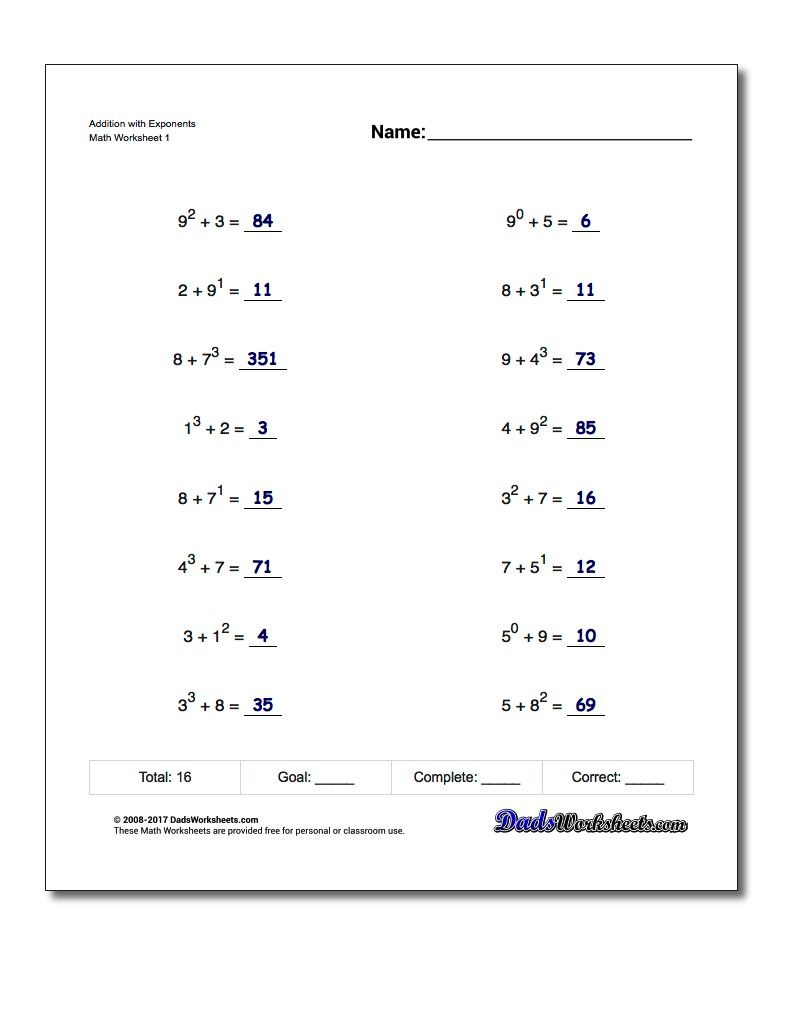
Negative exponents can seem daunting at first, but with a solid understanding of the concept, you’ll be able to tackle even the most complex problems with ease. So, what exactly are negative exponents? In simple terms, a negative exponent is a way of expressing a fraction as a power of the base number. For instance, 2^(-3) is equivalent to 1⁄2^3.
When dealing with negative exponents, it’s essential to remember the following rules:
- a^(-n) = 1/a^n: This rule allows you to rewrite a negative exponent as a positive exponent by flipping the base and changing the sign.
- a^(-n) = (1/a)^n: This rule is a variation of the previous one, where you can rewrite the negative exponent as a power of the reciprocal of the base.
How to Simplify Negative Exponents
Now that you know the rules, let’s dive into some examples to help you simplify negative exponents.
- 2^(-4): Using the first rule, you can rewrite this as 1⁄2^4, which equals 1⁄16.
- (3⁄4)^(-2): Applying the second rule, you can rewrite this as (4⁄3)^2, which equals 16⁄9.
Worksheet Help
Here’s a simple worksheet to help you practice simplifying negative exponents:
| Expression | Simplified Form |
|---|---|
| x^(-2) | ? |
| (2/3)^(-3) | ? |
| 5^(-1) | ? |
| (y/2)^(-4) | ? |
Answers:
- x^(-2) = 1/x^2
- (2⁄3)^(-3) = (3⁄2)^3 = 27⁄8
- 5^(-1) = 1⁄5
- (y/2)^(-4) = (2/y)^4 = 16/y^4
More Practice with Negative Exponents
For more practice, try simplifying the following expressions:
- (2x)^(-3)
- (5⁄2)^(-2)
- y^(-5)
- (3/x)^(-2)
Common Mistakes to Avoid
When working with negative exponents, it’s easy to get confused. Here are some common mistakes to avoid:
- Don’t confuse negative exponents with negative numbers: A negative exponent is not the same as a negative number. For example, 2^(-3) is not the same as -2^3.
- Don’t forget to flip the base: When rewriting a negative exponent as a positive exponent, don’t forget to flip the base. For instance, 2^(-3) becomes 1⁄2^3, not 2^3.
Conclusion
Mastering negative exponents takes practice, but with these simple rules and examples, you’ll be well on your way to becoming a pro. Remember to always follow the rules, practice regularly, and avoid common mistakes.
What is the difference between a negative exponent and a negative number?
+A negative exponent is a way of expressing a fraction as a power of the base number, whereas a negative number is simply a number less than zero.
How do I simplify a negative exponent?
+To simplify a negative exponent, you can use the rules a^(-n) = 1/a^n or a^(-n) = (1/a)^n.
What are some common mistakes to avoid when working with negative exponents?
+Common mistakes include confusing negative exponents with negative numbers and forgetting to flip the base when rewriting a negative exponent as a positive exponent.
