5 Ways to Master Laws of Exponents
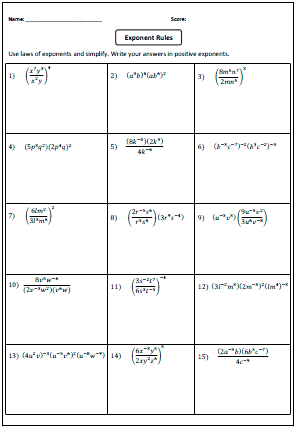
Mastering the Laws of Exponents: A Comprehensive Guide
The laws of exponents are a fundamental concept in mathematics, and understanding them is crucial for success in various mathematical disciplines, including algebra, geometry, and calculus. In this article, we will delve into the world of exponents and explore five ways to master the laws of exponents.
Understanding the Basics
Before we dive into the laws of exponents, it’s essential to understand the basics of exponents. An exponent is a small number that is raised to a power, indicating how many times the base number should be multiplied by itself. For example, in the expression 2^3, 2 is the base, and 3 is the exponent.
Key Concepts:
- Base: The number being raised to a power.
- Exponent: The small number that indicates the power to which the base is raised.
- Power: The result of raising the base to the exponent.
Law 1: The Product of Powers Law
The product of powers law states that when multiplying two or more numbers with the same base, you can add the exponents. This law is represented by the equation:
a^m × a^n = a^(m+n)
For example:
2^2 × 2^3 = 2^(2+3) = 2^5
💡 Note: This law only applies when the bases are the same.
Law 2: The Power of a Power Law
The power of a power law states that when raising a number to a power, and then raising that result to another power, you can multiply the exponents. This law is represented by the equation:
(a^m)^n = a^(m×n)
For example:
(2^2)^3 = 2^(2×3) = 2^6
🔍 Note: This law only applies when the bases are the same.
Law 3: The Power of a Product Law
The power of a product law states that when raising a product of numbers to a power, you can raise each number to that power and then multiply the results. This law is represented by the equation:
(ab)^n = a^n × b^n
For example:
(2 × 3)^2 = 2^2 × 3^2 = 4 × 9 = 36
💡 Note: This law only applies when the numbers are multiplied together.
Law 4: The Negative Exponent Law
The negative exponent law states that when a number has a negative exponent, you can rewrite it as the reciprocal of the number with a positive exponent. This law is represented by the equation:
a^(-n) = 1/a^n
For example:
2^(-3) = 1⁄2^3 = 1⁄8
🔍 Note: This law only applies when the exponent is negative.
Law 5: The Zero Exponent Law
The zero exponent law states that when a number has a zero exponent, the result is always 1. This law is represented by the equation:
a^0 = 1
For example:
2^0 = 1
💡 Note: This law applies to all numbers, except zero.
Conclusion
Mastering the laws of exponents requires practice, patience, and persistence. By understanding the five laws outlined in this article, you’ll be well on your way to becoming an exponent expert. Remember to apply these laws to various mathematical problems, and don’t be afraid to ask for help when needed. With time and practice, you’ll become proficient in using the laws of exponents to solve complex mathematical problems.
What is the product of powers law?
+The product of powers law states that when multiplying two or more numbers with the same base, you can add the exponents.
What is the power of a power law?
+The power of a power law states that when raising a number to a power, and then raising that result to another power, you can multiply the exponents.
What is the negative exponent law?
+The negative exponent law states that when a number has a negative exponent, you can rewrite it as the reciprocal of the number with a positive exponent.
Related Terms:
- Exponent Worksheet
- Exponent exercises pdf
- Exponent pdf
- Exponent grade 10