Graphing Quadratics from Standard Form Worksheet Solutions
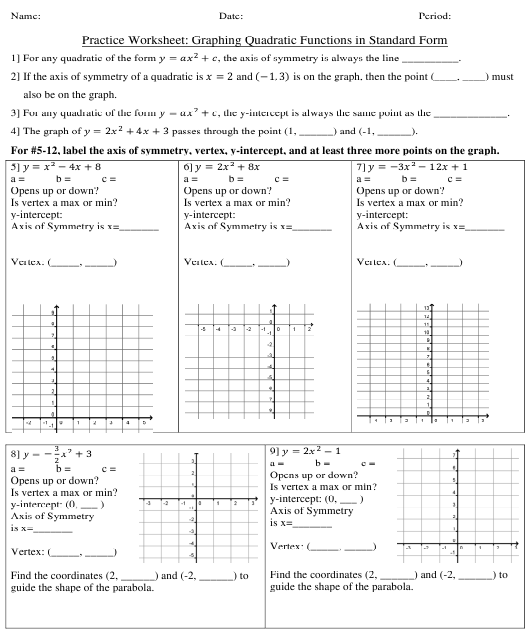
Understanding Quadratic Equations in Standard Form
Quadratic equations are a fundamental concept in algebra, and understanding how to graph them is crucial for problem-solving and critical thinking. In this post, we will delve into the world of quadratic equations in standard form and explore how to graph them using a step-by-step approach.
What is the Standard Form of a Quadratic Equation?
The standard form of a quadratic equation is ax^2 + bx + c = 0, where:
- a is the coefficient of the x^2 term (cannot be zero)
- b is the coefficient of the x term
- c is the constant term
How to Graph Quadratic Equations in Standard Form
Graphing quadratic equations in standard form involves several steps:
- Identify the coefficients: Determine the values of a, b, and c in the quadratic equation.
- Determine the direction of the parabola: If a > 0, the parabola opens upward. If a < 0, the parabola opens downward.
- Find the vertex: The vertex of the parabola is the lowest or highest point on the graph. The x-coordinate of the vertex can be found using the formula x = -b / 2a.
- Find the y-intercept: The y-intercept is the point where the parabola crosses the y-axis. The y-intercept can be found by substituting x = 0 into the equation.
- Plot additional points: Plot additional points on either side of the vertex to determine the shape of the parabola.
📝 Note: Make sure to label the x-axis and y-axis when graphing the parabola.
Example 1: Graphing a Quadratic Equation in Standard Form
Graph the quadratic equation x^2 + 4x + 4 = 0.
- Identify the coefficients: a = 1, b = 4, and c = 4.
- Determine the direction of the parabola: Since a > 0, the parabola opens upward.
- Find the vertex: The x-coordinate of the vertex is x = -4 / (2*1) = -2.
- Find the y-intercept: Substitute x = 0 into the equation to get y = 4.
- Plot additional points: Plot points on either side of the vertex to determine the shape of the parabola.
Example 2: Graphing a Quadratic Equation in Standard Form
Graph the quadratic equation -x^2 + 3x - 2 = 0.
- Identify the coefficients: a = -1, b = 3, and c = -2.
- Determine the direction of the parabola: Since a < 0, the parabola opens downward.
- Find the vertex: The x-coordinate of the vertex is x = -3 / (2*-1) = 3⁄2.
- Find the y-intercept: Substitute x = 0 into the equation to get y = -2.
- Plot additional points: Plot points on either side of the vertex to determine the shape of the parabola.
Common Mistakes to Avoid When Graphing Quadratic Equations
- Forgetting to label the x-axis and y-axis
- Failing to determine the direction of the parabola
- Not finding the vertex and y-intercept correctly
- Not plotting additional points to determine the shape of the parabola
🚨 Note: Always double-check your work to ensure accuracy.
Conclusion
Graphing quadratic equations in standard form requires attention to detail and a step-by-step approach. By following the steps outlined in this post, you can master the art of graphing quadratic equations and tackle more complex problems with confidence.
What is the standard form of a quadratic equation?
+The standard form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants and a cannot be zero.
How do I determine the direction of the parabola?
+If a > 0, the parabola opens upward. If a < 0, the parabola opens downward.
What is the vertex of a parabola?
+The vertex is the lowest or highest point on the graph of the parabola. The x-coordinate of the vertex can be found using the formula x = -b / 2a.