6 Ways to Find Volume of Triangular Prism
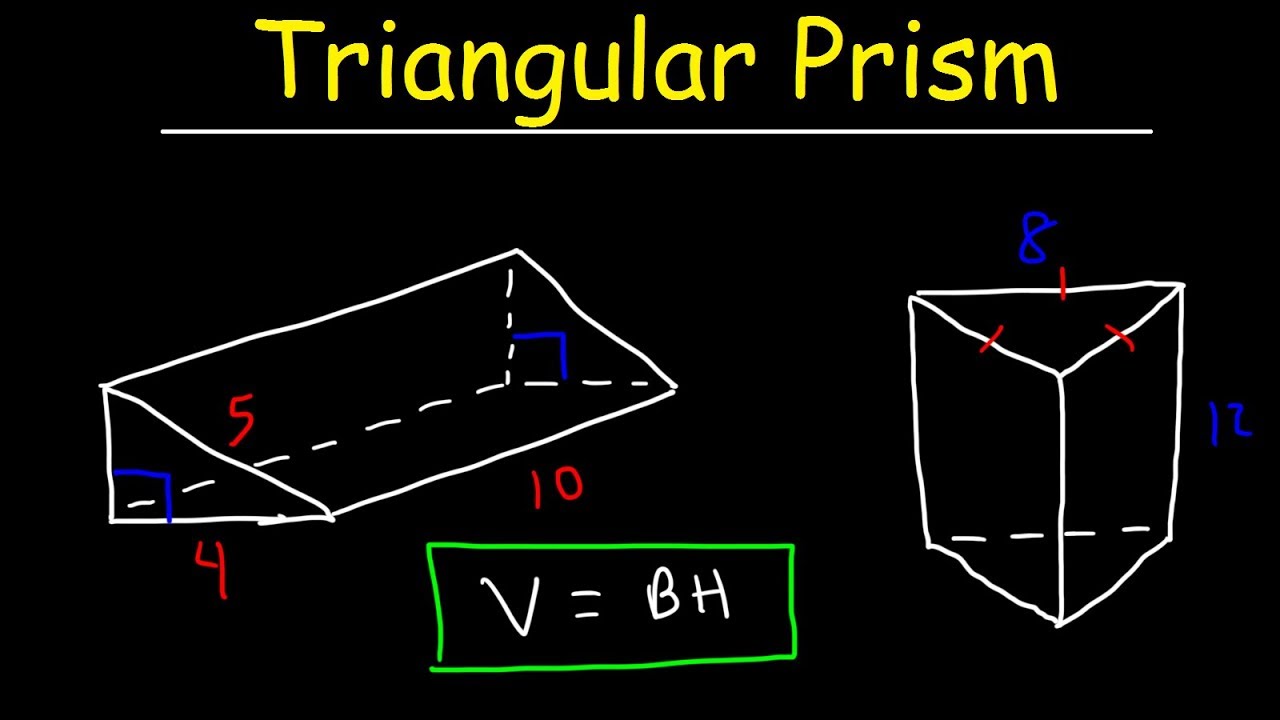
The triangular prism is a three-dimensional shape that has two identical faces that are triangles, and three rectangular faces that connect the corresponding sides of the triangles. Finding the volume of a triangular prism can be a bit tricky, but don’t worry, we’ve got you covered. In this article, we’ll explore six different ways to find the volume of a triangular prism.
Method 1: Using the Formula V = (1/2) × Base × Height × Length
The most straightforward way to find the volume of a triangular prism is by using the formula V = (1⁄2) × Base × Height × Length. This formula is similar to the formula for the area of a triangle, but with an additional dimension.
- Base: The base of the prism is the length of one of the triangular faces.
- Height: The height of the prism is the distance between the two triangular faces.
- Length: The length of the prism is the distance between the two rectangular faces that connect the corresponding sides of the triangles.
By plugging in the values for base, height, and length, you can easily calculate the volume of the triangular prism.
Method 2: Using the Formula V = Area of Base × Height
Another way to find the volume of a triangular prism is by using the formula V = Area of Base × Height. This formula is similar to the formula for the volume of a rectangular prism, but with a triangular base instead.
- Area of Base: The area of the base is the area of one of the triangular faces. This can be calculated using the formula for the area of a triangle, which is (1⁄2) × Base × Height.
- Height: The height of the prism is the distance between the two triangular faces.
By plugging in the values for area of base and height, you can easily calculate the volume of the triangular prism.
Method 3: Using the Formula V = (1/3) × Base × Height × Length (for a Right Triangular Prism)
If the triangular prism is a right triangular prism, meaning that the two rectangular faces that connect the corresponding sides of the triangles are perpendicular to each other, then you can use the formula V = (1⁄3) × Base × Height × Length.
- Base: The base of the prism is the length of one of the triangular faces.
- Height: The height of the prism is the distance between the two triangular faces.
- Length: The length of the prism is the distance between the two rectangular faces that connect the corresponding sides of the triangles.
By plugging in the values for base, height, and length, you can easily calculate the volume of the right triangular prism.
Method 4: Using the Formula V = (1/2) × Base1 × Base2 × Height (for an Isosceles Triangular Prism)
If the triangular prism is an isosceles triangular prism, meaning that the two triangular faces are congruent, then you can use the formula V = (1⁄2) × Base1 × Base2 × Height.
- Base1: The base of one of the triangular faces.
- Base2: The base of the other triangular face.
- Height: The height of the prism is the distance between the two triangular faces.
By plugging in the values for base1, base2, and height, you can easily calculate the volume of the isosceles triangular prism.
Method 5: Using the Formula V = (1/2) × Base × Height × Length (for a Scalene Triangular Prism)
If the triangular prism is a scalene triangular prism, meaning that the two triangular faces are not congruent, then you can use the formula V = (1⁄2) × Base × Height × Length.
- Base: The base of one of the triangular faces.
- Height: The height of the prism is the distance between the two triangular faces.
- Length: The length of the prism is the distance between the two rectangular faces that connect the corresponding sides of the triangles.
By plugging in the values for base, height, and length, you can easily calculate the volume of the scalene triangular prism.
Method 6: Using the Formula V = (1/2) × Area of Base × Length (for a Triangular Prism with a Non-Right Angle)
If the triangular prism has a non-right angle, meaning that the two rectangular faces that connect the corresponding sides of the triangles are not perpendicular to each other, then you can use the formula V = (1⁄2) × Area of Base × Length.
- Area of Base: The area of one of the triangular faces. This can be calculated using the formula for the area of a triangle, which is (1⁄2) × Base × Height.
- Length: The length of the prism is the distance between the two rectangular faces that connect the corresponding sides of the triangles.
By plugging in the values for area of base and length, you can easily calculate the volume of the triangular prism.
By now, you should be able to find the volume of a triangular prism using any of these six methods.
🤔 Note: Make sure to choose the correct method based on the specific characteristics of the triangular prism you are working with.
When it comes to finding the volume of a triangular prism, there are many different methods to choose from. By using one of the six methods outlined in this article, you should be able to easily calculate the volume of any triangular prism.
What is the formula for the volume of a triangular prism?
+
The formula for the volume of a triangular prism is V = (1⁄2) × Base × Height × Length.
How do I find the volume of a right triangular prism?
+
The formula for the volume of a right triangular prism is V = (1⁄3) × Base × Height × Length.
What is the difference between a right triangular prism and an isosceles triangular prism?
+
A right triangular prism has two rectangular faces that connect the corresponding sides of the triangles, which are perpendicular to each other. An isosceles triangular prism has two triangular faces that are congruent.