5 Ways to Master Volume of a Sphere
Understanding the Volume of a Sphere
The volume of a sphere is a fundamental concept in mathematics, particularly in geometry. It is used to calculate the amount of space inside a sphere. The formula for the volume of a sphere is (4⁄3)πr^3, where r is the radius of the sphere. However, mastering the volume of a sphere requires more than just memorizing the formula. It involves understanding the concept, applying it to real-life problems, and practicing with different examples.
1. Understand the Concept
To master the volume of a sphere, it is essential to understand the concept behind it. A sphere is a three-dimensional shape that is perfectly round. It has no beginning or end, and every point on the surface is equidistant from the center. The volume of a sphere is the amount of space inside the sphere, and it is calculated using the formula (4⁄3)πr^3.
Key Points to Remember:
- The volume of a sphere is directly proportional to the cube of its radius.
- The volume of a sphere is independent of its surface area.
- The volume of a sphere can be used to calculate the volume of other shapes, such as cylinders and cones.
2. Practice with Different Examples
Practicing with different examples is an excellent way to master the volume of a sphere. Here are a few examples to get you started:
- Find the volume of a sphere with a radius of 4 cm.
- Find the volume of a sphere with a diameter of 10 cm.
- Find the volume of a sphere with a circumference of 20 cm.
Step-by-Step Solution:
- Find the radius of the sphere (if not given).
- Plug the value of the radius into the formula (4⁄3)πr^3.
- Calculate the volume using the formula.
📝 Note: Use π = 3.14 for calculation purposes.
3. Apply to Real-Life Problems
The volume of a sphere has numerous real-life applications. Here are a few examples:
- Calculating the volume of a basketball or a soccer ball.
- Calculating the volume of a planet or a moon.
- Calculating the volume of a water tank or a container.
Real-Life Example:
- A water tank is in the shape of a sphere with a diameter of 10 meters. Calculate the volume of the water tank.
Step-by-Step Solution:
- Find the radius of the sphere (diameter/2).
- Plug the value of the radius into the formula (4⁄3)πr^3.
- Calculate the volume using the formula.
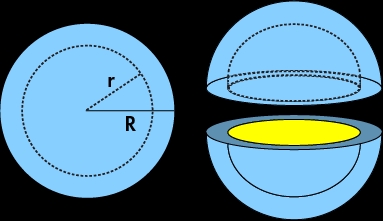
4. Use Visual Aids
Visual aids such as diagrams and illustrations can help you understand the concept of the volume of a sphere better. Here is a simple diagram to illustrate the concept:
| Radius (r) | Volume (V) |
| 4 cm | (4/3)π(4)^3 |
| 10 cm | (4/3)π(10)^3 |
Benefits of Visual Aids:
- Helps to visualize the concept.
- Makes it easier to understand the relationship between the radius and the volume.
- Helps to identify patterns and relationships.
5. Use Online Resources
There are numerous online resources available to help you master the volume of a sphere. Here are a few examples:
- Khan Academy: A free online platform that provides video tutorials and practice exercises on the volume of a sphere.
- Mathway: An online calculator that can help you calculate the volume of a sphere.
- Geometry Calculator: An online calculator that provides formulas and calculations for different geometric shapes, including the volume of a sphere.
Benefits of Online Resources:
- Provides interactive and engaging learning experiences.
- Offers practice exercises and quizzes to test your knowledge.
- Provides access to a wealth of information and resources.
Finally, mastering the volume of a sphere requires practice, patience, and persistence. With these tips and resources, you can become proficient in calculating the volume of a sphere and apply it to real-life problems.
In summary, mastering the volume of a sphere involves understanding the concept, practicing with different examples, applying it to real-life problems, using visual aids, and utilizing online resources. With these strategies, you can become proficient in calculating the volume of a sphere and apply it to a wide range of problems.
What is the formula for the volume of a sphere?
+The formula for the volume of a sphere is (4⁄3)πr^3, where r is the radius of the sphere.
How do I calculate the volume of a sphere with a diameter of 10 cm?
+First, find the radius of the sphere (diameter/2 = 10⁄2 = 5 cm). Then, plug the value of the radius into the formula (4⁄3)π(5)^3.
What are some real-life applications of the volume of a sphere?
+The volume of a sphere has numerous real-life applications, including calculating the volume of a basketball or a soccer ball, calculating the volume of a planet or a moon, and calculating the volume of a water tank or a container.