Solving Two Step Inequalities Made Easy
Understanding Two-Step Inequalities
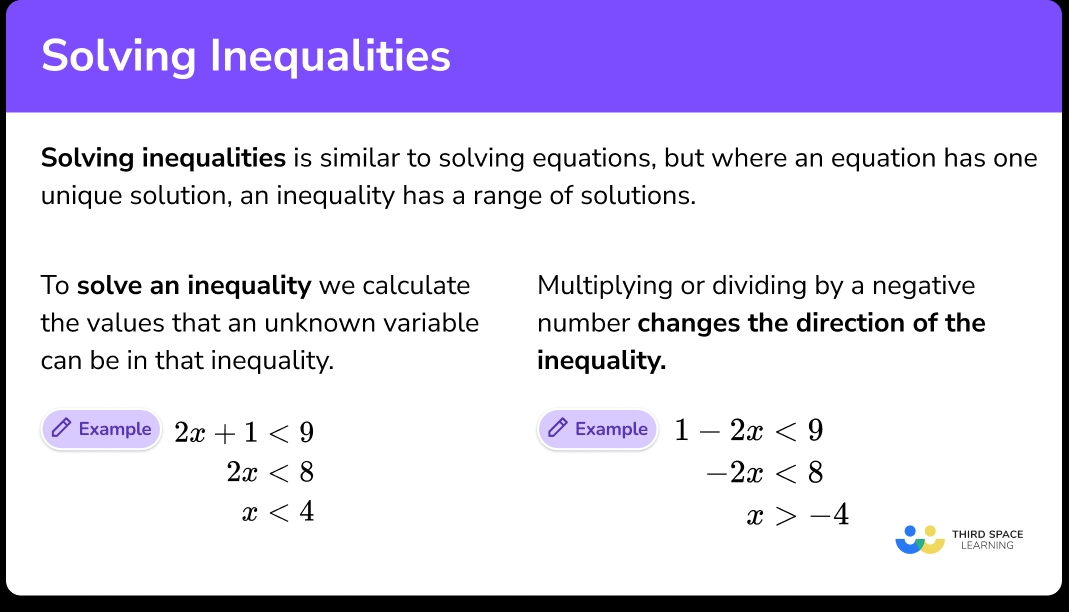
Inequalities can be a bit tricky to solve, especially when they involve multiple steps. Two-step inequalities are a type of inequality that requires two operations to solve. These inequalities can be additive, subtractive, multiplicative, or divisive. In this article, we will explore how to solve two-step inequalities in a step-by-step manner.
What are Two-Step Inequalities?
Two-step inequalities are inequalities that require two operations to isolate the variable. For example:
2x + 5 > 11
To solve this inequality, we need to perform two operations: subtracting 5 from both sides and then dividing both sides by 2.
Types of Two-Step Inequalities
There are four types of two-step inequalities:
- Additive inequalities: These inequalities involve addition or subtraction. For example: x + 3 > 7
- Subtractive inequalities: These inequalities involve subtraction. For example: x - 2 < 5
- Multiplicative inequalities: These inequalities involve multiplication or division. For example: 2x > 6
- Divisive inequalities: These inequalities involve division. For example: x/3 < 2
How to Solve Two-Step Inequalities
Solving two-step inequalities is a straightforward process. Here are the steps:
- Read the inequality carefully: Read the inequality carefully and identify the operations involved.
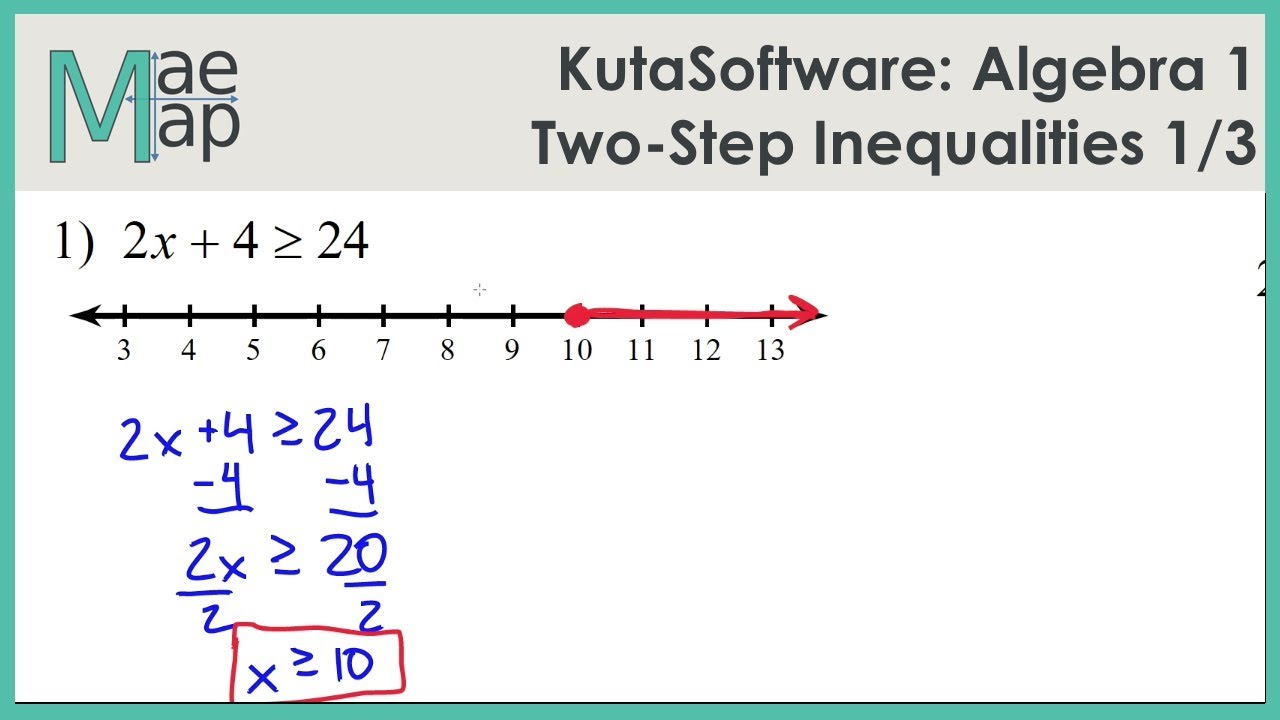
- Perform the first operation: Perform the first operation to isolate the variable. For example, if the inequality is 2x + 5 > 11, subtract 5 from both sides to get 2x > 6.
- Perform the second operation: Perform the second operation to isolate the variable. For example, divide both sides of the inequality 2x > 6 by 2 to get x > 3.
- Check the solution: Check the solution by plugging it back into the original inequality.
📝 Note: When solving two-step inequalities, it is essential to follow the order of operations (PEMDAS) to avoid errors.
Examples of Two-Step Inequalities
Here are some examples of two-step inequalities:
- x + 2 > 9
- 3x - 2 < 11
- 2x/3 > 4
- x - 1 < 6
How to Solve Two-Step Inequalities with Variables on Both Sides
Sometimes, two-step inequalities may have variables on both sides. To solve these inequalities, we need to follow these steps:
- Add or subtract the same value to both sides: Add or subtract the same value to both sides to get all the variables on one side.
- Perform the second operation: Perform the second operation to isolate the variable.
For example, consider the inequality x + 2 > 3x - 1. To solve this inequality, we need to add 1 to both sides to get x + 3 > 3x. Then, subtract x from both sides to get 3 > 2x. Finally, divide both sides by 2 to get x < 1.5.
Common Mistakes to Avoid
When solving two-step inequalities, there are some common mistakes to avoid:
- Reversing the inequality sign: When multiplying or dividing both sides by a negative number, the inequality sign must be reversed.
- Forgetting to follow the order of operations: It is essential to follow the order of operations (PEMDAS) to avoid errors.
- Not checking the solution: Always check the solution by plugging it back into the original inequality.
| Inequality | Solution |
|---|---|
| x + 2 > 9 | x > 7 |
| 3x - 2 < 11 | x < 4.33 |
| 2x/3 > 4 | x > 6 |
| x - 1 < 6 | x < 7 |
Conclusion
Solving two-step inequalities is a straightforward process that requires attention to detail and following the order of operations. By understanding the different types of two-step inequalities and following the steps outlined in this article, you can solve these inequalities with ease.
What is a two-step inequality?
+A two-step inequality is an inequality that requires two operations to solve.
How do I solve a two-step inequality?
+To solve a two-step inequality, perform the first operation to isolate the variable, and then perform the second operation to solve for the variable.
What are some common mistakes to avoid when solving two-step inequalities?
+Common mistakes to avoid include reversing the inequality sign, forgetting to follow the order of operations, and not checking the solution.