5 Ways to Master Multiplying Monomials by Polynomials
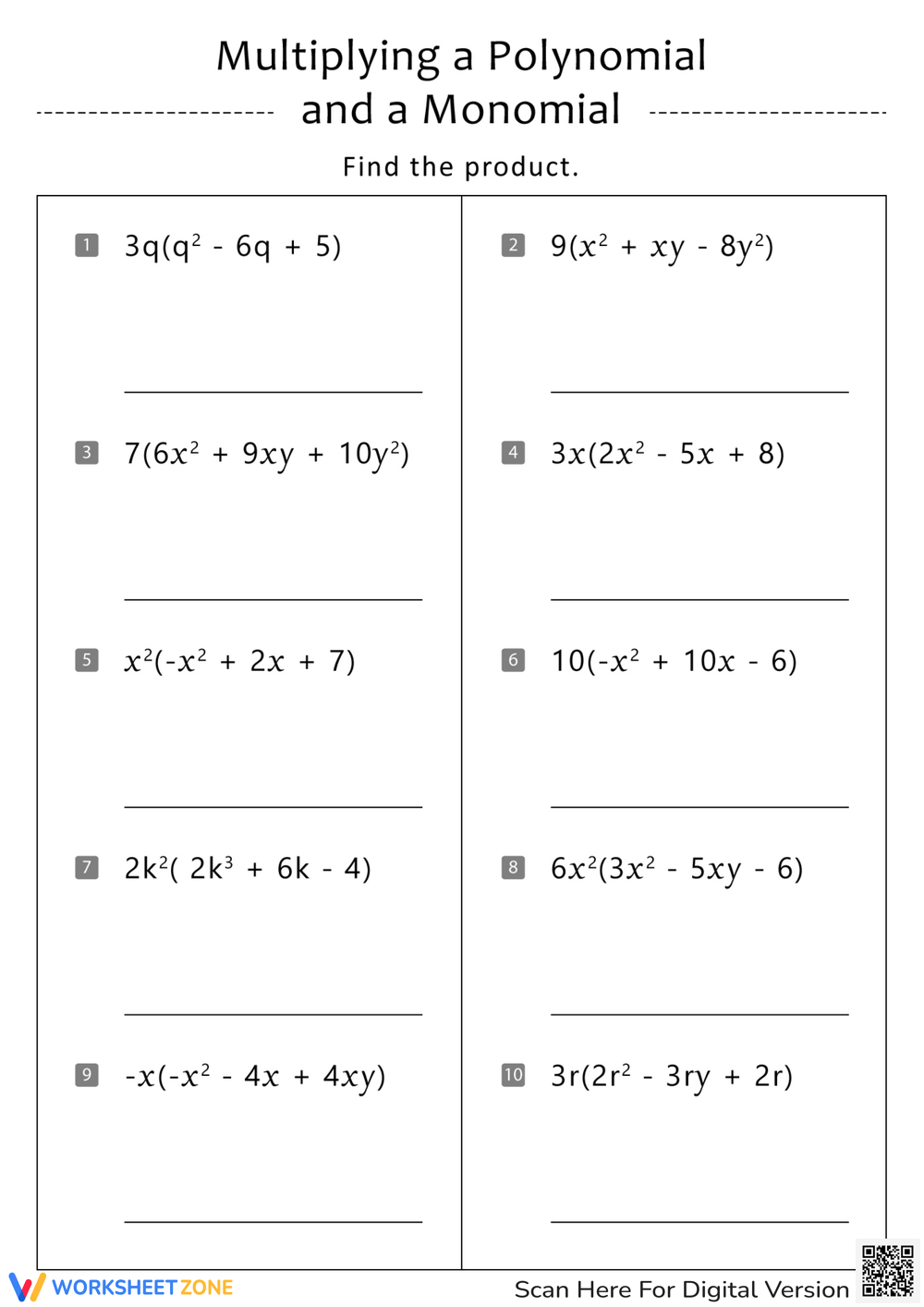
Understanding the Basics of Multiplying Monomials by Polynomials
Multiplying monomials by polynomials is a fundamental concept in algebra that can seem daunting at first, but with practice and patience, it can become second nature. A monomial is an expression consisting of a single term, such as 2x or 3y, while a polynomial is an expression consisting of multiple terms, such as x^2 + 3x - 4. When multiplying a monomial by a polynomial, the key is to apply the distributive property, which states that a single term can be multiplied by each term in the polynomial.
Method 1: Using the Distributive Property
The distributive property is the cornerstone of multiplying monomials by polynomials. It states that for any real numbers a, b, and c, a(b + c) = ab + ac. This property can be applied to polynomials as well. For example, when multiplying 2x by x^2 + 3x - 4, we can apply the distributive property as follows:
2x(x^2 + 3x - 4) = 2x(x^2) + 2x(3x) - 2x(4) = 2x^3 + 6x^2 - 8x
As you can see, the distributive property allows us to multiply each term in the polynomial by the monomial.
Method 2: Breaking Down the Polynomial
Another approach to multiplying monomials by polynomials is to break down the polynomial into smaller parts. For example, when multiplying 3y by 2x^2 + 5x - 3, we can break down the polynomial into three separate parts:
(3y)(2x^2) = 6yx^2 (3y)(5x) = 15yx (3y)(-3) = -9y
Then, we can combine the results to get the final answer:
6yx^2 + 15yx - 9y
Method 3: Using the FOIL Method
The FOIL method is a popular technique for multiplying two binomials, but it can also be applied to multiplying monomials by polynomials. FOIL stands for “First, Outer, Inner, Last,” which refers to the order in which we multiply the terms. For example, when multiplying 2x by x^2 + 3x - 4, we can use the FOIL method as follows:
(2x)(x^2) = 2x^3 (First) (2x)(3x) = 6x^2 (Outer) (2x)(-4) = -8x (Inner) (2x)(1) = 2x (Last)
Then, we can combine the results to get the final answer:
2x^3 + 6x^2 - 8x
Method 4: Visualizing the Multiplication
Visualizing the multiplication process can also be helpful when multiplying monomials by polynomials. We can use a diagram to represent the multiplication, with the monomial on one side and the polynomial on the other. For example, when multiplying 3y by 2x^2 + 5x - 3, we can create a diagram like this:

| 2x^2 | 5x | -3 | |
|---|---|---|---|
| 3y | 6yx^2 | 15yx | -9y |
As you can see, the diagram helps us visualize the multiplication process and ensures that we don’t miss any terms.
Method 5: Practicing with Different Types of Polynomials
Finally, the key to mastering multiplying monomials by polynomials is to practice with different types of polynomials. This includes polynomials with different degrees, such as quadratic and cubic polynomials, as well as polynomials with different coefficients, such as fractions and decimals. By practicing with different types of polynomials, we can develop a deeper understanding of the multiplication process and become more confident in our ability to multiply monomials by polynomials.
| Monomial | Polynomial | Product |
|---|---|---|
| 2x | x^2 + 3x - 4 | 2x^3 + 6x^2 - 8x |
| 3y | 2x^2 + 5x - 3 | 6yx^2 + 15yx - 9y |
| 4z | x^3 + 2x^2 - 5x + 1 | 4zx^3 + 8zx^2 - 20zx + 4z |
💡 Note: When multiplying monomials by polynomials, it's essential to apply the distributive property carefully and ensure that each term in the polynomial is multiplied by the monomial.
By using these five methods, we can become proficient in multiplying monomials by polynomials and develop a strong foundation in algebra.
What is the distributive property?
+The distributive property is a mathematical property that states that a single term can be multiplied by each term in a polynomial.
How do I multiply a monomial by a polynomial?
+To multiply a monomial by a polynomial, apply the distributive property by multiplying each term in the polynomial by the monomial.
What is the FOIL method?
+The FOIL method is a technique for multiplying two binomials, but it can also be applied to multiplying monomials by polynomials. FOIL stands for “First, Outer, Inner, Last.”